Question and Answers Forum

AlgebraQuestion and Answers: Page 266

Question Number 94603 Answers: 1 Comments: 1

List the elements in C={x:x is an x^2 ≤4, integer}

$$\mathrm{List}\:\mathrm{the}\:\mathrm{elements}\:\mathrm{in}\: \\ $$$${C}=\left\{{x}:{x}\:\mathrm{is}\:\mathrm{an}\:{x}^{\mathrm{2}} \leqslant\mathrm{4},\:\mathrm{integer}\right\} \\ $$

Question Number 94601 Answers: 1 Comments: 0

Question Number 94522 Answers: 3 Comments: 0

if sinA+sinB=n and cosA+cosB=m then sin(A+B)=? a: ((3mn)/(m^2 +n^2 )) b:((2mn)/(m^2 +n^2 )) c:((mn)/(m^2 +n^2 )) d:((2mn)/(m+n)) with steps?

$$\mathrm{if}\:\:\mathrm{sinA}+\mathrm{sinB}=\mathrm{n}\:\mathrm{and}\:\mathrm{cosA}+\mathrm{cosB}=\mathrm{m} \\ $$$$\mathrm{then}\:\:\mathrm{sin}\left(\mathrm{A}+\mathrm{B}\right)=? \\ $$$$\mathrm{a}:\:\frac{\mathrm{3mn}}{\mathrm{m}^{\mathrm{2}} +\mathrm{n}^{\mathrm{2}} }\:\:\:\:\mathrm{b}:\frac{\mathrm{2mn}}{\mathrm{m}^{\mathrm{2}} +\mathrm{n}^{\mathrm{2}} }\:\:\mathrm{c}:\frac{\mathrm{mn}}{\mathrm{m}^{\mathrm{2}} +\mathrm{n}^{\mathrm{2}} }\:\:\mathrm{d}:\frac{\mathrm{2mn}}{\mathrm{m}+\mathrm{n}} \\ $$$$\mathrm{with}\:\mathrm{steps}? \\ $$

Question Number 94521 Answers: 3 Comments: 3

if a^(10) +a^5 +1=0 then a^(2005) +(1/a^(2005) )=? a: a^(10) +a^(11) b:a^(10) +a^5 c:3(a^(10) +a^5 ) d:0 with steps?

$$\mathrm{if}\:\mathrm{a}^{\mathrm{10}} +\mathrm{a}^{\mathrm{5}} +\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{then}\:\mathrm{a}^{\mathrm{2005}} +\frac{\mathrm{1}}{\mathrm{a}^{\mathrm{2005}} }=? \\ $$$$\mathrm{a}:\:\mathrm{a}^{\mathrm{10}} +\mathrm{a}^{\mathrm{11}} \:\:\:\:\mathrm{b}:\mathrm{a}^{\mathrm{10}} +\mathrm{a}^{\mathrm{5}} \:\:\:\mathrm{c}:\mathrm{3}\left(\mathrm{a}^{\mathrm{10}} +\mathrm{a}^{\mathrm{5}} \right)\:\:\mathrm{d}:\mathrm{0} \\ $$$$\mathrm{with}\:\mathrm{steps}? \\ $$

Question Number 94479 Answers: 1 Comments: 0

find solution in C x^4 +x^3 +x^2 +x+1 = 0

$$\mathrm{find}\:\mathrm{solution}\:\mathrm{in}\:\mathbb{C}\: \\ $$$$\mathrm{x}^{\mathrm{4}} +\mathrm{x}^{\mathrm{3}} +\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}\:=\:\mathrm{0} \\ $$

Question Number 94463 Answers: 0 Comments: 2

lim_(x→∞) ((6x^(k−2) +3x^2 +10)/(3x^5 +x+20)) then k^2 +1=?

$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\frac{\mathrm{6x}^{\mathrm{k}−\mathrm{2}} +\mathrm{3x}^{\mathrm{2}} +\mathrm{10}}{\mathrm{3x}^{\mathrm{5}} +\mathrm{x}+\mathrm{20}} \\ $$$$\mathrm{then}\:\mathrm{k}^{\mathrm{2}} +\mathrm{1}=? \\ $$

Question Number 94456 Answers: 1 Comments: 0

log_x 16+log_(16) x=log_(512) x+log_x 512 x=?

$$\mathrm{log}_{\mathrm{x}} \mathrm{16}+\mathrm{log}_{\mathrm{16}} \mathrm{x}=\mathrm{log}_{\mathrm{512}} \mathrm{x}+\mathrm{log}_{\mathrm{x}} \mathrm{512} \\ $$$$\mathrm{x}=? \\ $$

Question Number 94424 Answers: 1 Comments: 4

y=x^x y^′ =?

$$\mathrm{y}=\mathrm{x}^{\mathrm{x}} \\ $$$$\mathrm{y}^{'} =? \\ $$

Question Number 94406 Answers: 0 Comments: 5

Question Number 94309 Answers: 0 Comments: 0

Question Number 94257 Answers: 2 Comments: 0

If 9y^2 + (1/y^2 ) =3, then find the value of 27y^3 + (1/y^3 )

$$\mathrm{If}\:\mathrm{9y}^{\mathrm{2}} +\:\frac{\mathrm{1}}{\mathrm{y}^{\mathrm{2}} }\:=\mathrm{3},\:\mathrm{then}\:\mathrm{find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of} \\ $$$$\mathrm{27y}^{\mathrm{3}} \:+\:\frac{\mathrm{1}}{\mathrm{y}^{\mathrm{3}} } \\ $$

Question Number 94174 Answers: 1 Comments: 3

{ ((x+y = 10)),(((x)^(1/(3 )) + (y)^(1/(3 )) = (5/2) ((xy))^(1/(6 )) )) :} find x &y??

$$\begin{cases}{\mathrm{x}+\mathrm{y}\:=\:\mathrm{10}}\\{\sqrt[{\mathrm{3}\:\:}]{\mathrm{x}}\:+\:\sqrt[{\mathrm{3}\:\:}]{\mathrm{y}}\:=\:\frac{\mathrm{5}}{\mathrm{2}}\:\sqrt[{\mathrm{6}\:\:}]{\mathrm{xy}}}\end{cases}\: \\ $$$$\mathrm{find}\:\mathrm{x}\:\&\mathrm{y}?? \\ $$

Question Number 94124 Answers: 0 Comments: 3

20+a=a cosh(((75)/a)) a=?

$$\mathrm{20}+{a}={a}\:{cosh}\left(\frac{\mathrm{75}}{{a}}\right) \\ $$$${a}=? \\ $$

Question Number 94095 Answers: 0 Comments: 0

How many subgroups do Z_3 ⊕Z_(16 ) has? Justify.

$$\mathrm{How}\:\mathrm{many}\:\mathrm{subgroups}\:\mathrm{do}\:\mathrm{Z}_{\mathrm{3}} \oplus\mathrm{Z}_{\mathrm{16}\:} \:\mathrm{has}?\:\mathrm{Justify}. \\ $$

Question Number 93963 Answers: 0 Comments: 12

we have for quadratic equations x=((−b±(√(b^2 −4ac)))/(2a)) what about cubic equation is there any rules or ways to solve?

$${we}\:{have}\:{for}\:{quadratic}\:{equations} \\ $$$${x}=\frac{−{b}\pm\sqrt{{b}^{\mathrm{2}} −\mathrm{4}{ac}}}{\mathrm{2}{a}} \\ $$$${what}\:{about}\:{cubic}\:{equation}\:{is}\:{there}\:{any} \\ $$$${rules}\:{or}\:{ways}\:{to}\:{solve}? \\ $$

Question Number 93916 Answers: 0 Comments: 2

Question Number 93742 Answers: 0 Comments: 5

No my post option again?? at Tinkutara. because i cannot find my post options again

$$\mathrm{No}\:\mathrm{my}\:\mathrm{post}\:\mathrm{option}\:\mathrm{again}?? \\ $$$$\mathrm{at}\:\mathrm{Tinkutara}. \\ $$$$\mathrm{because}\:\mathrm{i}\:\mathrm{cannot}\:\mathrm{find}\:\mathrm{my}\:\mathrm{post}\:\mathrm{options}\:\mathrm{again} \\ $$

Question Number 93726 Answers: 0 Comments: 1

find the value in sexagesimal numeral system 3° × 15°=? 3 × 15°=?

$${find}\:{the}\:{value}\:{in}\:{sexagesimal}\:{numeral} \\ $$$${system} \\ $$$$\mathrm{3}°\:×\:\mathrm{15}°=? \\ $$$$\mathrm{3}\:×\:\mathrm{15}°=? \\ $$

Question Number 93505 Answers: 0 Comments: 0

Does (x,y)+(x^′ ,y^′ )=(x+x^′ , y+y^′ ) form a vector space? λ(x,y)=(λx,λy)

$$\mathrm{Does} \\ $$$$\left(\mathrm{x},\mathrm{y}\right)+\left(\mathrm{x}^{'} ,\mathrm{y}^{'} \right)=\left(\mathrm{x}+\mathrm{x}^{'} ,\:\mathrm{y}+\mathrm{y}^{'} \right) \\ $$$$\mathrm{form}\:\mathrm{a}\:\mathrm{vector}\:\mathrm{space}? \\ $$$$\lambda\left(\mathrm{x},\mathrm{y}\right)=\left(\lambda\mathrm{x},\lambda\mathrm{y}\right) \\ $$

Question Number 93470 Answers: 1 Comments: 4

Solve: 3x^(x + 1) − 3x^(x − 1) = 8

$$\boldsymbol{\mathrm{Solve}}:\:\:\:\:\mathrm{3}\boldsymbol{\mathrm{x}}^{\boldsymbol{\mathrm{x}}\:\:+\:\:\mathrm{1}} \:\:−\:\:\mathrm{3}\boldsymbol{\mathrm{x}}^{\boldsymbol{\mathrm{x}}\:\:−\:\:\mathrm{1}} \:\:\:=\:\:\:\:\mathrm{8} \\ $$

Question Number 93460 Answers: 1 Comments: 1

solve for x,y >0 2x⌊y⌋ = 2020 3y⌊x⌋ = 2021

$$\mathrm{solve}\:\mathrm{for}\:\mathrm{x},\mathrm{y}\:>\mathrm{0} \\ $$$$\mathrm{2}{x}\lfloor\mathrm{y}\rfloor\:=\:\mathrm{2020} \\ $$$$\mathrm{3y}\lfloor{x}\rfloor\:=\:\mathrm{2021}\: \\ $$

Question Number 93446 Answers: 0 Comments: 1

what is the coefficient of x^5 in the expansion (1+x^2 )(1+x)^4

$$\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{coefficient}\:\mathrm{of}\:\mathrm{x}^{\mathrm{5}} \\ $$$$\mathrm{in}\:\mathrm{the}\:\mathrm{expansion}\:\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{x}\right)^{\mathrm{4}} \\ $$

Question Number 93439 Answers: 2 Comments: 0

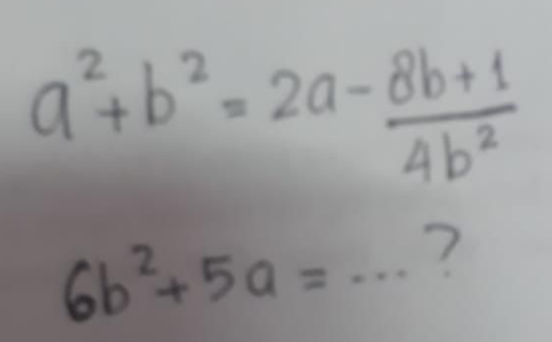
Question Number 93340 Answers: 1 Comments: 7

Σ_(k = 1) ^(2011) (1/(k(k+1)(k+2))) ?

$$\underset{\mathrm{k}\:=\:\mathrm{1}} {\overset{\mathrm{2011}} {\sum}}\:\frac{\mathrm{1}}{\mathrm{k}\left(\mathrm{k}+\mathrm{1}\right)\left(\mathrm{k}+\mathrm{2}\right)}\:? \\ $$

Question Number 93296 Answers: 1 Comments: 1

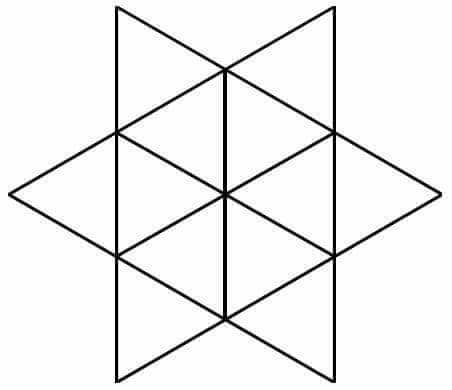
Question Number 93177 Answers: 0 Comments: 3

x^2 +(1/x^2 )=47 (√x)+(1/(√x))=...

$${x}^{\mathrm{2}} +\frac{\mathrm{1}}{{x}^{\mathrm{2}} }=\mathrm{47} \\ $$$$\sqrt{{x}}+\frac{\mathrm{1}}{\sqrt{{x}}}=... \\ $$

Pg 261 Pg 262 Pg 263 Pg 264 Pg 265 Pg 266 Pg 267 Pg 268 Pg 269 Pg 270

Contact: info@tinkutara.com