Question and Answers Forum

AlgebraQuestion and Answers: Page 264

Question Number 96072 Answers: 1 Comments: 0

(x^2 +24x+24).(x^2 +x+24)= 24x^2

$$\left(\mathrm{x}^{\mathrm{2}} +\mathrm{24x}+\mathrm{24}\right).\left(\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{24}\right)=\:\mathrm{24x}^{\mathrm{2}} \\ $$

Question Number 96021 Answers: 0 Comments: 1

if p and q are two complex number and p×q=m ,m is a real number . is there always exists a p^(1/3) and q^(1/3) (we know p^(1/3) and q^(1/3) each has actually 3 values) such that p^(1/3) ×q^(1/3) =m^(1/3) .where m^(1/3) is real .?? how to prove it?

Question Number 95964 Answers: 0 Comments: 0

{ ((x^2 +y^2 =13)),((2x^2 +3y=2xy^2 )) :}

$$\begin{cases}{\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} =\mathrm{13}}\\{\mathrm{2x}^{\mathrm{2}} +\mathrm{3y}=\mathrm{2xy}^{\mathrm{2}} }\end{cases} \\ $$

Question Number 95967 Answers: 1 Comments: 1

Question Number 95920 Answers: 3 Comments: 0

((54+(√x)))^(1/(3 )) + ((54−(√x)))^(1/(3 )) = ((18))^(1/(3 )) x = ?

$$\sqrt[{\mathrm{3}\:\:}]{\mathrm{54}+\sqrt{\mathrm{x}}}\:+\:\sqrt[{\mathrm{3}\:\:}]{\mathrm{54}−\sqrt{\mathrm{x}}}\:=\:\sqrt[{\mathrm{3}\:\:}]{\mathrm{18}}\: \\ $$$$\mathrm{x}\:=\:?\: \\ $$

Question Number 95919 Answers: 1 Comments: 0

∫3^(−4x^2 ) dx=? (0,∞)

$$ \\ $$$$\int\mathrm{3}^{−\mathrm{4x}^{\mathrm{2}} } \mathrm{dx}=?\:\:\:\:\left(\mathrm{0},\infty\right) \\ $$

Question Number 95898 Answers: 0 Comments: 0

x^2 +xy+(y^3 /3)=25 (y^2 /3)+z^2 =9 z^2 +zx+x^2 =16 so xy+2yz+3zx=?

$${x}^{\mathrm{2}} +{xy}+\frac{{y}^{\mathrm{3}} }{\mathrm{3}}=\mathrm{25} \\ $$$$\frac{{y}^{\mathrm{2}} }{\mathrm{3}}+{z}^{\mathrm{2}} =\mathrm{9} \\ $$$${z}^{\mathrm{2}} +{zx}+{x}^{\mathrm{2}} =\mathrm{16} \\ $$$${so}\:{xy}+\mathrm{2}{yz}+\mathrm{3}{zx}=? \\ $$

Question Number 95843 Answers: 1 Comments: 0

((((√(3x−7)))^2 −2)/(x−3)) ≤ ((3−((√x))^2 )/(x−3)) find the solution

$$\frac{\left(\sqrt{\mathrm{3x}−\mathrm{7}}\right)^{\mathrm{2}} −\mathrm{2}}{\mathrm{x}−\mathrm{3}}\:\leqslant\:\frac{\mathrm{3}−\left(\sqrt{\mathrm{x}}\right)^{\mathrm{2}} }{\mathrm{x}−\mathrm{3}}\: \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{solution}\: \\ $$

Question Number 95789 Answers: 1 Comments: 1

Find the semi−interquartile range of of the following numbers: 15, 10, 9, 15, 15, 8, 10, 11, 8, 12, 11, 14, 9 and 15

$$\:\mathrm{Find}\:\mathrm{the}\:\mathrm{semi}−\mathrm{interquartile}\:\mathrm{range}\:\mathrm{of}\: \\ $$$$\:\mathrm{of}\:\mathrm{the}\:\mathrm{following}\:\mathrm{numbers}: \\ $$$$\:\mathrm{15},\:\mathrm{10},\:\mathrm{9},\:\mathrm{15},\:\mathrm{15},\:\mathrm{8},\:\mathrm{10},\:\mathrm{11},\:\mathrm{8},\:\mathrm{12},\:\mathrm{11},\:\mathrm{14}, \\ $$$$\:\mathrm{9}\:\mathrm{and}\:\mathrm{15} \\ $$

Question Number 95767 Answers: 1 Comments: 0

Question Number 95766 Answers: 0 Comments: 1

Solve the equation 2^(2x) − 5x^2 + 4 = 0

$$\mathrm{Solve}\:\mathrm{the}\:\mathrm{equation} \\ $$$$\:\:\:\:\:\:\:\mathrm{2}^{\mathrm{2x}} \:\:−\:\:\mathrm{5x}^{\mathrm{2}} \:\:+\:\:\mathrm{4}\:\:\:=\:\:\:\mathrm{0} \\ $$

Question Number 95765 Answers: 0 Comments: 3

Sum the series: 2((1/(40)) + (1/(20)) + (1/(10)) + ... + n)

$$\mathrm{Sum}\:\mathrm{the}\:\mathrm{series}: \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{2}\left(\frac{\mathrm{1}}{\mathrm{40}}\:\:+\:\:\frac{\mathrm{1}}{\mathrm{20}}\:\:+\:\:\frac{\mathrm{1}}{\mathrm{10}}\:\:+\:\:...\:\:+\:\:\boldsymbol{\mathrm{n}}\right) \\ $$

Question Number 95742 Answers: 2 Comments: 2

(0/0)=2 ((100−100)/(100−100))=((10^2 −10^2 )/(10^2 −10^2 ))=(((10+10)(10−10))/(10(10−10))) ((20)/(10))=2 where is the mastike

$$\frac{\mathrm{0}}{\mathrm{0}}=\mathrm{2} \\ $$$$\frac{\mathrm{100}−\mathrm{100}}{\mathrm{100}−\mathrm{100}}=\frac{\mathrm{10}^{\mathrm{2}} −\mathrm{10}^{\mathrm{2}} }{\mathrm{10}^{\mathrm{2}} −\mathrm{10}^{\mathrm{2}} }=\frac{\left(\mathrm{10}+\mathrm{10}\right)\left(\mathrm{10}−\mathrm{10}\right)}{\mathrm{10}\left(\mathrm{10}−\mathrm{10}\right)} \\ $$$$\frac{\mathrm{20}}{\mathrm{10}}=\mathrm{2} \\ $$$$\mathrm{where}\:\mathrm{is}\:\mathrm{the}\:\mathrm{mastike} \\ $$

Question Number 95668 Answers: 1 Comments: 2

Prove that: 2^(1/4) .4^(1/8) .8^(1/16) .16^(1/32) . ... ∞ = 2

$$\mathrm{Prove}\:\mathrm{that}:\:\:\:\mathrm{2}^{\mathrm{1}/\mathrm{4}} .\mathrm{4}^{\mathrm{1}/\mathrm{8}} .\mathrm{8}^{\mathrm{1}/\mathrm{16}} .\mathrm{16}^{\mathrm{1}/\mathrm{32}} .\:\:...\:\:\infty\:\:\:=\:\:\:\mathrm{2} \\ $$

Question Number 95648 Answers: 0 Comments: 8

if 6^x =18 and 12^y =3 then x=? ((3y−4)/(y+4)) ((3y+2)/(y+2)) ((3y−3)/(3y−3)) ((3y+1)/(y+1))

$$\mathrm{if}\:\mathrm{6}^{\mathrm{x}} =\mathrm{18}\:\mathrm{and}\:\:\mathrm{12}^{\mathrm{y}} =\mathrm{3} \\ $$$$\mathrm{then}\:\:\mathrm{x}=? \\ $$$$\frac{\mathrm{3y}−\mathrm{4}}{\mathrm{y}+\mathrm{4}}\:\:\:\:\:\:\:\:\frac{\mathrm{3y}+\mathrm{2}}{\mathrm{y}+\mathrm{2}}\:\:\:\:\:\:\:\:\frac{\mathrm{3y}−\mathrm{3}}{\mathrm{3y}−\mathrm{3}}\:\:\:\:\:\:\:\:\:\:\frac{\mathrm{3y}+\mathrm{1}}{\mathrm{y}+\mathrm{1}} \\ $$

Question Number 95608 Answers: 3 Comments: 2

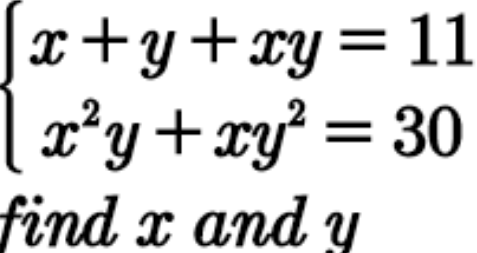
Question Number 95560 Answers: 1 Comments: 1

Question Number 95502 Answers: 1 Comments: 3

6 man + 8 woman ⇒working a job in 10 days 26 man + 48 woman ⇒ in 2 days if 15 man + 20 woman ⇒ ?? days

$$\mathrm{6}\:\mathrm{man}\:+\:\mathrm{8}\:\mathrm{woman}\:\Rightarrow\mathrm{working}\:\mathrm{a}\:\mathrm{job}\:\mathrm{in}\:\mathrm{10}\:\mathrm{days} \\ $$$$\mathrm{26}\:\mathrm{man}\:+\:\mathrm{48}\:\mathrm{woman}\:\Rightarrow\:\mathrm{in}\:\mathrm{2}\:\mathrm{days} \\ $$$$\mathrm{if}\:\mathrm{15}\:\mathrm{man}\:+\:\mathrm{20}\:\mathrm{woman}\:\Rightarrow\:??\:\mathrm{days} \\ $$

Question Number 95469 Answers: 0 Comments: 1

(9b^2 −25) why is this inside the bracket as it is a diffetence of two squares?

$$\left(\mathrm{9b}^{\mathrm{2}} −\mathrm{25}\right) \\ $$$$\mathrm{why}\:\mathrm{is}\:\mathrm{this}\:\mathrm{inside}\:\mathrm{the}\:\mathrm{bracket}\:\mathrm{as}\:\mathrm{it}\:\mathrm{is}\:\mathrm{a}\:\mathrm{diffetence}\:\mathrm{of}\:\mathrm{two}\:\mathrm{squares}? \\ $$

Question Number 95447 Answers: 1 Comments: 0

can I write the solution of ay′′+by′+cy=0 y= { ((c_1 e^(((−b+(√(b^2 −4ac)))/2)x) +c_2 e^(((−b−(√(b^2 −4ac)))/2)x) ,when b^2 −4ac≠0)),((c_1 e^(((−b)/2)x) +c_2 xe^(((−b)/2)x) ,when b^2 −4ac=0)) :} in one sentence not in the form of piecewide-define function

$${can}\:{I}\:{write}\:{the}\:{solution}\:{of} \\ $$$${ay}''+{by}'+{cy}=\mathrm{0} \\ $$$${y}=\begin{cases}{{c}_{\mathrm{1}} {e}^{\frac{−{b}+\sqrt{{b}^{\mathrm{2}} −\mathrm{4}{ac}}}{\mathrm{2}}{x}} +{c}_{\mathrm{2}} {e}^{\frac{−{b}−\sqrt{{b}^{\mathrm{2}} −\mathrm{4}{ac}}}{\mathrm{2}}{x}} ,{when}\:{b}^{\mathrm{2}} −\mathrm{4}{ac}\neq\mathrm{0}}\\{{c}_{\mathrm{1}} {e}^{\frac{−{b}}{\mathrm{2}}{x}} +{c}_{\mathrm{2}} {xe}^{\frac{−{b}}{\mathrm{2}}{x}} ,{when}\:{b}^{\mathrm{2}} −\mathrm{4}{ac}=\mathrm{0}}\end{cases} \\ $$$${in}\:{one}\:{sentence} \\ $$$${not}\:{in}\:{the}\:{form}\:{of}\:{piecewide}-{define}\:{function} \\ $$

Question Number 95440 Answers: 1 Comments: 0

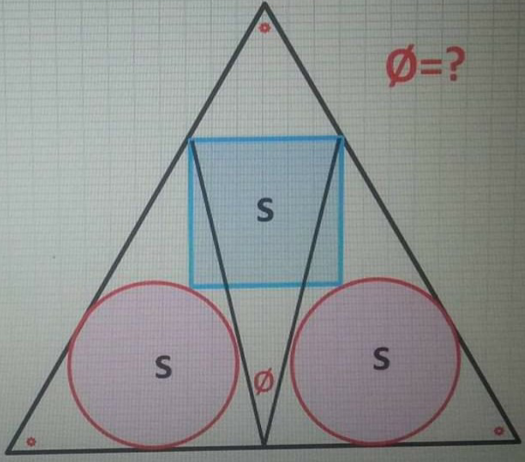
Question Number 95416 Answers: 1 Comments: 4

It takes 12 hours to fill a swimming pool using 2 pipes. If the larger pipe used , for 4 hours and the small pipe for 9 hours, only half the pool is filled. How long would it take for each pipe alone to fill the pool?

$$\mathrm{It}\:\mathrm{takes}\:\mathrm{12}\:\mathrm{hours}\:\mathrm{to}\:\mathrm{fill}\:\mathrm{a}\:\mathrm{swimming}\: \\ $$$$\mathrm{pool}\:\mathrm{using}\:\mathrm{2}\:\mathrm{pipes}.\:\mathrm{If}\:\mathrm{the}\:\mathrm{larger}\: \\ $$$$\mathrm{pipe}\:\mathrm{used}\:,\:\mathrm{for}\:\mathrm{4}\:\mathrm{hours}\:\mathrm{and}\:\mathrm{the}\: \\ $$$$\mathrm{small}\:\mathrm{pipe}\:\mathrm{for}\:\mathrm{9}\:\mathrm{hours},\:\mathrm{only}\:\mathrm{half} \\ $$$$\mathrm{the}\:\mathrm{pool}\:\mathrm{is}\:\mathrm{filled}.\:\mathrm{How}\:\mathrm{long}\:\mathrm{would}\: \\ $$$$\mathrm{it}\:\mathrm{take}\:\mathrm{for}\:\mathrm{each}\:\mathrm{pipe}\:\mathrm{alone}\:\mathrm{to}\: \\ $$$$\mathrm{fill}\:\mathrm{the}\:\mathrm{pool}? \\ $$

Question Number 95394 Answers: 0 Comments: 31

Solve: x + y = 3 .... (i) x^y + y^x = 6 ..... (ii)

$$\mathrm{Solve}:\:\:\:\mathrm{x}\:\:+\:\:\mathrm{y}\:\:=\:\:\mathrm{3}\:\:\:\:\:\:....\:\left(\mathrm{i}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{x}^{\mathrm{y}} \:\:+\:\:\mathrm{y}^{\mathrm{x}} \:\:=\:\:\mathrm{6}\:\:\:\:.....\:\:\left(\mathrm{ii}\right) \\ $$

Question Number 95373 Answers: 1 Comments: 2

Find the value of m for which the roots of the equation x^3 + 6x^2 + 11x +m = 0 form a linear sequence.

$$\: \\ $$$$\:\mathrm{Find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:{m}\:\mathrm{for}\:\mathrm{which}\:\mathrm{the}\:\mathrm{roots} \\ $$$$\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation}\:{x}^{\mathrm{3}} \:+\:\mathrm{6}{x}^{\mathrm{2}} \:+\:\mathrm{11}{x}\:+{m}\:=\:\mathrm{0} \\ $$$$\:\mathrm{form}\:\mathrm{a}\:\mathrm{linear}\:\mathrm{sequence}. \\ $$$$ \\ $$

Question Number 95328 Answers: 0 Comments: 3

Question Number 95324 Answers: 0 Comments: 2

If sin A + (sin A)^2 = 1 Then the value of (cos A)^(12) + 3(cos A)^(10) + 3(cos A)^8 + (cos A)^6 − 1 is ? (a) 0 (b) 1 (c) − 1 (d) 2

$$\mathrm{If}\:\:\:\mathrm{sin}\:\mathrm{A}\:\:+\:\:\left(\mathrm{sin}\:\mathrm{A}\right)^{\mathrm{2}} \:\:\:=\:\:\:\mathrm{1} \\ $$$$\mathrm{Then}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of} \\ $$$$\:\:\:\:\:\:\:\:\left(\mathrm{cos}\:\mathrm{A}\right)^{\mathrm{12}} \:\:+\:\:\mathrm{3}\left(\mathrm{cos}\:\mathrm{A}\right)^{\mathrm{10}} \:\:+\:\:\mathrm{3}\left(\mathrm{cos}\:\mathrm{A}\right)^{\mathrm{8}} \:\:+\:\:\left(\mathrm{cos}\:\mathrm{A}\right)^{\mathrm{6}} \:\:−\:\:\mathrm{1}\:\:\:\:\:\mathrm{is}\:? \\ $$$$ \\ $$$$\left(\mathrm{a}\right)\:\:\:\:\:\:\:\mathrm{0} \\ $$$$\left(\mathrm{b}\right)\:\:\:\:\:\:\:\:\mathrm{1} \\ $$$$\left(\mathrm{c}\right)\:\:\:\:−\:\mathrm{1} \\ $$$$\left(\mathrm{d}\right)\:\:\:\:\:\:\mathrm{2} \\ $$

Pg 259 Pg 260 Pg 261 Pg 262 Pg 263 Pg 264 Pg 265 Pg 266 Pg 267 Pg 268

Contact: info@tinkutara.com