Question and Answers Forum

All Questions Topic List

AlgebraQuestion and Answers: Page 259

Question Number 100660 Answers: 0 Comments: 1

∣x^2 −x∣ < 2+x . find solution set.

$$\mid{x}^{\mathrm{2}} −{x}\mid\:<\:\mathrm{2}+{x}\:.\:{find}\:{solution}\:{set}. \\ $$

Question Number 100622 Answers: 0 Comments: 2

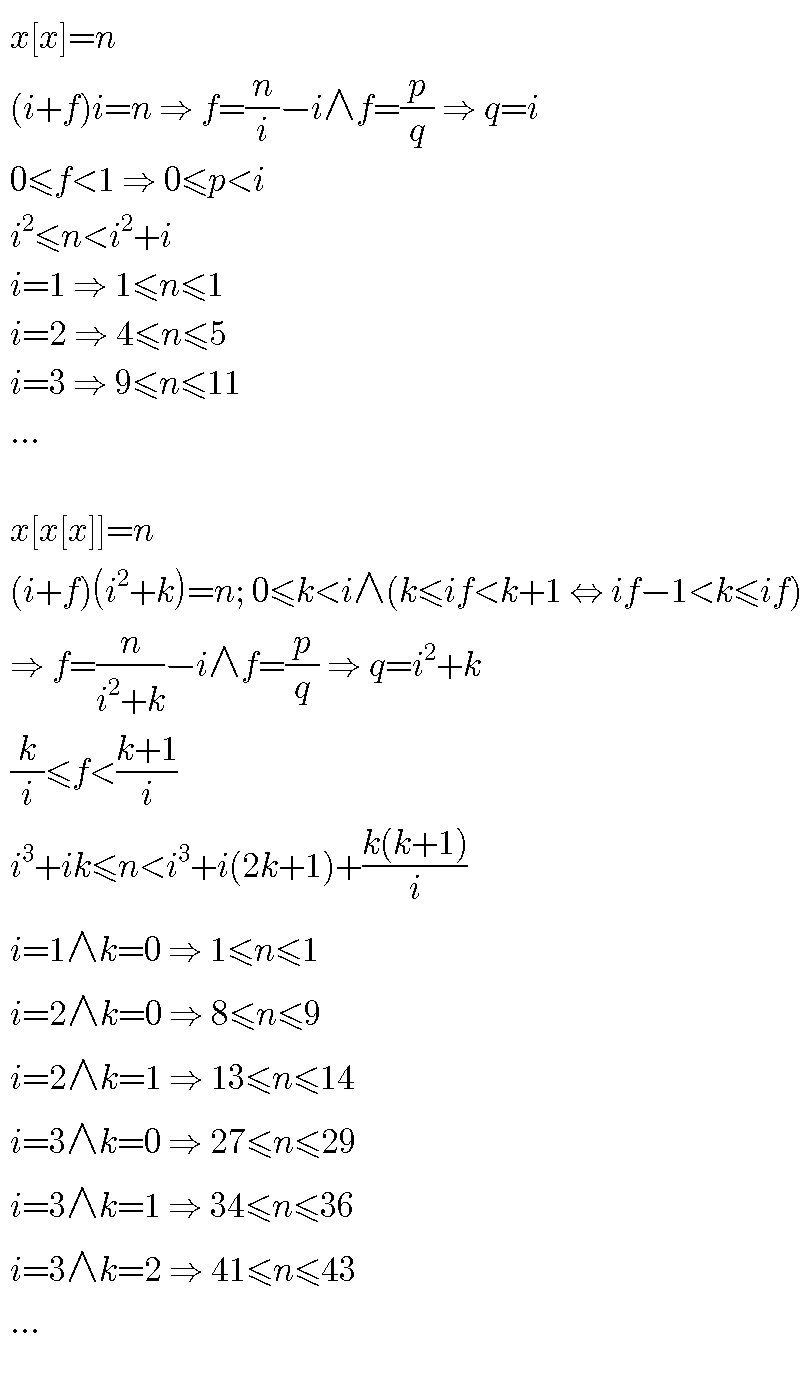
Question Number 100597 Answers: 2 Comments: 1

Question Number 100587 Answers: 2 Comments: 1

If the coefficients of x^k and x^(k+1) in the expansion (2+3x)^(19) are equal , what is the value of k ?

$$\mathrm{If}\:\mathrm{the}\:\mathrm{coefficients}\:\mathrm{of}\:{x}^{{k}} \:\mathrm{and}\:{x}^{{k}+\mathrm{1}} \:\mathrm{in}\:\mathrm{the}\: \\ $$$$\mathrm{expansion}\:\left(\mathrm{2}+\mathrm{3}{x}\right)^{\mathrm{19}} \:\mathrm{are}\:\mathrm{equal}\:,\:\mathrm{what}\:\mathrm{is} \\ $$$$\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:{k}\:? \\ $$

Question Number 100567 Answers: 0 Comments: 3

{ ((x−(√(yz)) = 42)),((y−(√(xz)) = 6)),((z−(√(xy)) = −30)) :} find x+y+z =

$$\begin{cases}{{x}−\sqrt{{yz}}\:=\:\mathrm{42}}\\{{y}−\sqrt{{xz}}\:=\:\mathrm{6}}\\{{z}−\sqrt{{xy}}\:=\:−\mathrm{30}}\end{cases} \\ $$$${find}\:{x}+{y}+{z}\:= \\ $$

Question Number 100562 Answers: 0 Comments: 0

Question Number 100540 Answers: 0 Comments: 1

Question Number 100492 Answers: 2 Comments: 3

((16−((64)/(16−((64)/(16−((64)/(16−...))))))))^(1/(3 )) −((−2−(1/(−2−(1/(−2−(1/(−2−...))))))))^(1/(3 ))

$$\sqrt[{\mathrm{3}\:\:\:}]{\mathrm{16}−\frac{\mathrm{64}}{\mathrm{16}−\frac{\mathrm{64}}{\mathrm{16}−\frac{\mathrm{64}}{\mathrm{16}−...}}}}−\sqrt[{\mathrm{3}\:\:}]{−\mathrm{2}−\frac{\mathrm{1}}{−\mathrm{2}−\frac{\mathrm{1}}{−\mathrm{2}−\frac{\mathrm{1}}{−\mathrm{2}−...}}}} \\ $$

Question Number 100404 Answers: 0 Comments: 11

Question Number 100385 Answers: 1 Comments: 2

find the solution set of inequality (((x^2 −9)(√(x+2)))/(x+(√((x+2)^2 )))) ≤ 0

$$\mathrm{find}\:\mathrm{the}\:\mathrm{solution}\:\mathrm{set}\:\mathrm{of}\:\mathrm{inequality} \\ $$$$\frac{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{9}\right)\sqrt{\mathrm{x}+\mathrm{2}}}{\mathrm{x}+\sqrt{\left(\mathrm{x}+\mathrm{2}\right)^{\mathrm{2}} }}\:\leqslant\:\mathrm{0} \\ $$

Question Number 100330 Answers: 0 Comments: 1

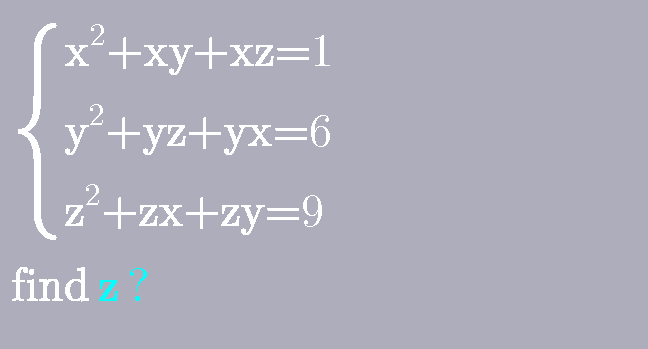
Question Number 100370 Answers: 1 Comments: 2

Find the maximum value of f(x) = (3/(2cosh (ln x) + 3))

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{maximum}\:\mathrm{value}\:\mathrm{of}\:\:{f}\left({x}\right)\:=\:\frac{\mathrm{3}}{\mathrm{2cosh}\:\left(\mathrm{ln}\:{x}\right)\:+\:\mathrm{3}} \\ $$

Question Number 100302 Answers: 0 Comments: 2

(√(3^(−(1/2)) +1)) = ((√(a+1))/3^(−(1/4)) ) . find a ?

$$\sqrt{\mathrm{3}^{−\frac{\mathrm{1}}{\mathrm{2}}} +\mathrm{1}}\:=\:\frac{\sqrt{\mathrm{a}+\mathrm{1}}}{\mathrm{3}^{−\frac{\mathrm{1}}{\mathrm{4}}} }\:.\:\mathrm{find}\:\mathrm{a}\:? \\ $$

Question Number 100198 Answers: 0 Comments: 1

Question Number 100193 Answers: 1 Comments: 3

(√(7+2(√(7−2(√(7+2(√(7−2(√(7+...)))))))))) ?

$$\sqrt{\mathrm{7}+\mathrm{2}\sqrt{\mathrm{7}−\mathrm{2}\sqrt{\mathrm{7}+\mathrm{2}\sqrt{\mathrm{7}−\mathrm{2}\sqrt{\mathrm{7}+...}}}}}\:? \\ $$

Question Number 100341 Answers: 1 Comments: 1

An open box with a square base is to be made out of a given quantity of a cardboard of area c^2 square units.show the maximum volume of the box (c^2 /(6(√3))) cubic units

$$\mathrm{An}\:\mathrm{open}\:\mathrm{box}\:\mathrm{with}\:\mathrm{a}\:\mathrm{square} \\ $$$$\mathrm{base}\:\mathrm{is}\:\mathrm{to}\:\mathrm{be}\:\mathrm{made}\:\mathrm{out} \\ $$$$\mathrm{of}\:\mathrm{a}\:\mathrm{given}\:\mathrm{quantity}\:\mathrm{of} \\ $$$$\mathrm{a}\:\mathrm{cardboard}\:\mathrm{of}\:\mathrm{area}\:\mathrm{c}^{\mathrm{2}} \\ $$$$\mathrm{square}\:\mathrm{units}.\mathrm{show}\:\mathrm{the} \\ $$$$\mathrm{maximum}\:\mathrm{volume}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{box}\:\frac{\mathrm{c}^{\mathrm{2}} }{\mathrm{6}\sqrt{\mathrm{3}}}\:\:\mathrm{cubic}\:\mathrm{units} \\ $$$$ \\ $$

Question Number 100040 Answers: 0 Comments: 2

Question Number 100002 Answers: 0 Comments: 0

Question Number 99916 Answers: 1 Comments: 0

can anyone recommend a good textbook from which i can learn calculus..^

$$\boldsymbol{\mathrm{can}}\:\boldsymbol{\mathrm{anyone}}\:\boldsymbol{\mathrm{recommend}}\:\boldsymbol{\mathrm{a}}\:\boldsymbol{\mathrm{good}}\:\boldsymbol{\mathrm{textbook}} \\ $$$$\boldsymbol{\mathrm{from}}\:\boldsymbol{\mathrm{which}}\:\boldsymbol{\mathrm{i}}\:\boldsymbol{\mathrm{can}}\:\boldsymbol{\mathrm{learn}}\:\boldsymbol{\mathrm{calculus}}.\hat {.} \\ $$

Question Number 99900 Answers: 0 Comments: 0

An insulated wire of diameter 1.22 mm carries a steady current of 5.4 A. The insulation material is 1.22 mm thick and has a? coeffiecient of thermal conductivity of 0.23 W/Km. the electrical resistivity of the material of the wire is 5.2 ×10^(−7) Ωm. find the temperature difference between the inner and outer surface of the insulated material when steady state is reached.

$$\mathrm{An}\:\mathrm{insulated}\:\mathrm{wire}\:\mathrm{of}\:\mathrm{diameter}\:\mathrm{1}.\mathrm{22}\:\mathrm{mm}\:\mathrm{carries}\:\mathrm{a}\:\mathrm{steady}\:\mathrm{current} \\ $$$$\mathrm{of}\:\mathrm{5}.\mathrm{4}\:\mathrm{A}.\:\mathrm{The}\:\mathrm{insulation}\:\mathrm{material}\:\mathrm{is}\:\mathrm{1}.\mathrm{22}\:\mathrm{mm}\:\mathrm{thick}\:\mathrm{and}\:\mathrm{has}\:\mathrm{a}? \\ $$$$\mathrm{coeffiecient}\:\mathrm{of}\:\mathrm{thermal}\:\mathrm{conductivity}\:\mathrm{of}\:\mathrm{0}.\mathrm{23}\:\mathrm{W}/\mathrm{Km}.\:\mathrm{the}\:\mathrm{electrical} \\ $$$$\mathrm{resistivity}\:\mathrm{of}\:\mathrm{the}\:\mathrm{material}\:\mathrm{of}\:\mathrm{the}\:\mathrm{wire}\:\mathrm{is}\:\mathrm{5}.\mathrm{2}\:×\mathrm{10}^{−\mathrm{7}} \Omega\mathrm{m}.\:\mathrm{find}\:\mathrm{the}\: \\ $$$$\mathrm{temperature}\:\mathrm{difference}\:\mathrm{between}\:\mathrm{the}\:\mathrm{inner}\:\mathrm{and}\:\mathrm{outer}\:\mathrm{surface}\:\mathrm{of}\: \\ $$$$\mathrm{the}\:\mathrm{insulated}\:\mathrm{material}\:\mathrm{when}\:\mathrm{steady}\:\mathrm{state}\:\mathrm{is}\:\mathrm{reached}. \\ $$

Question Number 99877 Answers: 0 Comments: 5

Question Number 99869 Answers: 2 Comments: 0

tng(𝛑/9) + 4sin(𝛑/9) =(√3)

$$\:\boldsymbol{{tng}}\frac{\boldsymbol{\pi}}{\mathrm{9}}\:\:+\:\mathrm{4}\boldsymbol{{sin}}\frac{\boldsymbol{\pi}}{\mathrm{9}}\:=\sqrt{\mathrm{3}} \\ $$

Question Number 99846 Answers: 0 Comments: 1

lim_(n→∞) (1−(1/(2!)))^(((1/(2!))−(1/(3!)))^(.........((1/(n!))−(1/((n+1)!)))) ) =?

$$\:\:\:\:\boldsymbol{{li}}\underset{\boldsymbol{{n}}\rightarrow\infty} {\boldsymbol{{m}}}\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}!}\right)^{\left(\frac{\mathrm{1}}{\mathrm{2}!}−\frac{\mathrm{1}}{\mathrm{3}!}\right)^{.........\left(\frac{\mathrm{1}}{\boldsymbol{{n}}!}−\frac{\mathrm{1}}{\left(\boldsymbol{{n}}+\mathrm{1}\right)!}\right)} } =? \\ $$

Question Number 99827 Answers: 0 Comments: 0

How many days after 12/7/1941 (pearl harbour bombed) was 9/11/2001 (the september 11 terrorist attack? please help, i′m having 27175days, which apparently isn′t correct.

$$\mathrm{How}\:\mathrm{many}\:\mathrm{days}\:\mathrm{after}\:\mathrm{12}/\mathrm{7}/\mathrm{1941} \\ $$$$\left(\mathrm{pearl}\:\mathrm{harbour}\:\mathrm{bombed}\right)\:\mathrm{was}\:\mathrm{9}/\mathrm{11}/\mathrm{2001} \\ $$$$\left(\mathrm{the}\:\mathrm{september}\:\mathrm{11}\:\mathrm{terrorist}\:\mathrm{attack}?\right. \\ $$$$ \\ $$$$\mathrm{please}\:\mathrm{help},\:\mathrm{i}'\mathrm{m}\:\mathrm{having}\:\mathrm{27175days},\: \\ $$$$\mathrm{which}\:\mathrm{apparently}\:\mathrm{isn}'\mathrm{t}\:\mathrm{correct}. \\ $$

Question Number 99807 Answers: 3 Comments: 0

Question Number 99803 Answers: 2 Comments: 0

Π_(k=1) ^∞ (1+(1/k^2 ))=? helpe me

$$\underset{\mathrm{k}=\mathrm{1}} {\overset{\infty} {\prod}}\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{k}^{\mathrm{2}} }\right)=? \\ $$$$\mathrm{helpe}\:\mathrm{me} \\ $$

Pg 254 Pg 255 Pg 256 Pg 257 Pg 258 Pg 259 Pg 260 Pg 261 Pg 262 Pg 263

Contact: info@tinkutara.com