
AlgebraQuestion and Answers: Page 254
Question Number 104782 Answers: 1 Comments: 0
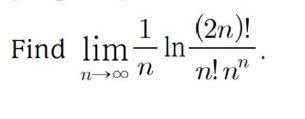
Question Number 104758 Answers: 0 Comments: 0

Question Number 104811 Answers: 2 Comments: 0
Question Number 104717 Answers: 0 Comments: 0
Question Number 104706 Answers: 1 Comments: 2
Question Number 104696 Answers: 1 Comments: 0

Question Number 105301 Answers: 3 Comments: 0
Question Number 104676 Answers: 3 Comments: 4

Question Number 104657 Answers: 1 Comments: 0
Question Number 104621 Answers: 1 Comments: 0
Question Number 104588 Answers: 1 Comments: 1
Question Number 104517 Answers: 2 Comments: 0

Question Number 104516 Answers: 1 Comments: 1
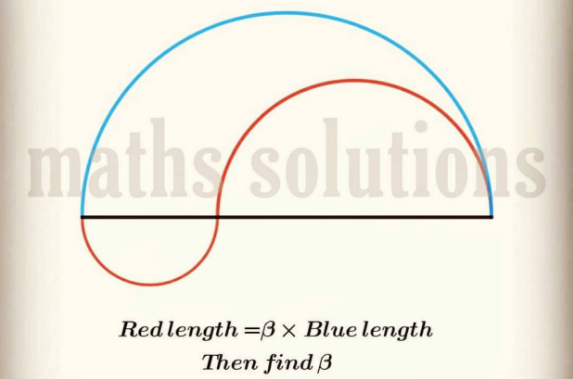
Question Number 104450 Answers: 1 Comments: 0
Question Number 104419 Answers: 2 Comments: 0
Question Number 104413 Answers: 2 Comments: 0
Question Number 104410 Answers: 1 Comments: 0
Question Number 104404 Answers: 1 Comments: 0
Question Number 104391 Answers: 0 Comments: 1
Question Number 104394 Answers: 1 Comments: 0

Question Number 104377 Answers: 1 Comments: 6
Question Number 104369 Answers: 1 Comments: 0

Question Number 104326 Answers: 1 Comments: 2
Question Number 104297 Answers: 1 Comments: 0
Question Number 104294 Answers: 1 Comments: 0
Question Number 104278 Answers: 1 Comments: 0
$${x}−\left(−\left(−{x}+\left(−{x}+{x}\right)\right)\right)=\:? \\ $$
Pg 249 Pg 250 Pg 251 Pg 252 Pg 253 Pg 254 Pg 255 Pg 256 Pg 257 Pg 258
