
AlgebraQuestion and Answers: Page 253
Question Number 106239 Answers: 1 Comments: 0
Question Number 106176 Answers: 3 Comments: 1
Question Number 106139 Answers: 0 Comments: 0
Question Number 106156 Answers: 2 Comments: 1
Question Number 106076 Answers: 2 Comments: 0
Question Number 106022 Answers: 0 Comments: 0

Question Number 105929 Answers: 1 Comments: 0

Question Number 105927 Answers: 3 Comments: 2
Question Number 105851 Answers: 1 Comments: 0
Question Number 105822 Answers: 2 Comments: 0
Question Number 105704 Answers: 1 Comments: 2
Question Number 105687 Answers: 3 Comments: 0
Question Number 105686 Answers: 1 Comments: 0
$${solve}\:\mathrm{7}^{{x}} \:+\:\mathrm{24}^{{x}} \:=\:\mathrm{25}^{{x}} \: \\ $$
Question Number 105634 Answers: 0 Comments: 0
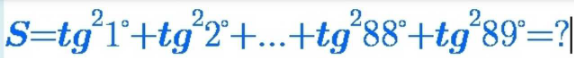
Question Number 105625 Answers: 1 Comments: 0
Question Number 105605 Answers: 2 Comments: 0
Question Number 105536 Answers: 1 Comments: 0

Question Number 105534 Answers: 1 Comments: 2

Question Number 105456 Answers: 2 Comments: 0
Question Number 105448 Answers: 3 Comments: 2
Question Number 105414 Answers: 0 Comments: 2
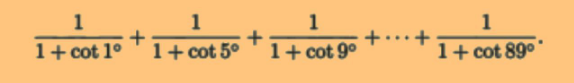
Question Number 105413 Answers: 0 Comments: 0

Question Number 105406 Answers: 0 Comments: 0

Question Number 105340 Answers: 1 Comments: 0
Question Number 105322 Answers: 0 Comments: 1

Question Number 105320 Answers: 0 Comments: 0
Pg 248 Pg 249 Pg 250 Pg 251 Pg 252 Pg 253 Pg 254 Pg 255 Pg 256 Pg 257
