Question and Answers Forum

AlgebraQuestion and Answers: Page 251

Question Number 107033 Answers: 0 Comments: 0

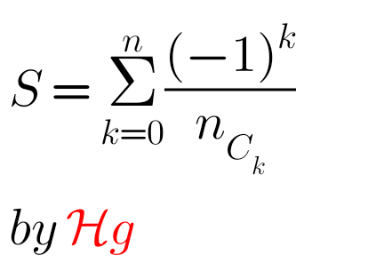
Question Number 106941 Answers: 1 Comments: 0

Find the maximum value of Σ_(i=1) ^n sin^5 θ_i with Σ_(i=1) ^n sin θ_i =0.

$${Find}\:{the}\:{maximum}\:{value}\:{of}\:\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}\mathrm{sin}^{\mathrm{5}} \:\theta_{{i}} \\ $$$${with}\:\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}\mathrm{sin}\:\theta_{{i}} =\mathrm{0}. \\ $$

Question Number 106943 Answers: 3 Comments: 1

Question Number 106907 Answers: 1 Comments: 0

Question Number 106906 Answers: 2 Comments: 0

Question Number 106816 Answers: 2 Comments: 1

prove by mathematical induction (1) n^2 ≤ 2^n ; n ≥ 4 (2) (n+1)^2 < 2n^2 ; n ≥ 3 (3) 2^n −3 ≥ 2^(n−2) ; n ≥ 5 @bemath@

$$\mathrm{prove}\:\mathrm{by}\:\mathrm{mathematical}\:\mathrm{induction} \\ $$$$\left(\mathrm{1}\right)\:\mathrm{n}^{\mathrm{2}} \:\leqslant\:\mathrm{2}^{\mathrm{n}} \:;\:\mathrm{n}\:\geqslant\:\mathrm{4} \\ $$$$\left(\mathrm{2}\right)\:\left(\mathrm{n}+\mathrm{1}\right)^{\mathrm{2}} \:<\:\mathrm{2n}^{\mathrm{2}} \:;\:\mathrm{n}\:\geqslant\:\mathrm{3} \\ $$$$\left(\mathrm{3}\right)\:\mathrm{2}^{\mathrm{n}} −\mathrm{3}\:\geqslant\:\mathrm{2}^{\mathrm{n}−\mathrm{2}} \:;\:\mathrm{n}\:\geqslant\:\mathrm{5} \\ $$$$\:\:\:\:\:\:@\mathrm{bemath}@ \\ $$

Question Number 106794 Answers: 3 Comments: 0

repost old question unanswer Given → { ((((4x^2 )/(1+4x^2 )) = y)),((((4y^2 )/(1+4y^2 )) = z)),((((4z^2 )/(1+4z^2 )) = x)) :}

$$\mathrm{repost}\:\mathrm{old}\:\mathrm{question}\:\mathrm{unanswer} \\ $$$$\mathcal{G}\mathrm{iven}\:\rightarrow\begin{cases}{\frac{\mathrm{4x}^{\mathrm{2}} }{\mathrm{1}+\mathrm{4x}^{\mathrm{2}} }\:=\:\mathrm{y}}\\{\frac{\mathrm{4y}^{\mathrm{2}} }{\mathrm{1}+\mathrm{4y}^{\mathrm{2}} }\:=\:\mathrm{z}}\\{\frac{\mathrm{4z}^{\mathrm{2}} }{\mathrm{1}+\mathrm{4z}^{\mathrm{2}} }\:=\:\mathrm{x}}\end{cases} \\ $$

Question Number 106745 Answers: 2 Comments: 8

Question Number 106730 Answers: 1 Comments: 0

Question Number 106727 Answers: 2 Comments: 0

Question Number 106774 Answers: 4 Comments: 0

^(@bemath@) (1) 3^x + 3^((√x) ) = 90. find x ? (2) x (dy/dx)−(1+x)y = xy^2

$$\:\:\:\:\overset{@\mathrm{bemath}@} {\:} \\ $$$$\:\left(\mathrm{1}\right)\:\:\:\:\mathrm{3}^{{x}} \:+\:\mathrm{3}^{\sqrt{{x}}\:} =\:\mathrm{90}.\:\mathrm{find}\:{x}\:?\: \\ $$$$\:\:\left(\mathrm{2}\right)\:\mathrm{x}\:\frac{\mathrm{dy}}{\mathrm{dx}}−\left(\mathrm{1}+\mathrm{x}\right)\mathrm{y}\:=\:\mathrm{xy}^{\mathrm{2}} \\ $$

Question Number 106659 Answers: 1 Comments: 0

How many terms has the polynomial; (X_1 +2X_2 −X_3 +34)^(10) ?

$$\mathrm{How}\:\mathrm{many}\:\mathrm{terms}\:\mathrm{has}\:\mathrm{the}\:\mathrm{polynomial}; \\ $$$$\left(\mathrm{X}_{\mathrm{1}} +\mathrm{2X}_{\mathrm{2}} −\mathrm{X}_{\mathrm{3}} +\mathrm{34}\right)^{\mathrm{10}} \:? \\ $$

Question Number 106655 Answers: 0 Comments: 1

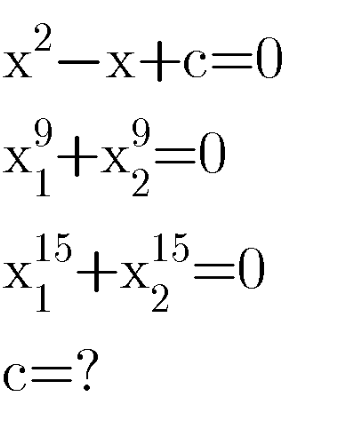
Question Number 106378 Answers: 2 Comments: 0

Question Number 106364 Answers: 1 Comments: 2

0^i =?????

$$\mathrm{0}^{{i}} =????? \\ $$

Question Number 106314 Answers: 3 Comments: 0

Question Number 106281 Answers: 2 Comments: 0

(√(x+50))+(√(y+100))+(√(z+150))=((x+y+z)/4)+78 find x+y−z

$$\sqrt{\mathrm{x}+\mathrm{50}}+\sqrt{\mathrm{y}+\mathrm{100}}+\sqrt{\mathrm{z}+\mathrm{150}}=\frac{\mathrm{x}+\mathrm{y}+\mathrm{z}}{\mathrm{4}}+\mathrm{78} \\ $$$$\mathrm{find}\:\mathrm{x}+\mathrm{y}−\mathrm{z}\: \\ $$

Question Number 106272 Answers: 1 Comments: 1

(√(1 + (√(5 +(√(11 + (√(19 + (√(29 + (√…))))))))))) = ?

$$\sqrt{\mathrm{1}\:+\:\sqrt{\mathrm{5}\:+\sqrt{\mathrm{11}\:+\:\sqrt{\mathrm{19}\:+\:\sqrt{\mathrm{29}\:+\:\sqrt{\ldots}}}}}}\:=\:? \\ $$

Question Number 106259 Answers: 2 Comments: 1

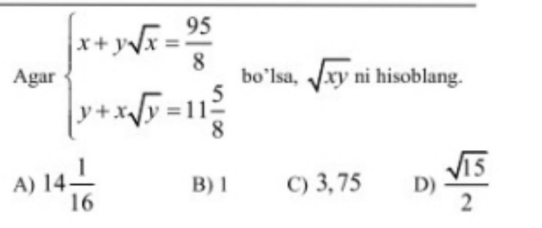
Question Number 106233 Answers: 2 Comments: 0

(x^2 + 1)(x − 1)^2 = 2017yz (y^2 + 1)(y − 1)^2 = 2017xz (z^2 + 1)(z − 1)^2 = 2017xy x ≥ 1 ; y ≥ 1 ; z ≥ 1 prove that x = y = z = ((1+ (√(2018)) + (√(2015+ 2(√(2018)))))/2)

$$\left({x}^{\mathrm{2}} \:+\:\mathrm{1}\right)\left({x}\:−\:\mathrm{1}\right)^{\mathrm{2}} \:=\:\mathrm{2017}{yz} \\ $$$$\left({y}^{\mathrm{2}} \:+\:\mathrm{1}\right)\left({y}\:−\:\mathrm{1}\right)^{\mathrm{2}} \:=\:\mathrm{2017}{xz} \\ $$$$\left({z}^{\mathrm{2}} \:+\:\mathrm{1}\right)\left({z}\:−\:\mathrm{1}\right)^{\mathrm{2}} \:=\:\mathrm{2017}{xy} \\ $$$${x}\:\geqslant\:\mathrm{1}\:;\:{y}\:\geqslant\:\mathrm{1}\:;\:{z}\:\geqslant\:\mathrm{1} \\ $$$${prove}\:{that}\:\:\:{x}\:=\:{y}\:=\:{z}\:= \\ $$$$\frac{\mathrm{1}+\:\sqrt{\mathrm{2018}}\:+\:\sqrt{\mathrm{2015}+\:\mathrm{2}\sqrt{\mathrm{2018}}}}{\mathrm{2}} \\ $$

Question Number 106188 Answers: 2 Comments: 0

Solve for x x^x^(...x^a ) =a with a∈R^+

$${Solve}\:{for}\:{x} \\ $$$${x}^{{x}^{...{x}^{{a}} } } ={a}\:{with}\:{a}\in\mathbb{R}^{+} \\ $$

Question Number 106239 Answers: 1 Comments: 0

A rope of length 10cm is used to form as ector of circle of radius 35cm What ish te size of the angle of the sector

$$ \\ $$$$\mathrm{A}\:\mathrm{rope}\:\mathrm{of}\:\mathrm{length}\:\mathrm{10cm}\:\mathrm{is}\:\mathrm{used}\:\mathrm{to}\:\mathrm{form}\:\mathrm{as} \\ $$$$\mathrm{ector}\:\mathrm{of}\:\mathrm{circle}\:\mathrm{of}\:\mathrm{radius}\:\mathrm{35cm}\:\mathrm{What}\:\mathrm{ish} \\ $$$$\mathrm{te}\:\mathrm{size}\:\mathrm{of}\:\mathrm{the}\:\mathrm{angle}\:\mathrm{of}\:\mathrm{the}\:\mathrm{sector} \\ $$

Question Number 106176 Answers: 3 Comments: 1

If 20 men can lay 36m of a pipe in 8 hours. How long would 25 men take to lay the next 54m of the pipe?

$$\mathrm{If}\:\mathrm{20}\:\mathrm{men}\:\mathrm{can}\:\mathrm{lay}\:\mathrm{36m}\:\mathrm{of}\:\mathrm{a}\:\mathrm{pipe} \\ $$$$\mathrm{in}\:\mathrm{8}\:\mathrm{hours}.\:\mathrm{How}\:\mathrm{long}\:\mathrm{would}\:\mathrm{25} \\ $$$$\mathrm{men}\:\mathrm{take}\:\mathrm{to}\:\mathrm{lay}\:\mathrm{the}\:\mathrm{next}\:\mathrm{54m}\:\mathrm{of} \\ $$$$\mathrm{the}\:\mathrm{pipe}? \\ $$

Question Number 106139 Answers: 0 Comments: 0

a,b∈Z ^3 (√(9^3 (√2) − 9)) = 1 −^3 (√a) +^3 (√b), a = ? b = ?

$${a},{b}\in\mathbb{Z}\:\:\:\:\:^{\mathrm{3}} \sqrt{\mathrm{9}\:^{\mathrm{3}} \sqrt{\mathrm{2}}\:−\:\mathrm{9}}\:=\:\mathrm{1}\:−\:^{\mathrm{3}} \sqrt{{a}}\:+\:^{\mathrm{3}} \sqrt{{b}},\:\:\:\:\:{a}\:=\:?\:\:\:\:\:\:{b}\:=\:? \\ $$

Question Number 106156 Answers: 2 Comments: 1

Given { (((√(xy)) +(1/(√x)) +(1/(√y)) =9)),(((√x)+(√y) = 20)) :} where x > y. find the value of x(√y) −y(√x) .

$$\mathrm{Given}\:\begin{cases}{\sqrt{\mathrm{xy}}\:+\frac{\mathrm{1}}{\sqrt{\mathrm{x}}}\:+\frac{\mathrm{1}}{\sqrt{\mathrm{y}}}\:=\mathrm{9}}\\{\sqrt{\mathrm{x}}+\sqrt{\mathrm{y}}\:=\:\mathrm{20}}\end{cases} \\ $$$$\mathrm{where}\:\mathrm{x}\:>\:\mathrm{y}.\:\mathrm{find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of} \\ $$$$\mathrm{x}\sqrt{\mathrm{y}}\:−\mathrm{y}\sqrt{\mathrm{x}}\:. \\ $$

Question Number 106076 Answers: 2 Comments: 0

solve this pls x^x^(1/2) =(1/2)

$${solve}\:{this}\:{pls} \\ $$$${x}^{{x}^{\frac{\mathrm{1}}{\mathrm{2}}} } =\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Pg 246 Pg 247 Pg 248 Pg 249 Pg 250 Pg 251 Pg 252 Pg 253 Pg 254 Pg 255

Contact: info@tinkutara.com