Question and Answers Forum

All Questions Topic List

AlgebraQuestion and Answers: Page 247

Question Number 110399 Answers: 0 Comments: 5

The identity 2[16a^4 +81b^4 +c^4 ]=[4a^2 +9b^2 +c^2 ]^2 cannot result from which of the following equations? A. 6b=4a+2c B. 6a=9b+3c C. 6b=−4a+2c D.c= −2a−3b E. 6c=2b+3a

$$\mathrm{The}\:\mathrm{identity} \\ $$$$\mathrm{2}\left[\mathrm{16a}^{\mathrm{4}} +\mathrm{81b}^{\mathrm{4}} +\mathrm{c}^{\mathrm{4}} \right]=\left[\mathrm{4a}^{\mathrm{2}} +\mathrm{9b}^{\mathrm{2}} +\mathrm{c}^{\mathrm{2}} \right]^{\mathrm{2}} \\ $$$$\mathrm{cannot}\:\mathrm{result}\:\mathrm{from}\:\mathrm{which}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{following}\:\mathrm{equations}?\: \\ $$$$\mathrm{A}.\:\mathrm{6b}=\mathrm{4a}+\mathrm{2c}\:\mathrm{B}.\:\mathrm{6a}=\mathrm{9b}+\mathrm{3c}\:\mathrm{C}. \\ $$$$\mathrm{6b}=−\mathrm{4a}+\mathrm{2c}\:\mathrm{D}.\mathrm{c}=\:−\mathrm{2a}−\mathrm{3b}\:\mathrm{E}. \\ $$$$\mathrm{6c}=\mathrm{2b}+\mathrm{3a} \\ $$

Question Number 110374 Answers: 2 Comments: 0

If P(x) is a polynomial whose sum of coefficients is 3 and P(x) can be factorised into two polynomials Q(x),R(x) with integer coefficients, the sum of the coefficients Q(x)^2 +R(x)^2 is

$$\mathrm{If}\:\mathrm{P}\left(\mathrm{x}\right)\:\mathrm{is}\:\mathrm{a}\:\mathrm{polynomial}\:\mathrm{whose}\:\mathrm{sum}\:\mathrm{of} \\ $$$$\mathrm{coefficients}\:\mathrm{is}\:\mathrm{3}\:\mathrm{and}\:\mathrm{P}\left(\mathrm{x}\right)\:\mathrm{can}\:\mathrm{be} \\ $$$$\mathrm{factorised}\:\mathrm{into}\:\mathrm{two}\:\mathrm{polynomials} \\ $$$$\mathrm{Q}\left(\mathrm{x}\right),\mathrm{R}\left(\mathrm{x}\right)\:\mathrm{with}\:\mathrm{integer}\:\mathrm{coefficients}, \\ $$$$\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{the}\:\mathrm{coefficients} \\ $$$$\mathrm{Q}\left(\mathrm{x}\right)^{\mathrm{2}} +\mathrm{R}\left(\mathrm{x}\right)^{\mathrm{2}} \:\mathrm{is} \\ $$

Question Number 110359 Answers: 1 Comments: 0

Let f(x)=∣x−2∣+∣x−4∣−∣2x−6∣, for 2≤x≤8. The sum of the largest and smallest values of f(x) is

$$\mathrm{Let} \\ $$$$\mathrm{f}\left(\mathrm{x}\right)=\mid\mathrm{x}−\mathrm{2}\mid+\mid\mathrm{x}−\mathrm{4}\mid−\mid\mathrm{2x}−\mathrm{6}\mid, \\ $$$$\mathrm{for}\:\mathrm{2}\leqslant\mathrm{x}\leqslant\mathrm{8}.\:\mathrm{The}\:\mathrm{sum}\:\mathrm{of} \\ $$$$\mathrm{the}\:\mathrm{largest}\:\mathrm{and} \\ $$$$\mathrm{smallest}\:\mathrm{values}\:\mathrm{of}\:\mathrm{f}\left(\mathrm{x}\right)\:\mathrm{is} \\ $$

Question Number 110318 Answers: 1 Comments: 0

(a+b−c)^2 =??

$$\left({a}+{b}−{c}\right)^{\mathrm{2}} =?? \\ $$

Question Number 110299 Answers: 3 Comments: 0

Question Number 110294 Answers: 1 Comments: 0

∣2x+1∣−∣x−2∣ < 4 find the solution set

$$\mid\mathrm{2}{x}+\mathrm{1}\mid−\mid{x}−\mathrm{2}\mid\:<\:\mathrm{4}\: \\ $$$${find}\:{the}\:{solution}\:{set} \\ $$

Question Number 110269 Answers: 1 Comments: 4

Question Number 110268 Answers: 0 Comments: 3

Question Number 110265 Answers: 0 Comments: 0

Question Number 110246 Answers: 1 Comments: 0

solve for z∈C: (a+bi)^z =b+ai

$${solve}\:{for}\:{z}\in\mathbb{C}:\:\left({a}+{bi}\right)^{{z}} ={b}+{ai} \\ $$

Question Number 110219 Answers: 0 Comments: 3

let A and C be two none empety set prove that A⊆B ∧ C ⊆D iff A×C ⊆B×D? help me sir

$${let}\:{A}\:{and}\:{C}\:{be}\:{two}\:{none}\:{empety}\:{set}\:{prove}\:{that} \\ $$$${A}\subseteq{B}\:\wedge\:{C}\:\subseteq{D}\:{iff}\:{A}×{C}\:\subseteq{B}×{D}? \\ $$$${help}\:{me}\:{sir} \\ $$

Question Number 110182 Answers: 1 Comments: 2

Solve x^3 +15x−92=0

$$\mathrm{Solve}\:{x}^{\mathrm{3}} +\mathrm{15}{x}−\mathrm{92}=\mathrm{0} \\ $$

Question Number 110173 Answers: 2 Comments: 0

If Σ_(r=1) ^n t_r =((n(n+1)(n+2)(n+3))/8) then lim_(n→∞) Σ_(r=1) ^n (1/t_r ) = ?

$${If}\:\:\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}{t}_{{r}} =\frac{{n}\left({n}+\mathrm{1}\right)\left({n}+\mathrm{2}\right)\left({n}+\mathrm{3}\right)}{\mathrm{8}} \\ $$$${then}\:\:\:\:\underset{{n}\rightarrow\infty} {\mathrm{lim}}\:\:\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\:\frac{\mathrm{1}}{{t}_{{r}} }\:=\:? \\ $$$$ \\ $$

Question Number 110157 Answers: 2 Comments: 0

If we have 5 people, how many ways can they be seated on a round table, if there are, (a) 7 chairs (b) 3 chairs available

$$\mathrm{If}\:\mathrm{we}\:\mathrm{have}\:\:\mathrm{5}\:\:\mathrm{people},\:\mathrm{how}\:\mathrm{many}\:\mathrm{ways}\:\mathrm{can}\:\mathrm{they}\:\mathrm{be}\:\mathrm{seated} \\ $$$$\mathrm{on}\:\mathrm{a}\:\mathrm{round}\:\mathrm{table},\:\mathrm{if}\:\mathrm{there}\:\mathrm{are}, \\ $$$$\left(\mathrm{a}\right)\:\:\:\mathrm{7}\:\:\mathrm{chairs}\:\:\:\:\:\:\:\:\:\left(\mathrm{b}\right)\:\:\:\:\mathrm{3}\:\:\mathrm{chairs}\:\:\:\:\:\:\:\:\:\:\:\mathrm{available} \\ $$

Question Number 110156 Answers: 1 Comments: 0

If we have 5 people, how many ways can they be seated in a row on a chair, if their are, (a) 7 chairs (b) 3 chairs available

$$\mathrm{If}\:\mathrm{we}\:\mathrm{have}\:\:\mathrm{5}\:\mathrm{people},\:\mathrm{how}\:\mathrm{many}\:\mathrm{ways}\:\mathrm{can}\:\mathrm{they}\:\mathrm{be}\:\mathrm{seated}\:\mathrm{in}\:\mathrm{a}\:\mathrm{row} \\ $$$$\mathrm{on}\:\mathrm{a}\:\mathrm{chair},\:\mathrm{if}\:\mathrm{their}\:\mathrm{are}, \\ $$$$\left(\mathrm{a}\right)\:\:\:\mathrm{7}\:\:\mathrm{chairs}\:\:\:\:\:\:\:\left(\mathrm{b}\right)\:\:\:\:\mathrm{3}\:\:\mathrm{chairs}\:\:\:\:\:\:\:\:\mathrm{available} \\ $$

Question Number 110136 Answers: 4 Comments: 0

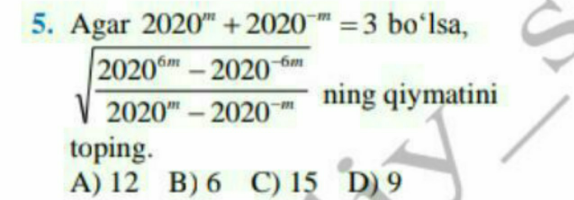
Question Number 110087 Answers: 0 Comments: 3

Solve the system following of equations { ((x+y+z=2)),((2x+3y+z=1)),((x^2 +(y+2)^2 +(z−1)^2 =9)) :}

$$\mathrm{Solve}\:\mathrm{the}\:\mathrm{system}\:\mathrm{following}\:\mathrm{of}\:\mathrm{equations} \\ $$$$\begin{cases}{\mathrm{x}+\mathrm{y}+\mathrm{z}=\mathrm{2}}\\{\mathrm{2x}+\mathrm{3y}+\mathrm{z}=\mathrm{1}}\\{\mathrm{x}^{\mathrm{2}} +\left(\mathrm{y}+\mathrm{2}\right)^{\mathrm{2}} +\left(\mathrm{z}−\mathrm{1}\right)^{\mathrm{2}} =\mathrm{9}}\end{cases} \\ $$

Question Number 109954 Answers: 1 Comments: 0

!3=????

$$!\mathrm{3}=???? \\ $$

Question Number 109914 Answers: 1 Comments: 0

Prove that tan142°30′+(√6)+(√3)−(√2) is an integer.

$$\mathrm{Prove}\:\mathrm{that}\:\mathrm{tan142}°\mathrm{30}'+\sqrt{\mathrm{6}}+\sqrt{\mathrm{3}}−\sqrt{\mathrm{2}} \\ $$$$\mathrm{is}\:\mathrm{an}\:\mathrm{integer}. \\ $$

Question Number 109901 Answers: 1 Comments: 0

If first n terms of an A.P. is cn^2 , find sum of squares of its first n terms.

$${If}\:{first}\:{n}\:{terms}\:{of}\:{an}\:{A}.{P}.\:{is}\:{cn}^{\mathrm{2}} , \\ $$$${find}\:{sum}\:{of}\:{squares}\:{of}\:{its}\:{first} \\ $$$${n}\:{terms}. \\ $$

Question Number 109895 Answers: 1 Comments: 3

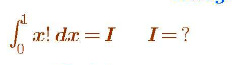
Question Number 109754 Answers: 1 Comments: 0

1.specify value absolute x if ? b.∣2x+3∣+x−3=0

$$\mathrm{1}.{specify}\:{value}\:{absolute}\:{x}\:{if}\:? \\ $$$$ \\ $$$${b}.\mid\mathrm{2}{x}+\mathrm{3}\mid+{x}−\mathrm{3}=\mathrm{0} \\ $$$$ \\ $$

Question Number 109721 Answers: 1 Comments: 0

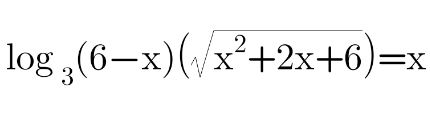
Question Number 109699 Answers: 1 Comments: 0

x(x−1)^2 ≥ 12(x−1)

$$\:\:\:\:\:{x}\left({x}−\mathrm{1}\right)^{\mathrm{2}} \:\geqslant\:\mathrm{12}\left({x}−\mathrm{1}\right) \\ $$

Question Number 109626 Answers: 1 Comments: 1

Question Number 109625 Answers: 0 Comments: 2

((sin 2𝛂+2sin 𝛂∙cos 2𝛂)/(1+cos 𝛂+cos2 𝛂+cos3 𝛂))

$$\frac{\mathrm{sin}\:\mathrm{2}\boldsymbol{\alpha}+\mathrm{2sin}\:\boldsymbol{\alpha}\centerdot\mathrm{cos}\:\mathrm{2}\boldsymbol{\alpha}}{\mathrm{1}+\mathrm{cos}\:\boldsymbol{\alpha}+\mathrm{cos2}\:\boldsymbol{\alpha}+\mathrm{cos3}\:\boldsymbol{\alpha}} \\ $$

Pg 242 Pg 243 Pg 244 Pg 245 Pg 246 Pg 247 Pg 248 Pg 249 Pg 250 Pg 251

Terms of Service

Contact: info@tinkutara.com