
AlgebraQuestion and Answers: Page 242
Question Number 116192 Answers: 2 Comments: 1

Question Number 116173 Answers: 0 Comments: 2
Question Number 116163 Answers: 1 Comments: 0
Question Number 116138 Answers: 3 Comments: 1
Question Number 116226 Answers: 2 Comments: 1
Question Number 116092 Answers: 1 Comments: 2
$${Solve}\:{for}\:{x}\:{and}\:{y}\:{if} \\ $$$${x}^{{y}} =\mathrm{36} \\ $$
Question Number 116091 Answers: 2 Comments: 0
Question Number 116059 Answers: 2 Comments: 0
$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}!}{\mathrm{3}^{{n}+\mathrm{1}} } \\ $$
Question Number 116057 Answers: 2 Comments: 0
Question Number 116056 Answers: 2 Comments: 0
$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{5}^{{n}} }{{n}!}=? \\ $$
Question Number 115986 Answers: 1 Comments: 0
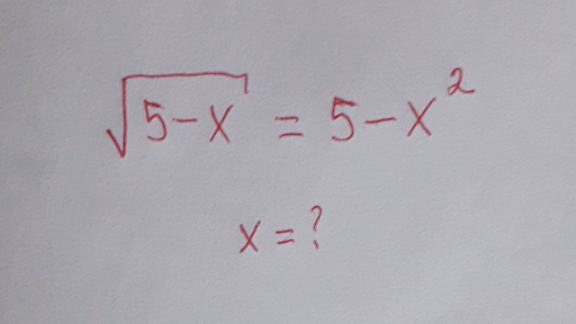
Question Number 115916 Answers: 1 Comments: 0

Question Number 115909 Answers: 1 Comments: 0
Question Number 115908 Answers: 1 Comments: 2
Question Number 115906 Answers: 1 Comments: 0
Question Number 115897 Answers: 2 Comments: 1
Question Number 115858 Answers: 2 Comments: 0
Question Number 115857 Answers: 1 Comments: 0
Question Number 115871 Answers: 1 Comments: 1

Question Number 115815 Answers: 1 Comments: 0
Question Number 115773 Answers: 0 Comments: 0
Question Number 115564 Answers: 1 Comments: 0

Question Number 115544 Answers: 1 Comments: 1
Question Number 115484 Answers: 1 Comments: 3
Question Number 115436 Answers: 1 Comments: 0
Question Number 115333 Answers: 1 Comments: 3

Pg 237 Pg 238 Pg 239 Pg 240 Pg 241 Pg 242 Pg 243 Pg 244 Pg 245 Pg 246
