Question and Answers Forum

AlgebraQuestion and Answers: Page 240

Question Number 118280 Answers: 0 Comments: 1

(26) OA^(→) = a^→ = (((4.8)),((3.6)) ) , OB^(→) = b^→ = ((( 8)),((15)) ) a^→ . b^→ = (4.8)(8) + (3.6)(15) = 92.4^(→ ) a = (√(4.8^2 +3.6^2 )) = (√(23.04+12.96)) = (√(36)) = 6 b = (√(8^2 +15^2 )) = (√(64+225)) = (√(289)) = 17 a.b = 6 . 17 = 102 cos AOB = ((a^→ .b^→ )/(a.b)) = ((92.4)/(102)) = 0.9059 = cos 25.06° ∴ ∠AOB = 25.06°

$$\left(\mathrm{26}\right)\:\:\:\overset{\rightarrow} {\mathrm{OA}}\:=\:\overset{\rightarrow} {\mathrm{a}}\:=\:\begin{pmatrix}{\mathrm{4}.\mathrm{8}}\\{\mathrm{3}.\mathrm{6}}\end{pmatrix}\:\:,\:\:\overset{\rightarrow} {\mathrm{OB}}\:=\:\overset{\rightarrow} {\mathrm{b}}\:=\:\begin{pmatrix}{\:\:\mathrm{8}}\\{\mathrm{15}}\end{pmatrix} \\ $$$$\:\:\:\:\:\:\:\:\:\:\overset{\rightarrow} {\mathrm{a}}.\:\overset{\rightarrow} {\mathrm{b}}\:=\:\left(\mathrm{4}.\mathrm{8}\right)\left(\mathrm{8}\right)\:+\:\left(\mathrm{3}.\mathrm{6}\right)\left(\mathrm{15}\right)\:=\:\mathrm{92}.\overset{\rightarrow\:} {\mathrm{4}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{a}\:=\:\sqrt{\mathrm{4}.\mathrm{8}^{\mathrm{2}} +\mathrm{3}.\mathrm{6}^{\mathrm{2}} }\:=\:\:\sqrt{\mathrm{23}.\mathrm{04}+\mathrm{12}.\mathrm{96}}\:=\:\sqrt{\mathrm{36}}\:=\:\mathrm{6} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{b}\:=\:\sqrt{\mathrm{8}^{\mathrm{2}} +\mathrm{15}^{\mathrm{2}} }\:=\:\sqrt{\mathrm{64}+\mathrm{225}}\:=\:\sqrt{\mathrm{289}}\:=\:\mathrm{17} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{a}.\mathrm{b}\:=\:\mathrm{6}\:.\:\mathrm{17}\:=\:\mathrm{102} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\mathrm{cos}\:\mathrm{AOB}\:=\:\frac{\overset{\rightarrow} {\mathrm{a}}.\overset{\rightarrow} {\mathrm{b}}}{\mathrm{a}.\mathrm{b}}\:=\:\frac{\mathrm{92}.\mathrm{4}}{\mathrm{102}}\:=\:\mathrm{0}.\mathrm{9059}\:=\:\mathrm{cos}\:\mathrm{25}.\mathrm{06}° \\ $$$$\:\:\:\:\:\:\:\:\:\:\therefore\:\:\angle\mathrm{AOB}\:=\:\mathrm{25}.\mathrm{06}° \\ $$

Question Number 118277 Answers: 0 Comments: 0

(23) Let a^→ = ((( 7)),((24)) ) and b^→ = ((( 3)),((−4)) ) a^→ .b^→ = (7)(3) + (24)(−4) = 21−96 = −75 a = (√(7^2 +24^2 )) = (√(49+576)) = (√(625)) = 25 b = (√(3^2 +(−4)^2 )) = (√(9+16)) = (√(25)) = 5 a.b = 25 . 5 = 125 Let θ be the angle between a^→ and b^→ . cosθ = ((a^→ .b^→ )/(a.b)) = ((−75)/(125)) = −0.6 = cos126.87° ∴ θ = 126.87°

$$\left(\mathrm{23}\right)\:\mathrm{Let}\:\overset{\rightarrow} {\mathrm{a}}\:=\:\begin{pmatrix}{\:\:\mathrm{7}}\\{\mathrm{24}}\end{pmatrix}\:\:\:\mathrm{and}\:\:\overset{\rightarrow} {\mathrm{b}}\:=\:\begin{pmatrix}{\:\:\:\mathrm{3}}\\{−\mathrm{4}}\end{pmatrix} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\overset{\rightarrow} {\mathrm{a}}.\overset{\rightarrow} {\mathrm{b}}\:=\:\left(\mathrm{7}\right)\left(\mathrm{3}\right)\:+\:\left(\mathrm{24}\right)\left(−\mathrm{4}\right)\:=\:\mathrm{21}−\mathrm{96}\:=\:−\mathrm{75} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{a}\:=\:\:\sqrt{\mathrm{7}^{\mathrm{2}} +\mathrm{24}^{\mathrm{2}} }\:=\:\sqrt{\mathrm{49}+\mathrm{576}}\:=\:\sqrt{\mathrm{625}}\:=\:\mathrm{25} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{b}\:=\:\:\sqrt{\mathrm{3}^{\mathrm{2}} +\left(−\mathrm{4}\right)^{\mathrm{2}} }\:=\:\sqrt{\mathrm{9}+\mathrm{16}}\:=\:\sqrt{\mathrm{25}}\:=\:\mathrm{5} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{a}.\mathrm{b}\:=\:\mathrm{25}\:.\:\mathrm{5}\:=\:\:\mathrm{125}\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{Let}\:\theta\:\mathrm{be}\:\mathrm{the}\:\mathrm{angle}\:\mathrm{between}\:\overset{\rightarrow} {\mathrm{a}}\:\mathrm{and}\:\overset{\rightarrow} {\mathrm{b}}. \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{cos}\theta\:=\:\frac{\overset{\rightarrow} {\mathrm{a}}.\overset{\rightarrow} {\mathrm{b}}}{\mathrm{a}.\mathrm{b}}\:=\:\frac{−\mathrm{75}}{\mathrm{125}}\:=\:−\mathrm{0}.\mathrm{6}\:=\:\mathrm{cos126}.\mathrm{87}° \\ $$$$\:\:\:\:\:\:\:\:\:\:\therefore\:\:\theta\:=\:\mathrm{126}.\mathrm{87}°\:\:\:\: \\ $$

Question Number 118275 Answers: 1 Comments: 1

Question Number 118263 Answers: 0 Comments: 0

Question Number 118227 Answers: 1 Comments: 0

If x = (√(42−(√(42−(√(42−...)))))) y = (√(x+(√(x+(√(x+...)))))) z=(√(y.(√(y.(√(y.(√(y...)))))))) . Find x+y+z .

$${If}\:{x}\:=\:\sqrt{\mathrm{42}−\sqrt{\mathrm{42}−\sqrt{\mathrm{42}−...}}} \\ $$$${y}\:=\:\sqrt{{x}+\sqrt{{x}+\sqrt{{x}+...}}} \\ $$$${z}=\sqrt{{y}.\sqrt{{y}.\sqrt{{y}.\sqrt{{y}...}}}}\:.\:{Find}\:{x}+{y}+{z}\:. \\ $$

Question Number 118196 Answers: 1 Comments: 0

solve in N: b^3 (2b^2 +2b+1)=18360

$${solve}\:{in}\:\mathbb{N}: \\ $$$${b}^{\mathrm{3}} \left(\mathrm{2}{b}^{\mathrm{2}} +\mathrm{2}{b}+\mathrm{1}\right)=\mathrm{18360} \\ $$

Question Number 118193 Answers: 1 Comments: 0

Given A=n^2 −2n+2 , B=n^2 +2n+2 n ∈ N^∗ −{1}. Show that ∀ divisor of A which divise n can also divise 2. Show that all common divisor of A and B can divise 4n.

$${Given}\:{A}={n}^{\mathrm{2}} −\mathrm{2}{n}+\mathrm{2}\:,\:{B}={n}^{\mathrm{2}} +\mathrm{2}{n}+\mathrm{2} \\ $$$${n}\:\in\:\mathbb{N}^{\ast} −\left\{\mathrm{1}\right\}. \\ $$$${Show}\:{that}\:\forall\:{divisor}\:{of}\:{A}\:{which}\:{divise} \\ $$$${n}\:{can}\:{also}\:{divise}\:\mathrm{2}. \\ $$$${Show}\:{that}\:{all}\:{common}\:{divisor}\:{of}\: \\ $$$${A}\:{and}\:{B}\:{can}\:{divise}\:\mathrm{4}{n}. \\ $$

Question Number 118184 Answers: 2 Comments: 1

factorise x^4 +4

$${factorise}\:{x}^{\mathrm{4}} +\mathrm{4} \\ $$

Question Number 118181 Answers: 1 Comments: 1

find all numbers >1 from N which their cube are <18360

$${find}\:{all}\:{numbers}\:>\mathrm{1}\:{from}\:\mathbb{N}\:{which} \\ $$$${their}\:{cube}\:{are}\:<\mathrm{18360} \\ $$

Question Number 118180 Answers: 1 Comments: 0

show that if n is odd , n(n^2 +3) is even.

$${show}\:{that}\:{if}\:{n}\:{is}\:{odd}\:,\:{n}\left({n}^{\mathrm{2}} +\mathrm{3}\right)\:{is}\:{even}. \\ $$

Question Number 118172 Answers: 1 Comments: 0

Find the area of a rhombus with side 8 cm

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{area}\:\mathrm{of}\:\mathrm{a}\:\mathrm{rhombus}\:\mathrm{with}\:\mathrm{side}\:\:\mathrm{8}\:\mathrm{cm} \\ $$

Question Number 118023 Answers: 1 Comments: 0

..calculus.. x,y,z ∈R^+ and x^2 +y^2 +z^2 =1 find min_(x,y,z∈R^(+ ) ) ((((yz)/x)+((xz)/y)+((xy)/z)) )=? m.n.1970..

$$ \\ $$$$\:\:\:\:\:\:\:..{calculus}.. \\ $$$$\:\:{x},{y},{z}\:\in\mathbb{R}^{+} \:\:{and}\:\:{x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} \:=\mathrm{1} \\ $$$$\:\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:{find}\:\:\:\:\:\: \\ $$$$\:\:\:\:{min}_{{x},{y},{z}\in\mathbb{R}^{+\:\:\:\:} } \left(\left(\frac{{yz}}{{x}}+\frac{{xz}}{{y}}+\frac{{xy}}{{z}}\right)\:\right)=? \\ $$$$ \\ $$$$\:\:\:\:\:\:\:{m}.{n}.\mathrm{1970}.. \\ $$

Question Number 118011 Answers: 4 Comments: 0

Question Number 118002 Answers: 1 Comments: 0

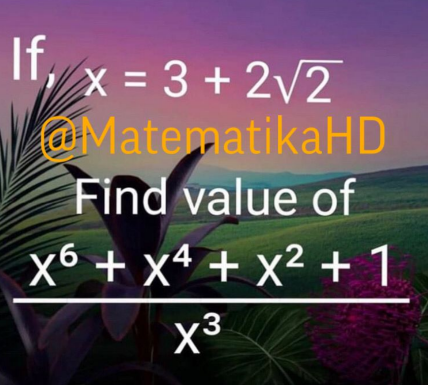
Question Number 117984 Answers: 1 Comments: 0

If f(x) is a polynomial function satisfying the relation f(x)+f((1/x))=f(x)f((1/x)) for all 0≠x∈R and if f(2)=9, then f(6) is (A) 216 (B) 217 (C) 126 (D) 127

$$\mathrm{If}\:{f}\left({x}\right)\:\mathrm{is}\:\mathrm{a}\:\mathrm{polynomial}\:\mathrm{function}\:\mathrm{satisfying}\:\mathrm{the}\:\mathrm{relation} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{f}\left({x}\right)+{f}\left(\frac{\mathrm{1}}{{x}}\right)={f}\left({x}\right){f}\left(\frac{\mathrm{1}}{{x}}\right) \\ $$$$\mathrm{for}\:\mathrm{all}\:\mathrm{0}\neq{x}\in\mathbb{R}\:\mathrm{and}\:\mathrm{if}\:{f}\left(\mathrm{2}\right)=\mathrm{9},\:\mathrm{then}\:\mathrm{f}\left(\mathrm{6}\right)\:\mathrm{is} \\ $$$$\left(\mathrm{A}\right)\:\mathrm{216}\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{B}\right)\:\mathrm{217}\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{C}\right)\:\mathrm{126}\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{D}\right)\:\mathrm{127} \\ $$

Question Number 117972 Answers: 2 Comments: 0

The number of surjections of {1,2,3,4} onto {x,y} is (A) 16 (B) 8 (C) 14 (D) 6

$$\mathrm{The}\:\mathrm{number}\:\mathrm{of}\:\mathrm{surjections}\:\mathrm{of}\:\left\{\mathrm{1},\mathrm{2},\mathrm{3},\mathrm{4}\right\}\:\mathrm{onto}\:\left\{\mathrm{x},\mathrm{y}\right\}\:\mathrm{is} \\ $$$$\left(\mathrm{A}\right)\:\mathrm{16}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{B}\right)\:\mathrm{8}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{C}\right)\:\mathrm{14}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{D}\right)\:\mathrm{6} \\ $$

Question Number 117934 Answers: 1 Comments: 0

Let f : [1,∞)→[2,∞) be the function defined by f(x)=x+(1/x) If g : [2,∞)→[1,∞), is a function such that (g○f)(x)=x for all x≥1. Show that g(t)=((t+(√(t^2 −4)))/2)

$$\mathrm{Let}\:{f}\::\:\left[\mathrm{1},\infty\right)\rightarrow\left[\mathrm{2},\infty\right)\:\mathrm{be}\:\mathrm{the}\:\mathrm{function}\:\mathrm{defined}\:\mathrm{by} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{f}\left({x}\right)={x}+\frac{\mathrm{1}}{{x}} \\ $$$$\mathrm{If}\:\mathrm{g}\::\:\left[\mathrm{2},\infty\right)\rightarrow\left[\mathrm{1},\infty\right),\:\mathrm{is}\:\mathrm{a}\:\mathrm{function}\:\mathrm{such}\:\mathrm{that}\:\left(\mathrm{g}\circ{f}\right)\left({x}\right)={x} \\ $$$$\mathrm{for}\:\mathrm{all}\:{x}\geqslant\mathrm{1}.\:\mathrm{Show}\:\mathrm{that}\:\mathrm{g}\left({t}\right)=\frac{{t}+\sqrt{{t}^{\mathrm{2}} −\mathrm{4}}}{\mathrm{2}} \\ $$

Question Number 117828 Answers: 1 Comments: 0

1)((√3)−1)((√3)+1)=(√3)×(√3)−(√3)−1 =3−(√3)−1 =2−(√3) 2)(2x+(√3))(2x−(√3))=(2x)^2 −2x(√3)+2x(√3)−3 =4x^2 −3

$$\left.\mathrm{1}\right)\left(\sqrt{\mathrm{3}}−\mathrm{1}\right)\left(\sqrt{\mathrm{3}}+\mathrm{1}\right)=\sqrt{\mathrm{3}}×\sqrt{\mathrm{3}}−\sqrt{\mathrm{3}}−\mathrm{1} \\ $$$$=\mathrm{3}−\sqrt{\mathrm{3}}−\mathrm{1} \\ $$$$=\mathrm{2}−\sqrt{\mathrm{3}} \\ $$$$\left.\mathrm{2}\right)\left(\mathrm{2}{x}+\sqrt{\mathrm{3}}\right)\left(\mathrm{2}{x}−\sqrt{\mathrm{3}}\right)=\left(\mathrm{2}{x}\right)^{\mathrm{2}} −\mathrm{2}{x}\sqrt{\mathrm{3}}+\mathrm{2}{x}\sqrt{\mathrm{3}}−\mathrm{3} \\ $$$$=\mathrm{4}{x}^{\mathrm{2}} −\mathrm{3} \\ $$

Question Number 117827 Answers: 0 Comments: 5

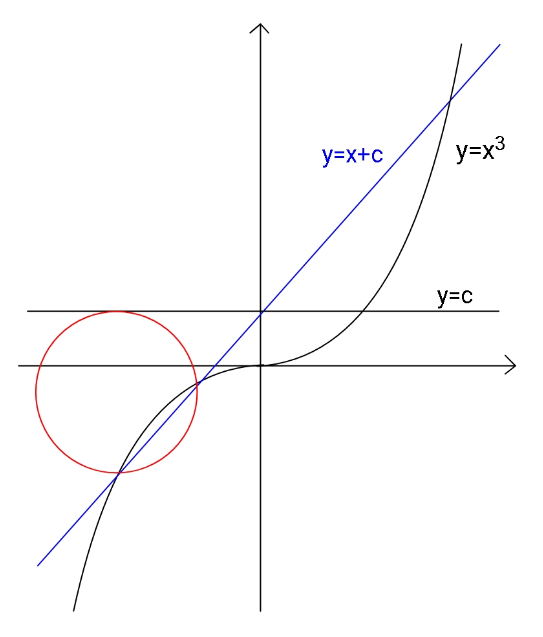
Question Number 117781 Answers: 1 Comments: 0

Log (cosβ) = p ⇒ cos β = 10^p ∴ secβ = (1/(cosβ)) = (1/(10^p )) = 10^(−p) ∴ Log (secβ) = Log 10^(−p) = −p Log 10 = −p

$$\:\:\:\:\:\:\:\:\:\:\:\mathrm{Log}\:\left(\mathrm{cos}\beta\right)\:=\:\mathrm{p}\:\:\:\:\:\Rightarrow\:\:\:\mathrm{cos}\:\beta\:=\:\mathrm{10}^{\mathrm{p}} \:\: \\ $$$$\:\:\:\:\:\:\:\therefore\:\:\:\mathrm{sec}\beta\:=\:\:\frac{\mathrm{1}}{\mathrm{cos}\beta}\:\:=\:\:\frac{\mathrm{1}}{\mathrm{10}^{\mathrm{p}} }\:\:=\:\mathrm{10}^{−\mathrm{p}} \\ $$$$\:\:\:\:\:\:\:\therefore\:\:\:\mathrm{Log}\:\left(\mathrm{sec}\beta\right)\:=\:\:\mathrm{Log}\:\mathrm{10}^{−\mathrm{p}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:−\mathrm{p}\:\mathrm{Log}\:\mathrm{10} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:−\mathrm{p} \\ $$

Question Number 117767 Answers: 1 Comments: 1

x^2 +y_ ^2 =a^2 (√(2 )) x^2 +y^2 =a^2 what is intersection Angle=?

$${x}^{\mathrm{2}} +{y}_{} ^{\mathrm{2}} ={a}^{\mathrm{2}} \sqrt{\mathrm{2}\:} \\ $$$${x}^{\mathrm{2}} +{y}^{\mathrm{2}} ={a}^{\mathrm{2}} \:\:\:\:\:\: \\ $$$$ \\ $$$${what}\:{is}\:{intersection}\:\:{Angle}=?\: \\ $$

Question Number 117666 Answers: 0 Comments: 3

Question Number 117649 Answers: 1 Comments: 0

Let P(x) be a polynomial function of degree n such that P(k)=(k/(k+1)) for k=0,1,2,...,n. Then P(n+1) is equal to (A) −1 if n is even (B) 1 if n is odd (C) (n/(n+2)) if n is even (D) (n/(n+2)) if n is odd Which among the four proposals is/are correct ?

$$\mathrm{Let}\:\mathrm{P}\left({x}\right)\:\mathrm{be}\:\mathrm{a}\:\mathrm{polynomial}\:\mathrm{function}\:\mathrm{of}\:\mathrm{degree}\:\mathrm{n}\:\mathrm{such}\:\mathrm{that} \\ $$$$\mathrm{P}\left({k}\right)=\frac{{k}}{{k}+\mathrm{1}}\:\:\mathrm{for}\:{k}=\mathrm{0},\mathrm{1},\mathrm{2},...,\mathrm{n}.\:\mathrm{Then}\:\mathrm{P}\left(\mathrm{n}+\mathrm{1}\right)\:\mathrm{is}\:\mathrm{equal}\:\mathrm{to}\: \\ $$$$\left(\mathrm{A}\right)\:−\mathrm{1}\:\mathrm{if}\:\mathrm{n}\:\mathrm{is}\:\mathrm{even}\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{B}\right)\:\mathrm{1}\:\mathrm{if}\:{n}\:\mathrm{is}\:\mathrm{odd} \\ $$$$\left(\mathrm{C}\right)\:\frac{{n}}{{n}+\mathrm{2}}\:\mathrm{if}\:{n}\:\mathrm{is}\:\mathrm{even}\:\:\:\:\:\:\:\:\:\:\left(\mathrm{D}\right)\:\frac{{n}}{{n}+\mathrm{2}}\:\:\mathrm{if}\:{n}\:\mathrm{is}\:\mathrm{odd} \\ $$$$\mathrm{Which}\:\mathrm{among}\:\mathrm{the}\:\mathrm{four}\:\mathrm{proposals}\:\mathrm{is}/\mathrm{are}\:\mathrm{correct}\:? \\ $$

Question Number 117603 Answers: 0 Comments: 0

Let f : R→R be a function satisfying the following : (a) f(−x)=−f(x) (b) f(x+1)=f(x)+1 (c) f((1/x))=((f(x))/x^2 ) for all x≠0 Show that (i)f(x)=x for all x,y∈R (ii) f(x+y)=f(x)+f(y) for all x,y∈R (iii) f(xy)=f(x)f(y) for all x,y∈R (iv) f((x/y))=((f(x))/(f(y))) for all x,y∈R with y≠0

$$\mathrm{Let}\:\mathrm{f}\::\:\mathbb{R}\rightarrow\mathbb{R}\:\mathrm{be}\:\mathrm{a}\:\mathrm{function}\:\mathrm{satisfying}\:\mathrm{the}\:\mathrm{following}\:: \\ $$$$\left(\mathrm{a}\right)\:{f}\left(−{x}\right)=−{f}\left({x}\right) \\ $$$$\left(\mathrm{b}\right)\:{f}\left({x}+\mathrm{1}\right)={f}\left({x}\right)+\mathrm{1} \\ $$$$\left(\mathrm{c}\right)\:{f}\left(\frac{\mathrm{1}}{{x}}\right)=\frac{{f}\left({x}\right)}{{x}^{\mathrm{2}} }\:\mathrm{for}\:\mathrm{all}\:{x}\neq\mathrm{0} \\ $$$$\mathrm{Show}\:\mathrm{that} \\ $$$$\left(\mathrm{i}\right){f}\left({x}\right)={x}\:\mathrm{for}\:\mathrm{all}\:{x},\mathrm{y}\in\mathbb{R} \\ $$$$\left(\mathrm{ii}\right)\:{f}\left({x}+{y}\right)={f}\left({x}\right)+{f}\left(\mathrm{y}\right)\:\mathrm{for}\:\mathrm{all}\:{x},\mathrm{y}\in\mathbb{R} \\ $$$$\left(\mathrm{iii}\right)\:{f}\left({xy}\right)={f}\left({x}\right){f}\left(\mathrm{y}\right)\:\mathrm{for}\:\mathrm{all}\:{x},\mathrm{y}\in\mathbb{R} \\ $$$$\left(\mathrm{iv}\right)\:{f}\left(\frac{{x}}{\mathrm{y}}\right)=\frac{{f}\left({x}\right)}{{f}\left(\mathrm{y}\right)}\:\mathrm{for}\:\mathrm{all}\:{x},\mathrm{y}\in\mathbb{R}\:\mathrm{with}\:\mathrm{y}\neq\mathrm{0} \\ $$

Question Number 117555 Answers: 2 Comments: 0

Alternative forms { (((√x)+(√y)=((23)/(12)))),((9x+16y=29)) :}

$${Alternative}\:{forms} \\ $$$$\begin{cases}{\sqrt{{x}}+\sqrt{{y}}=\frac{\mathrm{23}}{\mathrm{12}}}\\{\mathrm{9}{x}+\mathrm{16}{y}=\mathrm{29}}\end{cases} \\ $$$$ \\ $$

Question Number 117172 Answers: 1 Comments: 0

Pg 235 Pg 236 Pg 237 Pg 238 Pg 239 Pg 240 Pg 241 Pg 242 Pg 243 Pg 244

Contact: info@tinkutara.com