
AlgebraQuestion and Answers: Page 238
Question Number 118907 Answers: 0 Comments: 0
Question Number 118896 Answers: 1 Comments: 0

Question Number 118849 Answers: 1 Comments: 2
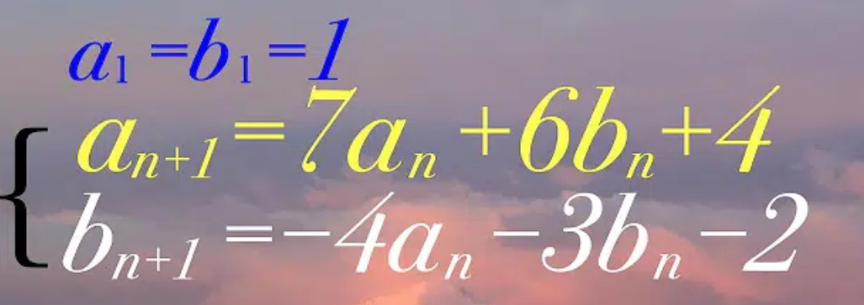
Question Number 118790 Answers: 0 Comments: 0
Question Number 118780 Answers: 2 Comments: 0
Question Number 118777 Answers: 1 Comments: 0
Question Number 118740 Answers: 1 Comments: 1
Question Number 118712 Answers: 4 Comments: 0
Question Number 118651 Answers: 1 Comments: 2

Question Number 118636 Answers: 1 Comments: 2
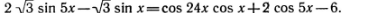
Question Number 118634 Answers: 0 Comments: 3
Question Number 118482 Answers: 1 Comments: 0
Question Number 118452 Answers: 2 Comments: 3
Question Number 118435 Answers: 2 Comments: 0
Question Number 118391 Answers: 2 Comments: 0
Question Number 118371 Answers: 0 Comments: 1
Question Number 118346 Answers: 0 Comments: 0
Question Number 118322 Answers: 2 Comments: 2
Question Number 118298 Answers: 0 Comments: 1
Question Number 118286 Answers: 2 Comments: 0
Question Number 118280 Answers: 0 Comments: 1
Question Number 118277 Answers: 0 Comments: 0
Question Number 118275 Answers: 1 Comments: 1

Question Number 118263 Answers: 0 Comments: 0

Question Number 118227 Answers: 1 Comments: 0
Question Number 118196 Answers: 1 Comments: 0
Pg 233 Pg 234 Pg 235 Pg 236 Pg 237 Pg 238 Pg 239 Pg 240 Pg 241 Pg 242
