Question and Answers Forum

AlgebraQuestion and Answers: Page 235

Question Number 121957 Answers: 0 Comments: 4

resoudre equation∣−2x−(√(3/))4∣=5−2(√7)

$${resoudre}\:{equation}\mid−\mathrm{2}{x}−\sqrt{\mathrm{3}/}\mathrm{4}\mid=\mathrm{5}−\mathrm{2}\sqrt{\mathrm{7}} \\ $$

Question Number 121950 Answers: 1 Comments: 0

Calculate the sum Σ_(k = 1) ^n (1/( (√(k+(√(k^2 +1)))))) .

$$\mathrm{Calculate}\:\mathrm{the}\:\mathrm{sum}\:\underset{\mathrm{k}\:=\:\mathrm{1}} {\overset{\mathrm{n}} {\sum}}\frac{\mathrm{1}}{\:\sqrt{\mathrm{k}+\sqrt{\mathrm{k}^{\mathrm{2}} +\mathrm{1}}}}\:. \\ $$

Question Number 121948 Answers: 0 Comments: 0

Question Number 121919 Answers: 2 Comments: 0

Σ_(k=p) ^∞ 4∙3^(2−k) = (2/9) p = ?

$$\: \\ $$$$\: \\ $$$$\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\underset{{k}={p}} {\overset{\infty} {\sum}}\:\mathrm{4}\centerdot\mathrm{3}^{\mathrm{2}−{k}} \:=\:\frac{\mathrm{2}}{\mathrm{9}} \\ $$$$\:\:\:\:\:\:\:\:\:\:{p}\:=\:? \\ $$$$\: \\ $$$$\: \\ $$

Question Number 121918 Answers: 1 Comments: 6

a, b and c are solutions of x^3 −4x^2 −5x+8=0. Without determinating a, b and c ; calculate a+b+c.

$${a},\:{b}\:{and}\:{c}\:{are}\:{solutions}\:{of}\:{x}^{\mathrm{3}} −\mathrm{4}{x}^{\mathrm{2}} −\mathrm{5}{x}+\mathrm{8}=\mathrm{0}. \\ $$$${Without}\:{determinating}\:{a},\:{b}\:{and}\:{c}\:;\: \\ $$$${calculate}\:{a}+{b}+{c}. \\ $$

Question Number 121913 Answers: 1 Comments: 0

{ ((x^2 +y=36)),((x+y^2 =25 x=? y=?)) :}

$$\begin{cases}{{x}^{\mathrm{2}} +{y}=\mathrm{36}}\\{{x}+{y}^{\mathrm{2}} =\mathrm{25}\:\:{x}=?\:{y}=?}\end{cases} \\ $$

Question Number 121890 Answers: 2 Comments: 1

Question Number 121889 Answers: 2 Comments: 0

Question Number 121847 Answers: 0 Comments: 0

Question Number 121754 Answers: 2 Comments: 0

Question Number 121720 Answers: 2 Comments: 0

Does this example work that way. f(x)=x^x f′(x)=x^x ∙(lnx+1)

$${Does}\:{this}\:{example}\:{work}\:{that}\:{way}. \\ $$$${f}\left({x}\right)={x}^{{x}} \:\:\:{f}'\left({x}\right)={x}^{{x}} \centerdot\left({lnx}+\mathrm{1}\right) \\ $$

Question Number 121693 Answers: 2 Comments: 2

Question Number 121684 Answers: 0 Comments: 1

θ ∈ [0;2π]. solve in C this equation: z^2 −(2^(θ+1) cosθ)z+2^(2θ) =0

$$\theta\:\in\:\left[\mathrm{0};\mathrm{2}\pi\right]. \\ $$$${solve}\:{in}\:\mathbb{C}\:{this}\:{equation}: \\ $$$${z}^{\mathrm{2}} −\left(\mathrm{2}^{\theta+\mathrm{1}} {cos}\theta\right){z}+\mathrm{2}^{\mathrm{2}\theta} =\mathrm{0} \\ $$$$ \\ $$

Question Number 121657 Answers: 0 Comments: 2

Determinate the module and the argument of the complex number z=((1−cosθ+itanθ)/(1+cosθ−isinθ)) with π<θ<2π

$$\mathrm{Determinate}\:\mathrm{the}\:\mathrm{module} \\ $$$$\mathrm{and}\:\mathrm{the}\:\mathrm{argument}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{complex}\:\mathrm{number}\: \\ $$$$\mathrm{z}=\frac{\mathrm{1}−\mathrm{cos}\theta+\mathrm{itan}\theta}{\mathrm{1}+\mathrm{cos}\theta−\mathrm{isin}\theta} \\ $$$$\mathrm{with}\:\pi<\theta<\mathrm{2}\pi \\ $$$$ \\ $$

Question Number 121631 Answers: 0 Comments: 1

Question Number 121590 Answers: 1 Comments: 0

x^2 +2x=8(√6) 2x−((12)/x)=?

$$\boldsymbol{{x}}^{\mathrm{2}} +\mathrm{2}\boldsymbol{{x}}=\mathrm{8}\sqrt{\mathrm{6}} \\ $$$$\mathrm{2}\boldsymbol{{x}}−\frac{\mathrm{12}}{\boldsymbol{{x}}}=? \\ $$

Question Number 121552 Answers: 0 Comments: 5

Question Number 121538 Answers: 1 Comments: 0

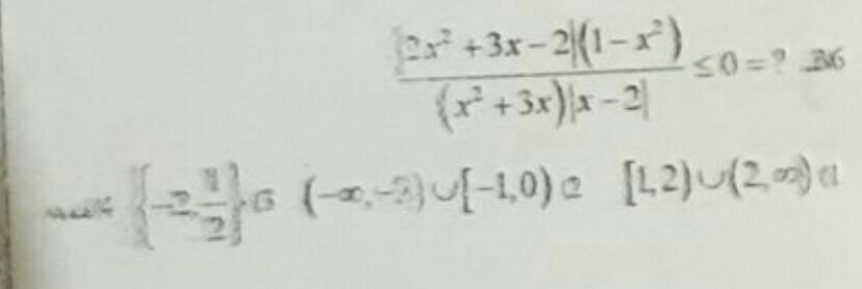
Question Number 121449 Answers: 3 Comments: 1

{ ((x+y=3)),((x^5 +y^5 =33)) :}

$$\:\:\begin{cases}{\mathrm{x}+\mathrm{y}=\mathrm{3}}\\{\mathrm{x}^{\mathrm{5}} +\mathrm{y}^{\mathrm{5}} =\mathrm{33}}\end{cases} \\ $$

Question Number 121446 Answers: 3 Comments: 0

Question Number 121444 Answers: 0 Comments: 3

6^x^2 +81.4^x ≤ 4^x .3^x^2 + 81.2^x^2

$$\:\mathrm{6}^{\mathrm{x}^{\mathrm{2}} } +\mathrm{81}.\mathrm{4}^{\mathrm{x}} \:\leqslant\:\mathrm{4}^{\mathrm{x}} .\mathrm{3}^{\mathrm{x}^{\mathrm{2}} } +\:\mathrm{81}.\mathrm{2}^{\mathrm{x}^{\mathrm{2}} } \\ $$

Question Number 121442 Answers: 2 Comments: 0

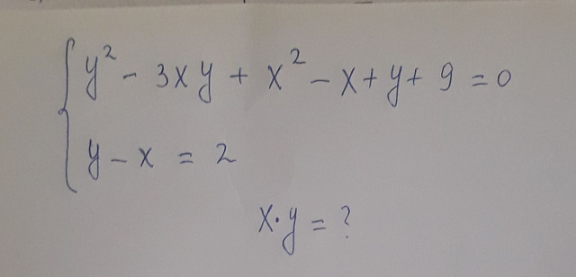
Question Number 121429 Answers: 0 Comments: 1

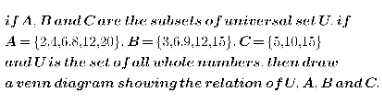
Question Number 121423 Answers: 2 Comments: 0

x^4 −2(√2)x^2 −x+2−(√2)=0 x=?

$${x}^{\mathrm{4}} −\mathrm{2}\sqrt{\mathrm{2}}{x}^{\mathrm{2}} −{x}+\mathrm{2}−\sqrt{\mathrm{2}}=\mathrm{0}\:\:\:{x}=? \\ $$

Question Number 121359 Answers: 0 Comments: 0

Find all n∈N such that (n+3)^n = Σ_(k=3) ^(n+2) k^n

$$\mathrm{Find}\:\mathrm{all}\:\mathrm{n}\in\mathbb{N}\:\mathrm{such}\:\mathrm{that}\:\left(\mathrm{n}+\mathrm{3}\right)^{\mathrm{n}} \:=\:\underset{\mathrm{k}=\mathrm{3}} {\overset{\mathrm{n}+\mathrm{2}} {\sum}}\:\mathrm{k}^{\mathrm{n}} \\ $$

Question Number 121257 Answers: 2 Comments: 4

If today is June 17,2009 and George was born on November 25, 1967. How old is George?

$$\mathrm{If}\:\mathrm{today}\:\mathrm{is}\:\mathrm{June}\:\mathrm{17},\mathrm{2009}\:\mathrm{and}\:\mathrm{George} \\ $$$$\mathrm{was}\:\mathrm{born}\:\mathrm{on}\:\mathrm{November}\:\mathrm{25},\:\mathrm{1967}.\: \\ $$$$\mathrm{How}\:\mathrm{old}\:\mathrm{is}\:\mathrm{George}? \\ $$

Pg 230 Pg 231 Pg 232 Pg 233 Pg 234 Pg 235 Pg 236 Pg 237 Pg 238 Pg 239

Contact: info@tinkutara.com