Question and Answers Forum

AlgebraQuestion and Answers: Page 233

Question Number 123746 Answers: 0 Comments: 1

Question Number 123638 Answers: 0 Comments: 1

show that if x∈R then x and −x cannot be positive

$$\mathrm{show}\:\mathrm{that}\:\mathrm{if}\:\mathrm{x}\in\mathbb{R}\:\mathrm{then}\:\mathrm{x}\:\mathrm{and}\:−\mathrm{x}\:\mathrm{cannot}\:\mathrm{be}\:\mathrm{positive} \\ $$

Question Number 123626 Answers: 0 Comments: 0

Question Number 123595 Answers: 0 Comments: 1

For real numbers (a/b/c) define s_n =a^n +b^n +c^n Suppose s_1 =2 s_2 =6 and s_(3 ) =14.Prove that ∣s_n ^2 −s_(n−1) s_(n+1) ∣=8=holds ∀ n>1

$${For}\:{real}\:{numbers}\:\left({a}/{b}/{c}\right)\:{define}\:{s}_{{n}} ={a}^{{n}} +{b}^{{n}} +{c}^{{n}} \\ $$$${Suppose}\:{s}_{\mathrm{1}} =\mathrm{2}\:{s}_{\mathrm{2}} =\mathrm{6}\:{and}\:{s}_{\mathrm{3}\:} =\mathrm{14}.{Prove}\:{that}\: \\ $$$$\mid{s}_{{n}} ^{\mathrm{2}} −{s}_{{n}−\mathrm{1}} {s}_{{n}+\mathrm{1}} \mid=\mathrm{8}={holds}\:\forall\:{n}>\mathrm{1} \\ $$

Question Number 123591 Answers: 0 Comments: 2

Question Number 123576 Answers: 1 Comments: 2

Question Number 123574 Answers: 1 Comments: 0

Question Number 123548 Answers: 1 Comments: 1

a man 6 ft tall walks at a rate of 7 ft/sec away from a lamppost that is 14 ft hight. at what rate is the lenght of his shadow charging when he is 30 ft away from the lamppost

$${a}\:{man}\:\mathrm{6}\:{ft}\:{tall}\:{walks}\:{at}\:{a}\:{rate} \\ $$$${of}\:\mathrm{7}\:{ft}/{sec}\:{away}\:{from}\:{a}\:{lamppost} \\ $$$${that}\:{is}\:\mathrm{14}\:{ft}\:{hight}. \\ $$$${at}\:{what}\:{rate}\:{is}\:{the}\:{lenght}\:{of}\:{his} \\ $$$${shadow}\:{charging}\:{when}\:{he}\:{is}\:\mathrm{30}\:{ft}\: \\ $$$${away}\:{from}\:{the}\:{lamppost} \\ $$

Question Number 123510 Answers: 0 Comments: 0

sorry −(π/2)

$${sorry}\:−\frac{\pi}{\mathrm{2}} \\ $$

Question Number 123509 Answers: 1 Comments: 0

∫_(−(π/)2) ^(π/2) ∣cosx∣=?

$$\underset{−\frac{\pi}{}\mathrm{2}} {\overset{\frac{\pi}{\mathrm{2}}} {\int}}\mid{cosx}\mid=? \\ $$

Question Number 123426 Answers: 0 Comments: 5

find the radii of all circles with center C tangenting the curve h C= ((5),(1) ) h: x^2 −y^2 =1

$$\mathrm{find}\:\mathrm{the}\:\mathrm{radii}\:\mathrm{of}\:\mathrm{all}\:\mathrm{circles}\:\mathrm{with}\:\mathrm{center}\:{C} \\ $$$$\mathrm{tangenting}\:\mathrm{the}\:\mathrm{curve}\:{h} \\ $$$${C}=\begin{pmatrix}{\mathrm{5}}\\{\mathrm{1}}\end{pmatrix} \\ $$$${h}:\:{x}^{\mathrm{2}} −{y}^{\mathrm{2}} =\mathrm{1} \\ $$

Question Number 123409 Answers: 1 Comments: 0

x^4 +12x−5=0 show that the sum of two roots of the equation is 2

$${x}^{\mathrm{4}} +\mathrm{12}{x}−\mathrm{5}=\mathrm{0}\:\:\:{show}\:{that}\:{the}\:{sum}\:{of}\:{two}\:{roots} \\ $$$${of}\:{the}\:{equation}\:{is}\:\mathrm{2} \\ $$

Question Number 123362 Answers: 1 Comments: 0

Question Number 123265 Answers: 2 Comments: 2

Question Number 123175 Answers: 1 Comments: 0

Show by recurrence that ∀ n ∈ N, Σ_(k=0 ) ^(n−1) q^k =((q^n −1)/(q−1))

$${Show}\:{by}\:{recurrence}\:{that} \\ $$$$\forall\:{n}\:\in\:\mathbb{N},\:\sum_{{k}=\mathrm{0}\:} ^{{n}−\mathrm{1}} {q}^{{k}} =\frac{{q}^{{n}} −\mathrm{1}}{{q}−\mathrm{1}}\: \\ $$

Question Number 123114 Answers: 2 Comments: 2

Is there any solution(s)?? { ((36x^2 y−27y^3 =8)),((4x^3 −27xy^2 =4)) :} please....

$${Is}\:{there}\:{any}\:{solution}\left({s}\right)?? \\ $$$$\begin{cases}{\mathrm{36}{x}^{\mathrm{2}} {y}−\mathrm{27}{y}^{\mathrm{3}} =\mathrm{8}}\\{\mathrm{4}{x}^{\mathrm{3}} −\mathrm{27}{xy}^{\mathrm{2}} =\mathrm{4}}\end{cases} \\ $$$${please}.... \\ $$

Question Number 123108 Answers: 0 Comments: 2

Question Number 123053 Answers: 0 Comments: 0

Find all P(x) with real coefficients such that P(x^2 +x+1) divides P(x^3 −1)

$${Find}\:{all}\:{P}\left({x}\right)\:{with}\:{real}\:{coefficients}\:{such}\:{that}\: \\ $$$${P}\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)\:{divides}\:{P}\left({x}^{\mathrm{3}} −\mathrm{1}\right) \\ $$

Question Number 123039 Answers: 1 Comments: 0

{ ((3^x +9^(2y) =27)),((2^x +4^(−y) =2^(−3) )) :} x=? y=?

$$\begin{cases}{\mathrm{3}^{{x}} +\mathrm{9}^{\mathrm{2}{y}} =\mathrm{27}}\\{\mathrm{2}^{{x}} +\mathrm{4}^{−{y}} =\mathrm{2}^{−\mathrm{3}} }\end{cases} \\ $$$${x}=?\:{y}=? \\ $$

Question Number 123062 Answers: 1 Comments: 0

Erica′s age is 8 years and her mother is 42. In how many years time will the mother be 3 times as old as her daughter?

$$\mathrm{Erica}'\mathrm{s}\:\mathrm{age}\:\mathrm{is}\:\mathrm{8}\:\mathrm{years}\:\mathrm{and}\:\mathrm{her}\:\mathrm{mother}\:\mathrm{is}\:\mathrm{42}. \\ $$$$\mathrm{In}\:\mathrm{how}\:\mathrm{many}\:\mathrm{years}\:\mathrm{time}\:\mathrm{will}\:\mathrm{the}\:\mathrm{mother}\:\mathrm{be} \\ $$$$\mathrm{3}\:\mathrm{times}\:\mathrm{as}\:\mathrm{old}\:\mathrm{as}\:\mathrm{her}\:\mathrm{daughter}? \\ $$

Question Number 123025 Answers: 1 Comments: 4

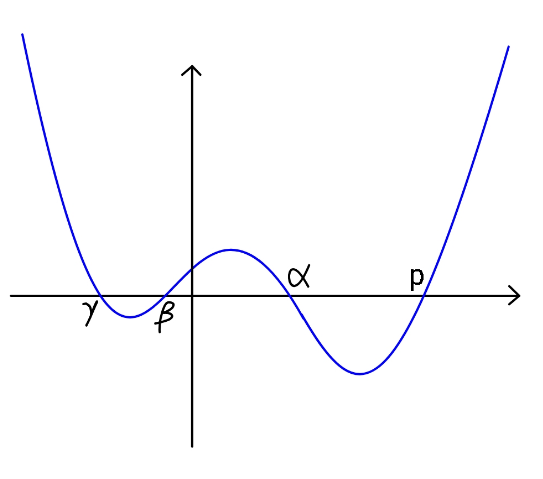
Question Number 123024 Answers: 1 Comments: 0

Question Number 122957 Answers: 1 Comments: 1

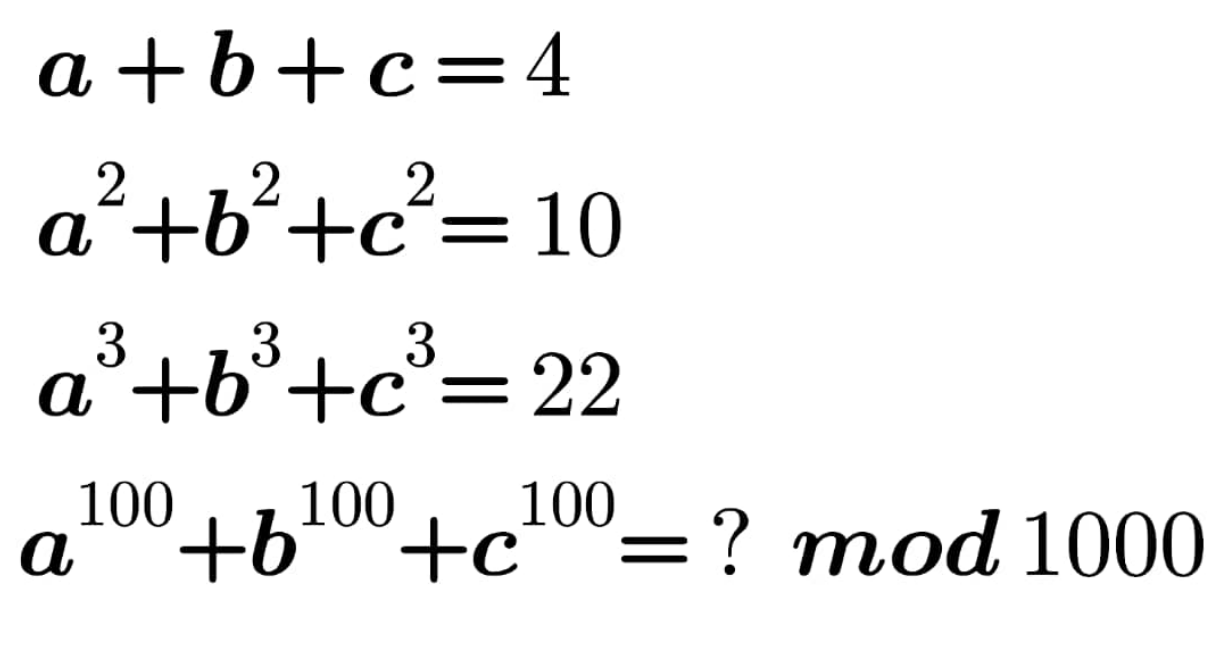
Question Number 122950 Answers: 0 Comments: 1

(√(1+(√(1+2(√(3+...(√(1+2014+(√(1+2015.2016)))))))))) = ?

$$\:\:\sqrt{\mathrm{1}+\sqrt{\mathrm{1}+\mathrm{2}\sqrt{\mathrm{3}+...\sqrt{\mathrm{1}+\mathrm{2014}+\sqrt{\mathrm{1}+\mathrm{2015}.\mathrm{2016}}}}}}\:=\:? \\ $$

Question Number 122897 Answers: 2 Comments: 0

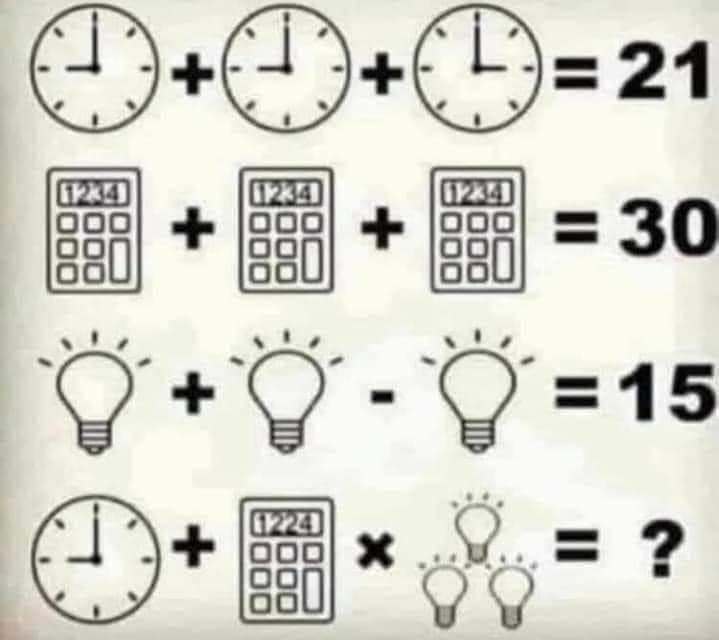
Question Number 122883 Answers: 4 Comments: 2

Σ_1 ^∞ (((sin (x))/x))=?

$$\sum_{\mathrm{1}} ^{\infty} \left(\frac{\mathrm{sin}\:\left(\mathrm{x}\right)}{\mathrm{x}}\right)=? \\ $$

Pg 228 Pg 229 Pg 230 Pg 231 Pg 232 Pg 233 Pg 234 Pg 235 Pg 236 Pg 237

Contact: info@tinkutara.com