Question and Answers Forum

AlgebraQuestion and Answers: Page 230

Question Number 125132 Answers: 0 Comments: 0

Find the number of real roots of ax^7 −4x^4 +x^2 +1=0 where a>2

$${Find}\:{the}\:{number}\:{of}\:{real}\:{roots}\:{of}\:{ax}^{\mathrm{7}} −\mathrm{4}{x}^{\mathrm{4}} +{x}^{\mathrm{2}} +\mathrm{1}=\mathrm{0} \\ $$$${where}\:{a}>\mathrm{2} \\ $$

Question Number 125131 Answers: 0 Comments: 0

Let a,b,c∈ complex numbers such that the roots of the equation ax^2 +bx+c=0 have same modulus Prove that a=0 iff b=0

$${Let}\:{a},{b},{c}\in\:{complex}\:{numbers}\:{such}\:{that}\:{the}\:{roots} \\ $$$${of}\:{the}\:{equation}\:{ax}^{\mathrm{2}} +{bx}+{c}=\mathrm{0}\:{have}\:{same}\:{modulus} \\ $$$${Prove}\:{that}\:{a}=\mathrm{0}\:{iff}\:{b}=\mathrm{0} \\ $$

Question Number 125107 Answers: 0 Comments: 1

Question Number 124988 Answers: 1 Comments: 3

Question Number 124963 Answers: 2 Comments: 1

∫_0 ^( (π/4)) ((sin(ς)dς)/(cos(ς)+sin(ς)))dς where ς : zeta

$$\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{4}}} \frac{\mathrm{sin}\left(\varsigma\right)\mathrm{d}\varsigma}{\mathrm{cos}\left(\varsigma\right)+\mathrm{sin}\left(\varsigma\right)}\mathrm{d}\varsigma \\ $$$$\mathrm{where}\:\varsigma\::\:\mathrm{zeta}\: \\ $$

Question Number 124907 Answers: 0 Comments: 0

Question Number 125185 Answers: 1 Comments: 0

{ (((√x) + y = 11)),((x + (√y) = 7 )) :}

$$\:\begin{cases}{\sqrt{{x}}\:+\:{y}\:=\:\mathrm{11}}\\{{x}\:+\:\sqrt{{y}}\:=\:\mathrm{7}\:}\end{cases} \\ $$

Question Number 124820 Answers: 1 Comments: 0

Question Number 124797 Answers: 2 Comments: 0

Question Number 124791 Answers: 1 Comments: 1

Question Number 124740 Answers: 1 Comments: 1

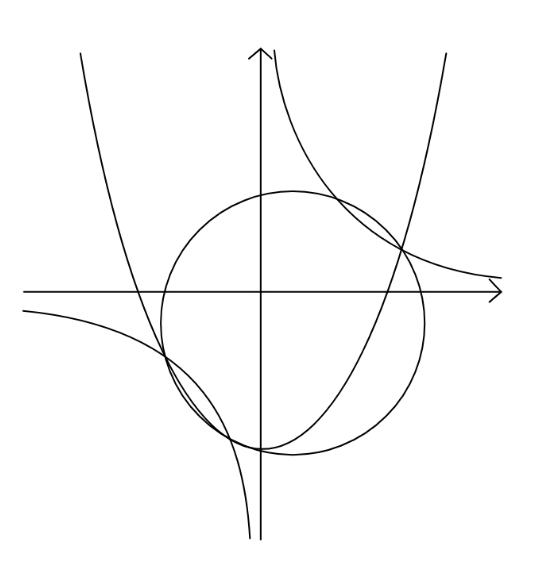
Question Number 124617 Answers: 0 Comments: 0

Question Number 124567 Answers: 1 Comments: 0

find the smallest integer which has 28 divisors and is divisible by 28.

$${find}\:{the}\:{smallest}\:{integer}\:{which}\:{has} \\ $$$$\mathrm{28}\:{divisors}\:{and}\:{is}\:{divisible}\:{by}\:\mathrm{28}. \\ $$

Question Number 124522 Answers: 1 Comments: 0

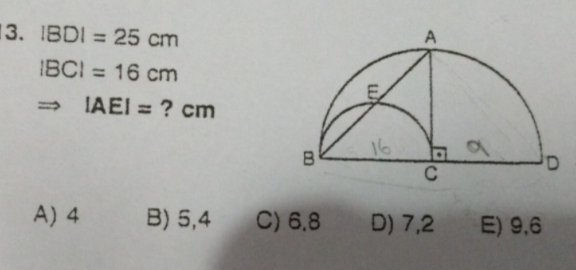
Question Number 124496 Answers: 0 Comments: 0

show that between 2 real numbers ∃ x,y s.t x<0 and y>0

$${show}\:{that}\:{between}\:\mathrm{2}\:{real}\:{numbers}\:\exists\:{x},{y}\:{s}.{t}\:{x}<\mathrm{0}\:{and}\:{y}>\mathrm{0} \\ $$

Question Number 124458 Answers: 0 Comments: 0

Question Number 124387 Answers: 1 Comments: 0

Solve x^2 −(1/2)x−7=x−3(√(2x^2 −3x+2)) for xεR .

$$\:{Solve}\:{x}^{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{2}}{x}−\mathrm{7}={x}−\mathrm{3}\sqrt{\mathrm{2}{x}^{\mathrm{2}} −\mathrm{3}{x}+\mathrm{2}} \\ $$$${for}\:{x}\epsilon{R}\:. \\ $$

Question Number 124375 Answers: 0 Comments: 0

Question Number 124314 Answers: 0 Comments: 1

{ ((x(√x) +y(√y) = 19)),((x(√y) +y(√x) = 15)) :}. find x+y { ((x(√x) +y(√y) = 133)),((y(√x) − x(√y) = 30)) :}. find x+y+xy { (((√x) +y = 53)),((x + (√y) = 23)) :}. find x+y

$$\begin{cases}{{x}\sqrt{{x}}\:+{y}\sqrt{{y}}\:=\:\mathrm{19}}\\{{x}\sqrt{{y}}\:+{y}\sqrt{{x}}\:=\:\mathrm{15}}\end{cases}.\:{find}\:{x}+{y} \\ $$$$\:\begin{cases}{{x}\sqrt{{x}}\:+{y}\sqrt{{y}}\:=\:\mathrm{133}}\\{{y}\sqrt{{x}}\:−\:{x}\sqrt{{y}}\:=\:\mathrm{30}}\end{cases}.\:{find}\:{x}+{y}+{xy} \\ $$$$\:\begin{cases}{\sqrt{{x}}\:+{y}\:=\:\mathrm{53}}\\{{x}\:+\:\sqrt{{y}}\:=\:\mathrm{23}}\end{cases}.\:{find}\:{x}+{y} \\ $$

Question Number 124312 Answers: 1 Comments: 0

3^x + 3^(−x) = 3 −(x−3)^2 x =?

$$\:\:\:\:\mathrm{3}^{{x}} \:+\:\mathrm{3}^{−{x}} \:=\:\mathrm{3}\:−\left({x}−\mathrm{3}\right)^{\mathrm{2}} \\ $$$$\:{x}\:=?\: \\ $$

Question Number 124240 Answers: 0 Comments: 0

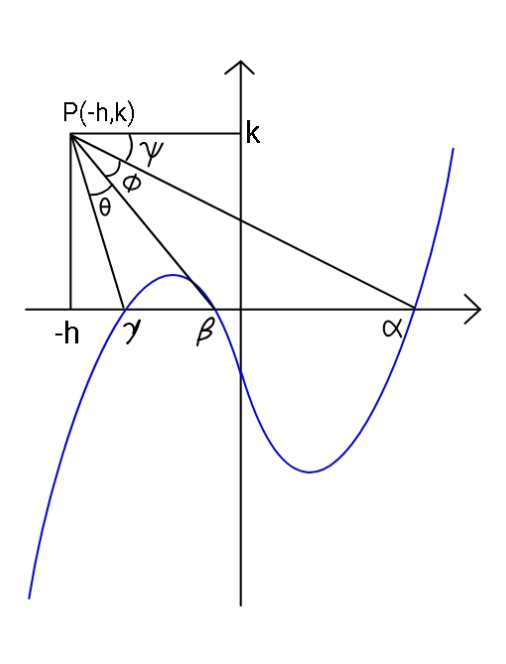
Question Number 124210 Answers: 2 Comments: 0

If x + (√x) = 2020 then x + ((2020)/( (√x))) = ?

$$\:{If}\:{x}\:+\:\sqrt{{x}}\:=\:\mathrm{2020}\: \\ $$$${then}\:{x}\:+\:\frac{\mathrm{2020}}{\:\sqrt{{x}}}\:=\:? \\ $$

Question Number 124149 Answers: 1 Comments: 4

Two men and four women line up at a checkout counter in a store. (a) In how many ways can they line up??? (b) In how many ways can they line up if the first person line is a woman and the line changes by gender. (w, m, w, w, m, w)??

$$\mathrm{Two}\:\mathrm{men}\:\mathrm{and}\:\mathrm{four}\:\mathrm{women}\:\mathrm{line}\:\mathrm{up}\:\mathrm{at}\:\mathrm{a}\:\mathrm{checkout}\:\mathrm{counter}\:\mathrm{in}\:\mathrm{a}\:\mathrm{store}. \\ $$$$\left(\mathrm{a}\right)\:\:\:\:\mathrm{In}\:\mathrm{how}\:\mathrm{many}\:\mathrm{ways}\:\mathrm{can}\:\mathrm{they}\:\mathrm{line}\:\mathrm{up}??? \\ $$$$\left(\mathrm{b}\right)\:\:\:\:\mathrm{In}\:\mathrm{how}\:\mathrm{many}\:\mathrm{ways}\:\mathrm{can}\:\mathrm{they}\:\mathrm{line}\:\mathrm{up}\:\mathrm{if}\:\mathrm{the}\:\mathrm{first}\:\mathrm{person}\:\mathrm{line}\:\mathrm{is} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\mathrm{a}\:\mathrm{woman}\:\mathrm{and}\:\mathrm{the}\:\mathrm{line}\:\mathrm{changes}\:\mathrm{by}\:\mathrm{gender}.\:\left(\mathrm{w},\:\:\mathrm{m},\:\:\mathrm{w},\:\:\mathrm{w},\:\:\mathrm{m},\:\:\mathrm{w}\right)?? \\ $$

Question Number 124110 Answers: 2 Comments: 0

N=(3548)^9 ×(2537)^(31) Determinate the last digit of N.

$$ \\ $$$${N}=\left(\mathrm{3548}\right)^{\mathrm{9}} ×\left(\mathrm{2537}\right)^{\mathrm{31}} \\ $$$${Determinate}\:{the}\:{last}\:{digit}\:{of}\:{N}. \\ $$

Question Number 124109 Answers: 1 Comments: 0

N=x32y in base 5. determinate x and y such that N is divisible by 3 and 4.

$$ \\ $$$$ \\ $$$${N}={x}\mathrm{32}{y}\:{in}\:{base}\:\mathrm{5}. \\ $$$${determinate}\:{x}\:{and}\:{y}\:{such}\:{that}\:{N}\:{is} \\ $$$${divisible}\:{by}\:\mathrm{3}\:{and}\:\mathrm{4}. \\ $$

Question Number 124108 Answers: 1 Comments: 0

N=x43y in base 7. determinate x and y such that N is divisible by 6.

$${N}={x}\mathrm{43}{y}\:{in}\:{base}\:\mathrm{7}. \\ $$$${determinate}\:{x}\:{and}\:{y}\:{such}\:{that}\:{N}\:{is} \\ $$$${divisible}\:{by}\:\mathrm{6}. \\ $$$$ \\ $$

Pg 225 Pg 226 Pg 227 Pg 228 Pg 229 Pg 230 Pg 231 Pg 232 Pg 233 Pg 234

Contact: info@tinkutara.com