Question and Answers Forum

AlgebraQuestion and Answers: Page 229

Question Number 126935 Answers: 0 Comments: 0

Prove or give a counter example: (a+1)^(n−1) =Σ_(k=1) ^n ( _(k−1) ^(n−1) )a^(k−1) ( _r ^n )=((n!)/(r!(n−r)!))

$${Prove}\:{or}\:{give}\:{a}\:{counter}\:{example}: \\ $$$$\left({a}+\mathrm{1}\right)^{{n}−\mathrm{1}} =\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}\left(\underset{{k}−\mathrm{1}} {\overset{{n}−\mathrm{1}} {\:}}\right){a}^{{k}−\mathrm{1}} \\ $$$$\left(\underset{{r}} {\overset{{n}} {\:}}\right)=\frac{{n}!}{{r}!\left({n}−{r}\right)!} \\ $$

Question Number 126931 Answers: 1 Comments: 0

y=(1−x)^(cosx)

$${y}=\left(\mathrm{1}−{x}\right)^{{cosx}} \\ $$

Question Number 126930 Answers: 1 Comments: 4

(√(1997×1996×1995×1994+1)) =?

$$\:\:\sqrt{\mathrm{1997}×\mathrm{1996}×\mathrm{1995}×\mathrm{1994}+\mathrm{1}}\:=? \\ $$

Question Number 126887 Answers: 2 Comments: 2

... calculus... please solve: ( with explanation) {_(x^2 +y=3) ^(x+y^2 =5)

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:...\:{calculus}... \\ $$$${please}\:\:{solve}:\:\left(\:{with}\:{explanation}\right) \\ $$$$\:\:\:\:\left\{_{{x}^{\mathrm{2}} +{y}=\mathrm{3}} ^{{x}+{y}^{\mathrm{2}} =\mathrm{5}} \right. \\ $$$$ \\ $$

Question Number 126882 Answers: 1 Comments: 0

x^x =3

$$\boldsymbol{{x}}^{\boldsymbol{{x}}} =\mathrm{3} \\ $$

Question Number 126845 Answers: 3 Comments: 0

... calculus (I)... prove :: i:: ⌊2x⌋=^? ⌊x⌋+⌊x+(1/2)⌋ ii:: ⌊3x⌋=⌊x⌋+⌊x+(1/3)⌋+⌊x+(2/3)⌋

$$\:\:\:\:\:\:\:\:\:\:\:...\:{calculus}\:\:\left(\mathrm{I}\right)... \\ $$$$\:\:\:\:{prove}\:::\: \\ $$$$\:\:\:\:\:\:\:{i}::\:\:\lfloor\mathrm{2}{x}\rfloor\overset{?} {=}\lfloor{x}\rfloor+\lfloor{x}+\frac{\mathrm{1}}{\mathrm{2}}\rfloor \\ $$$$\:\:\:\:\:\:\:{ii}::\:\lfloor\mathrm{3}{x}\rfloor=\lfloor{x}\rfloor+\lfloor{x}+\frac{\mathrm{1}}{\mathrm{3}}\rfloor+\lfloor{x}+\frac{\mathrm{2}}{\mathrm{3}}\rfloor \\ $$$$ \\ $$

Question Number 126723 Answers: 0 Comments: 2

Question Number 126702 Answers: 1 Comments: 0

if tanh(x/2)=t prove that cosh(x)=((1+t^2 )/(1−t^2 ))

$${if}\:\:\:\:{tanh}\frac{{x}}{\mathrm{2}}={t}\:\:{prove}\:{that}\:\:{cosh}\left({x}\right)=\frac{\mathrm{1}+{t}^{\mathrm{2}} }{\mathrm{1}−{t}^{\mathrm{2}} } \\ $$$$ \\ $$

Question Number 126701 Answers: 2 Comments: 0

use right triangles to explain why cos^(−1) (x)+sin^(−1) (x)=π/2

$${use}\:{right}\:{triangles}\:{to}\:{explain} \\ $$$${why}\:{cos}^{−\mathrm{1}} \left({x}\right)+{sin}^{−\mathrm{1}} \left({x}\right)=\pi/\mathrm{2} \\ $$

Question Number 126700 Answers: 2 Comments: 0

θ=sin^(−1) ((2/5)) find cos(θ) and tan(θ)

$$\theta={sin}^{−\mathrm{1}} \left(\frac{\mathrm{2}}{\mathrm{5}}\right)\:{find}\:{cos}\left(\theta\right)\:{and}\:{tan}\left(\theta\right) \\ $$$$ \\ $$

Question Number 126619 Answers: 2 Comments: 0

5^x + 7^x = (9.8)^x x = ?

$$\: \\ $$$$\:\:\mathrm{5}^{\boldsymbol{\mathrm{x}}} \:+\:\mathrm{7}^{\boldsymbol{\mathrm{x}}} \:=\:\left(\mathrm{9}.\mathrm{8}\right)^{\boldsymbol{\mathrm{x}}} \\ $$$$\: \\ $$$$\:\boldsymbol{\mathrm{x}}\:=\:? \\ $$$$\: \\ $$

Question Number 126504 Answers: 0 Comments: 0

Question Number 126484 Answers: 0 Comments: 6

Question Number 126482 Answers: 1 Comments: 0

Question Number 126430 Answers: 3 Comments: 1

log _6^(15) =a log _(12)^(18) =b find log _(25)^(24) in terms of a and b

$$\mathrm{log}\:_{\mathrm{6}} ^{\mathrm{15}} =\mathrm{a}\:\:\:\mathrm{log}\:_{\mathrm{12}} ^{\mathrm{18}} =\mathrm{b}\:\:\mathrm{find}\:\mathrm{log}\:_{\mathrm{25}} ^{\mathrm{24}} \\ $$$$\mathrm{in}\:\mathrm{terms}\:\mathrm{of}\:\mathrm{a}\:\mathrm{and}\:\mathrm{b} \\ $$

Question Number 126391 Answers: 3 Comments: 0

show that m^2 +m and 2m+1 are prime betwen them.

$$ \\ $$$${show}\:{that}\: \\ $$$${m}^{\mathrm{2}} +{m}\:{and}\:\:\mathrm{2}{m}+\mathrm{1}\:{are}\:{prime}\:{betwen} \\ $$$${them}. \\ $$

Question Number 126363 Answers: 2 Comments: 0

x^3 −x−c=0 ; [c<2/(3(√3))] (Solve by a method other than trigonometric solution).

$${x}^{\mathrm{3}} −{x}−{c}=\mathrm{0}\:\:\:\:\:\:\:;\:\:\:\:\left[{c}<\mathrm{2}/\left(\mathrm{3}\sqrt{\mathrm{3}}\right)\right] \\ $$$$\left({Solve}\:{by}\:{a}\:\:{method}\:{other}\:{than}\right. \\ $$$$\left.{trigonometric}\:{solution}\right). \\ $$

Question Number 126329 Answers: 1 Comments: 0

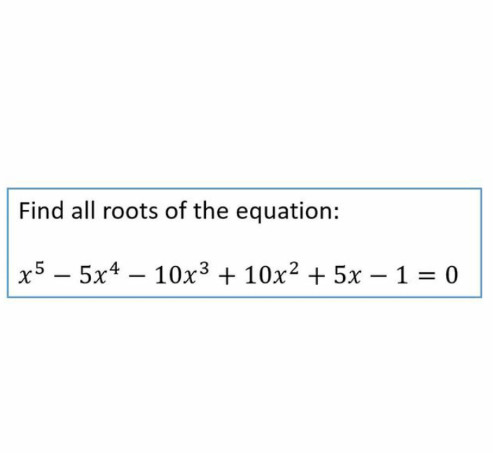
Question Number 126180 Answers: 2 Comments: 0

solve ∣ ∣x−1∣ −2∣ = ∣ x−3 ∣

$${solve}\:\mid\:\mid{x}−\mathrm{1}\mid\:−\mathrm{2}\mid\:=\:\mid\:{x}−\mathrm{3}\:\mid\: \\ $$

Question Number 126165 Answers: 0 Comments: 1

if: b^2 = −3 −c^2 +2(a−b−c) −a^2 calculate: a + b + c = ?

$${if}:\:\:\:{b}^{\mathrm{2}} \:=\:−\mathrm{3}\:−{c}^{\mathrm{2}} \:+\mathrm{2}\left({a}−{b}−{c}\right)\:−{a}^{\mathrm{2}} \\ $$$$ \\ $$$${calculate}:\:{a}\:+\:{b}\:+\:{c}\:=\:? \\ $$

Question Number 126161 Answers: 3 Comments: 0

find “a” ^a (√(a^2 )) = ((4^(−(1/2)) )^(1/2) )^(−2)

$${find}\:\:``{a}'' \\ $$$$ \\ $$$$\:^{{a}} \sqrt{{a}^{\mathrm{2}} \:}\:=\:\left(\left(\mathrm{4}^{−\frac{\mathrm{1}}{\mathrm{2}}} \right)^{\frac{\mathrm{1}}{\mathrm{2}}} \right)^{−\mathrm{2}} \:\:\:\:\: \\ $$

Question Number 126109 Answers: 2 Comments: 0

....nice calculus... verify that :: A=(((2+(√5)))^(1/3) /(1+(√5))) is a rational number ...

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:....{nice}\:{calculus}... \\ $$$$\:\:{verify}\:\:{that}\:::\:\:\mathrm{A}=\frac{\sqrt[{\mathrm{3}}]{\mathrm{2}+\sqrt{\mathrm{5}}}}{\mathrm{1}+\sqrt{\mathrm{5}}}\:{is} \\ $$$$\:\:\:\:\:\:{a}\:\:{rational}\:\:{number}\:... \\ $$

Question Number 126105 Answers: 1 Comments: 1

1≤a;b;c;d≤2 ∣(1−a)(1−b)(1−c)(1−d)∣≤((abcd)/4)

$$\mathrm{1}\leqslant{a};{b};{c};{d}\leqslant\mathrm{2} \\ $$$$\mid\left(\mathrm{1}−{a}\right)\left(\mathrm{1}−{b}\right)\left(\mathrm{1}−{c}\right)\left(\mathrm{1}−{d}\right)\mid\leqslant\frac{{abcd}}{\mathrm{4}} \\ $$

Question Number 126104 Answers: 0 Comments: 1

((a+b)/c) + ((b+c)/a) +((a+c)/b) ≥ 4a−3b+c a≥b≥c

$$\frac{{a}+{b}}{{c}}\:+\:\frac{{b}+{c}}{{a}}\:+\frac{{a}+{c}}{{b}}\:\geqslant\:\mathrm{4}{a}−\mathrm{3}{b}+{c} \\ $$$${a}\geqslant{b}\geqslant{c} \\ $$

Question Number 126030 Answers: 1 Comments: 0

2^x =4x solve it please

$$\mathrm{2}^{\mathrm{x}} =\mathrm{4x} \\ $$$$\mathrm{solve}\:\mathrm{it}\:\mathrm{please} \\ $$

Question Number 125990 Answers: 0 Comments: 0

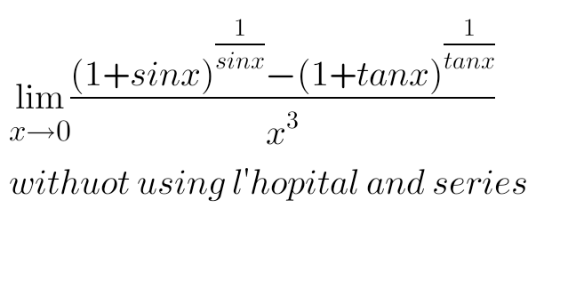
Pg 224 Pg 225 Pg 226 Pg 227 Pg 228 Pg 229 Pg 230 Pg 231 Pg 232 Pg 233

Contact: info@tinkutara.com