Question and Answers Forum

All Questions Topic List

AlgebraQuestion and Answers: Page 227

Question Number 120471 Answers: 1 Comments: 0

solve in Z x^3 +2x+1≡1[4]

$${solve}\:{in}\:\mathbb{Z}\:{x}^{\mathrm{3}} +\mathrm{2}{x}+\mathrm{1}\equiv\mathrm{1}\left[\mathrm{4}\right] \\ $$

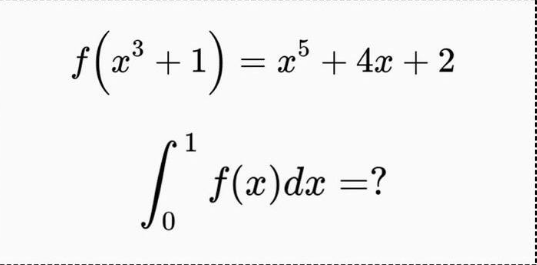
Question Number 120470 Answers: 0 Comments: 0

solve in function of n: 2^n ≡x−4[3] n∈N

$${solve}\:{in}\:{function}\:{of}\:{n}: \\ $$$$\mathrm{2}^{{n}} \equiv{x}−\mathrm{4}\left[\mathrm{3}\right] \\ $$$${n}\in\mathbb{N} \\ $$

Question Number 120469 Answers: 1 Comments: 0

c alculate the rest of the division of 2^n by 3 ; n ∈ N

$${c}\:{alculate}\:{the}\:{rest}\:{of}\:{the}\:{division}\:{of} \\ $$$$\mathrm{2}^{{n}} \:{by}\:\mathrm{3}\:;\:{n}\:\in\:\mathbb{N} \\ $$

Question Number 120464 Answers: 1 Comments: 0

Question Number 120455 Answers: 0 Comments: 0

Question Number 120355 Answers: 3 Comments: 2

Question Number 120325 Answers: 0 Comments: 6

Let f:R→R be a function satisfying the functional relation (f(x))^y +(f(y))^x =2f(xy) for all x, y ∈R and it is given that f(1)=1/2. Answer the following questions. (i) f(x+y)= (A) f(x)+f(y) (B) f(x)f(y) (C) f(x^y y^x ) (D) ((f(x))/(f(y))) (ii) f(xy)= (A) f(x)f(y) (B) f(x)+f(y) (C) (f(x))^y (D) (f(xy))^(xy) (iii) Σ_(k=0) ^∞ f(k)= (A) 5/2 (B) 3/2 (C) 3 (D) 2

$$\mathrm{Let}\:{f}:\mathbb{R}\rightarrow\mathbb{R}\:\mathrm{be}\:\mathrm{a}\:\mathrm{function}\:\mathrm{satisfying}\:\mathrm{the} \\ $$$$\mathrm{functional}\:\mathrm{relation} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left({f}\left(\mathrm{x}\right)\right)^{\mathrm{y}} +\left({f}\left(\mathrm{y}\right)\right)^{\mathrm{x}} =\mathrm{2}{f}\left(\mathrm{xy}\right) \\ $$$$\mathrm{for}\:\mathrm{all}\:\mathrm{x},\:\mathrm{y}\:\in\mathbb{R}\:\mathrm{and}\:\mathrm{it}\:\mathrm{is}\:\mathrm{given}\:\mathrm{that}\:{f}\left(\mathrm{1}\right)=\mathrm{1}/\mathrm{2}.\:\mathrm{Answer} \\ $$$$\mathrm{the}\:\mathrm{following}\:\mathrm{questions}. \\ $$$$\left(\boldsymbol{\mathrm{i}}\right)\:\:\:\:{f}\left(\mathrm{x}+\mathrm{y}\right)= \\ $$$$\:\:\:\:\:\:\:\:\:\left(\mathrm{A}\right)\:{f}\left(\mathrm{x}\right)+{f}\left(\mathrm{y}\right)\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{B}\right)\:\:{f}\left(\mathrm{x}\right){f}\left(\mathrm{y}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\left(\mathrm{C}\right)\:{f}\left(\mathrm{x}^{\mathrm{y}} \mathrm{y}^{\mathrm{x}} \right)\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{D}\right)\:\:\:\frac{{f}\left(\mathrm{x}\right)}{{f}\left(\mathrm{y}\right)} \\ $$$$\left(\boldsymbol{\mathrm{ii}}\right)\:\:\:\:{f}\left(\mathrm{xy}\right)= \\ $$$$\:\:\:\:\:\:\:\:\:\:\left(\mathrm{A}\right)\:{f}\left(\mathrm{x}\right){f}\left(\mathrm{y}\right)\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{B}\right)\:{f}\left(\mathrm{x}\right)+{f}\left(\mathrm{y}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\left(\mathrm{C}\right)\:\left({f}\left(\mathrm{x}\right)\right)^{\mathrm{y}} \:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{D}\right)\:\:\left({f}\left(\mathrm{xy}\right)\right)^{\mathrm{xy}} \\ $$$$\left(\boldsymbol{\mathrm{iii}}\right)\:\:\underset{\mathrm{k}=\mathrm{0}} {\overset{\infty} {\sum}}{f}\left({k}\right)= \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{A}\right)\:\mathrm{5}/\mathrm{2}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{B}\right)\:\mathrm{3}/\mathrm{2}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{C}\right)\:\mathrm{3}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{D}\right)\:\mathrm{2} \\ $$

Question Number 120324 Answers: 1 Comments: 0

we are in C. (E): z^3 +(4−5i)z^2 +(8−20i)z−40i=0 1) Show that (E) has one imaginary pure root 2) solve (E)

$$\mathrm{we}\:\mathrm{are}\:\mathrm{in}\:\mathbb{C}. \\ $$$$\left(\mathrm{E}\right):\:\mathrm{z}^{\mathrm{3}} +\left(\mathrm{4}−\mathrm{5i}\right)\mathrm{z}^{\mathrm{2}} +\left(\mathrm{8}−\mathrm{20i}\right)\mathrm{z}−\mathrm{40i}=\mathrm{0} \\ $$$$\left.\mathrm{1}\right)\:\mathrm{Show}\:\mathrm{that}\:\left(\mathrm{E}\right)\:\mathrm{has}\:\mathrm{one}\:\mathrm{imaginary}\:\mathrm{pure}\:\mathrm{root} \\ $$$$\left.\mathrm{2}\right)\:\mathrm{solve}\:\left(\mathrm{E}\right) \\ $$

Question Number 120240 Answers: 1 Comments: 0

→

$$\rightarrow \\ $$

Question Number 120229 Answers: 2 Comments: 0

Question Number 120228 Answers: 0 Comments: 0

Please solve using special function like LambertW function or any other function (if possibe) ((8/7))^x +17^x =25x

$${Please}\:{solve}\:{using}\:{special}\:{function}\:{like}\:{LambertW} \\ $$$${function}\:{or}\:{any}\:{other}\:{function}\:\left({if}\:{possibe}\right) \\ $$$$\left(\frac{\mathrm{8}}{\mathrm{7}}\right)^{{x}} +\mathrm{17}^{{x}} =\mathrm{25}{x} \\ $$

Question Number 120185 Answers: 1 Comments: 0

solve for x, y∈Z (√x)+(√y)=(√(2020))

$${solve}\:{for}\:{x},\:{y}\in{Z} \\ $$$$\sqrt{{x}}+\sqrt{{y}}=\sqrt{\mathrm{2020}} \\ $$

Question Number 120154 Answers: 1 Comments: 0

solve using LambertW function ((8/7))^x +17=25x

$${solve}\:{using}\:{LambertW}\:{function} \\ $$$$\left(\frac{\mathrm{8}}{\mathrm{7}}\right)^{{x}} +\mathrm{17}=\mathrm{25}{x} \\ $$

Question Number 120152 Answers: 0 Comments: 0

Determinate and construct the set of points M which have as affix z in each case: 1) arg(i−z)=0[π] 2) arg(z+1−i)=(π/6)[2π]

$$\mathrm{Determinate}\:\mathrm{and}\:\mathrm{construct}\:\mathrm{the}\:\mathrm{set}\:\mathrm{of}\:\mathrm{points}\:\mathrm{M} \\ $$$$\mathrm{which}\:\mathrm{have}\:\mathrm{as}\:\mathrm{affix}\:\mathrm{z}\:\:\mathrm{in}\:\mathrm{each}\:\mathrm{case}: \\ $$$$\left.\mathrm{1}\right)\:\mathrm{arg}\left(\mathrm{i}−\mathrm{z}\right)=\mathrm{0}\left[\pi\right] \\ $$$$\left.\mathrm{2}\right)\:\mathrm{arg}\left(\mathrm{z}+\mathrm{1}−\mathrm{i}\right)=\frac{\pi}{\mathrm{6}}\left[\mathrm{2}\pi\right] \\ $$$$ \\ $$

Question Number 120151 Answers: 0 Comments: 0

Represent in complex plane the set of points M which have as affix z such that ∣z∣=2 and arg(z+1)=(π/4)[π]

$$\mathrm{Represent}\:\mathrm{in}\:\mathrm{complex}\:\mathrm{plane}\:\mathrm{the}\:\mathrm{set}\:\mathrm{of}\:\mathrm{points} \\ $$$$\mathrm{M}\:\mathrm{which}\:\mathrm{have}\:\mathrm{as}\:\mathrm{affix}\:\mathrm{z}\:\mathrm{such}\:\mathrm{that}\:\mid\mathrm{z}\mid=\mathrm{2}\:\mathrm{and} \\ $$$$\mathrm{arg}\left(\mathrm{z}+\mathrm{1}\right)=\frac{\pi}{\mathrm{4}}\left[\pi\right] \\ $$

Question Number 120110 Answers: 2 Comments: 0

{ ((((4x^2 )/(4x^2 +1)) = y)),((((4y^2 )/(4y^2 +1)) = z )),((((4z^2 )/(4z^2 +1)) = x)) :} where x,y,z ≠ 0

$$\begin{cases}{\frac{\mathrm{4}{x}^{\mathrm{2}} }{\mathrm{4}{x}^{\mathrm{2}} +\mathrm{1}}\:=\:{y}}\\{\frac{\mathrm{4}{y}^{\mathrm{2}} }{\mathrm{4}{y}^{\mathrm{2}} +\mathrm{1}}\:=\:{z}\:}\\{\frac{\mathrm{4}{z}^{\mathrm{2}} }{\mathrm{4}{z}^{\mathrm{2}} +\mathrm{1}}\:=\:{x}}\end{cases} \\ $$$${where}\:{x},{y},{z}\:\neq\:\mathrm{0}\: \\ $$

Question Number 120108 Answers: 0 Comments: 0

(x^4 +ax^2 +a^2 )^2 +x^6 =1 solve for: x,a∈R

$$\left(\boldsymbol{\mathrm{x}}^{\mathrm{4}} +\boldsymbol{\mathrm{ax}}^{\mathrm{2}} +\boldsymbol{\mathrm{a}}^{\mathrm{2}} \right)^{\mathrm{2}} +\boldsymbol{\mathrm{x}}^{\mathrm{6}} =\mathrm{1} \\ $$$$\boldsymbol{\mathrm{solve}}\:\boldsymbol{\mathrm{for}}:\:\:\mathrm{x},\mathrm{a}\in\boldsymbol{\mathrm{R}} \\ $$

Question Number 120036 Answers: 1 Comments: 0

Question Number 120035 Answers: 1 Comments: 0

Question Number 120006 Answers: 0 Comments: 0

Question Number 119997 Answers: 4 Comments: 0

solve for x,a∈R. (√(x^2 +ax+a^2 ))+(√(x^2 −ax+a^2 ))=1

$$\mathrm{solve}\:\mathrm{for}\:\mathrm{x},\mathrm{a}\in\boldsymbol{\mathrm{R}}. \\ $$$$\sqrt{\boldsymbol{{x}}^{\mathrm{2}} +\boldsymbol{{ax}}+\boldsymbol{{a}}^{\mathrm{2}} }+\sqrt{\boldsymbol{{x}}^{\mathrm{2}} −\boldsymbol{{ax}}+\boldsymbol{{a}}^{\mathrm{2}} }=\mathrm{1} \\ $$

Question Number 119996 Answers: 1 Comments: 0

{ ((x^3 +y^2 =a)),((x^2 +y^3 =b)) :} [solve for:x,y,a≠b∈R]

$$\begin{cases}{\boldsymbol{{x}}^{\mathrm{3}} +\boldsymbol{{y}}^{\mathrm{2}} =\boldsymbol{{a}}}\\{\boldsymbol{{x}}^{\mathrm{2}} +\boldsymbol{{y}}^{\mathrm{3}} =\boldsymbol{{b}}}\end{cases}\:\:\:\left[\boldsymbol{{solve}}\:\boldsymbol{{for}}:\mathrm{x},\mathrm{y},\mathrm{a}\neq\mathrm{b}\in\boldsymbol{\mathrm{R}}\right] \\ $$

Question Number 119979 Answers: 3 Comments: 1

Question Number 119939 Answers: 3 Comments: 0

Question Number 119921 Answers: 2 Comments: 0

solving the following system of equations { ((((3x−y)/(x−3y))=x^2 )),((((3y−z)/(y−3z))=y^2 )),((((3z−x)/(z−3x))=z^2 )) :}

$${solving}\:{the}\:{following}\:{system}\:{of}\:{equations} \\ $$$$\begin{cases}{\frac{\mathrm{3}{x}−{y}}{{x}−\mathrm{3}{y}}={x}^{\mathrm{2}} }\\{\frac{\mathrm{3}{y}−{z}}{{y}−\mathrm{3}{z}}={y}^{\mathrm{2}} }\\{\frac{\mathrm{3}{z}−{x}}{{z}−\mathrm{3}{x}}={z}^{\mathrm{2}} }\end{cases} \\ $$

Question Number 119894 Answers: 2 Comments: 0

Pg 222 Pg 223 Pg 224 Pg 225 Pg 226 Pg 227 Pg 228 Pg 229 Pg 230 Pg 231

Terms of Service

Contact: info@tinkutara.com