Question and Answers Forum

All Questions Topic List

AlgebraQuestion and Answers: Page 226

Question Number 125900 Answers: 0 Comments: 1

Question Number 125890 Answers: 0 Comments: 1

(Z^(+,∗) ) where x^∗ y= x−y for all x,y ∈ z^+ .

$$\left(\mathbb{Z}^{+,\ast} \right)\:{where}\:{x}^{\ast} {y}=\:{x}−{y}\:{for}\:{all}\:{x},{y}\:\in\:{z}^{+} . \\ $$

Question Number 125873 Answers: 1 Comments: 0

Question Number 125868 Answers: 1 Comments: 0

Question Number 125864 Answers: 1 Comments: 0

(1/(1+1^2 +1^4 ))+(2/(1+2^2 +2^4 ))+(3/(1+3^2 +3^4 ))+...+((50)/(1+50^2 +50^4 ))=?

$$\frac{\mathrm{1}}{\mathrm{1}+\mathrm{1}^{\mathrm{2}} +\mathrm{1}^{\mathrm{4}} }+\frac{\mathrm{2}}{\mathrm{1}+\mathrm{2}^{\mathrm{2}} +\mathrm{2}^{\mathrm{4}} }+\frac{\mathrm{3}}{\mathrm{1}+\mathrm{3}^{\mathrm{2}} +\mathrm{3}^{\mathrm{4}} }+...+\frac{\mathrm{50}}{\mathrm{1}+\mathrm{50}^{\mathrm{2}} +\mathrm{50}^{\mathrm{4}} }=? \\ $$

Question Number 125785 Answers: 1 Comments: 0

⊕+⊕+⊕=33 ▲+▲+9▼=25 9▼+▲+2★=19 2⊕+8▼ ∙ ★= ?

$$\oplus+\oplus+\oplus=\mathrm{33} \\ $$$$\blacktriangle+\blacktriangle+\mathrm{9}\blacktrinagledown=\mathrm{25} \\ $$$$\mathrm{9}\blacktrinagledown+\blacktriangle+\mathrm{2}\bigstar=\mathrm{19} \\ $$$$\mathrm{2}\oplus+\mathrm{8}\blacktrinagledown\:\centerdot\:\bigstar=\:? \\ $$$$\:\: \\ $$

Question Number 125743 Answers: 0 Comments: 7

x ; y ; z → simple numbers , y<x<z , y+x+z=68 , y ∙ x + x ∙ z + z ∙ y = 1121 , y ∙ x = ?

$$\boldsymbol{{x}}\:;\:\boldsymbol{{y}}\:;\:\boldsymbol{{z}}\:\rightarrow\:\boldsymbol{{simple}}\:\boldsymbol{{numbers}}\:, \\ $$$$\boldsymbol{{y}}<\boldsymbol{{x}}<\boldsymbol{{z}}\:, \\ $$$$\boldsymbol{{y}}+\boldsymbol{{x}}+\boldsymbol{{z}}=\mathrm{68}\:, \\ $$$$\boldsymbol{{y}}\:\centerdot\:\boldsymbol{{x}}\:+\:\boldsymbol{{x}}\:\centerdot\:\boldsymbol{{z}}\:+\:\boldsymbol{{z}}\:\centerdot\:\boldsymbol{{y}}\:=\:\mathrm{1121}\:, \\ $$$$\boldsymbol{{y}}\:\centerdot\:\boldsymbol{{x}}\:=\:? \\ $$

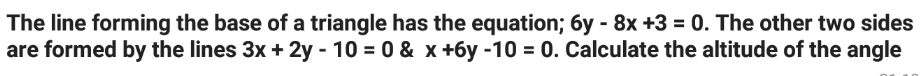
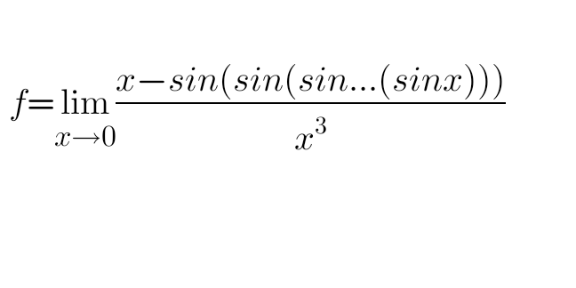
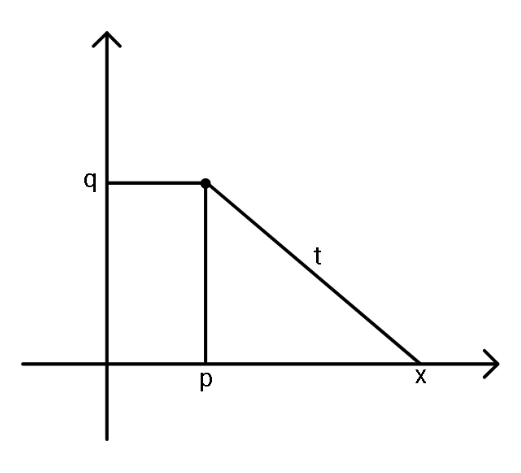
Question Number 125686 Answers: 0 Comments: 1

show that cos((4π)/5)+cos((2π)/5)+1=0

$${show}\:{that} \\ $$$${cos}\frac{\mathrm{4}\pi}{\mathrm{5}}+{cos}\frac{\mathrm{2}\pi}{\mathrm{5}}+\mathrm{1}=\mathrm{0} \\ $$

Question Number 125670 Answers: 1 Comments: 0

N<10200 , N has five digits. N≡22[23] and N≡5[17]. Determinate the integer N.

$${N}<\mathrm{10200}\:,\:{N}\:{has}\:{five}\:{digits}. \\ $$$${N}\equiv\mathrm{22}\left[\mathrm{23}\right]\:{and}\:{N}\equiv\mathrm{5}\left[\mathrm{17}\right]. \\ $$$${Determinate}\:{the}\:{integer}\:{N}. \\ $$

Question Number 125669 Answers: 1 Comments: 0

we are in C. solve z^5 =1. show that the sum of its solutions is null the deduct that cos(((2π)/5))+cos(((4π)/5))=−(1/2)

$${we}\:{are}\:{in}\:\mathbb{C}. \\ $$$${solve}\:{z}^{\mathrm{5}} =\mathrm{1}. \\ $$$${show}\:{that}\:{the}\:{sum}\:{of}\:{its}\:{solutions}\:{is} \\ $$$${null}\:{the}\:{deduct}\:{that}\:{cos}\left(\frac{\mathrm{2}\pi}{\mathrm{5}}\right)+{cos}\left(\frac{\mathrm{4}\pi}{\mathrm{5}}\right)=−\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Question Number 125654 Answers: 1 Comments: 1

Question Number 125632 Answers: 1 Comments: 0

Question Number 125577 Answers: 1 Comments: 1

Question Number 125539 Answers: 2 Comments: 1

Question Number 125504 Answers: 1 Comments: 0

n!=2^(10) ∙10!(1∙3∙5∙7∙∙∙19) n=??? help me

$${n}!=\mathrm{2}^{\mathrm{10}} \centerdot\mathrm{10}!\left(\mathrm{1}\centerdot\mathrm{3}\centerdot\mathrm{5}\centerdot\mathrm{7}\centerdot\centerdot\centerdot\mathrm{19}\right)\:\:\:\:\:\:\:\:{n}=??? \\ $$$${help}\:{me} \\ $$

Question Number 125465 Answers: 1 Comments: 0

Question Number 125464 Answers: 0 Comments: 1

Question Number 125442 Answers: 1 Comments: 0

find 2^2^2^2

$${find}\:\mathrm{2}^{\mathrm{2}^{\mathrm{2}^{\mathrm{2}} } } \\ $$

Question Number 125428 Answers: 0 Comments: 1

Question Number 125288 Answers: 1 Comments: 2

Question Number 125262 Answers: 0 Comments: 1

Find GP the matrix permutation where P = ((a,b,c,d),(b,c,a,d) ) and G = ((a,b,c,d),(a,c,d,b) ) explain

$$\mathrm{Find}\:{GP}\:\mathrm{the}\:\mathrm{matrix}\:\mathrm{permutation}\:\mathrm{where} \\ $$$$\:{P}\:=\:\begin{pmatrix}{{a}}&{{b}}&{{c}}&{{d}}\\{{b}}&{{c}}&{{a}}&{{d}}\end{pmatrix}\:\mathrm{and}\:{G}\:=\:\begin{pmatrix}{{a}}&{{b}}&{{c}}&{{d}}\\{{a}}&{{c}}&{{d}}&{{b}}\end{pmatrix} \\ $$$$\mathrm{explain} \\ $$

Question Number 125258 Answers: 0 Comments: 1

a,b,c => ▲ tomonlari (p−a)(p−b)+(p−c)(p−a)+(p−b)(p−c)≥(√3) S

$${a},{b},{c}\:\:=>\:\:\blacktriangle\:\boldsymbol{{tomonlari}} \\ $$$$\left(\boldsymbol{{p}}−\boldsymbol{{a}}\right)\left(\boldsymbol{{p}}−\boldsymbol{{b}}\right)+\left(\boldsymbol{{p}}−\boldsymbol{{c}}\right)\left(\boldsymbol{{p}}−\boldsymbol{{a}}\right)+\left(\boldsymbol{{p}}−\boldsymbol{{b}}\right)\left(\boldsymbol{{p}}−\boldsymbol{{c}}\right)\geqslant\sqrt{\mathrm{3}}\:\boldsymbol{{S}} \\ $$

Question Number 125226 Answers: 0 Comments: 5

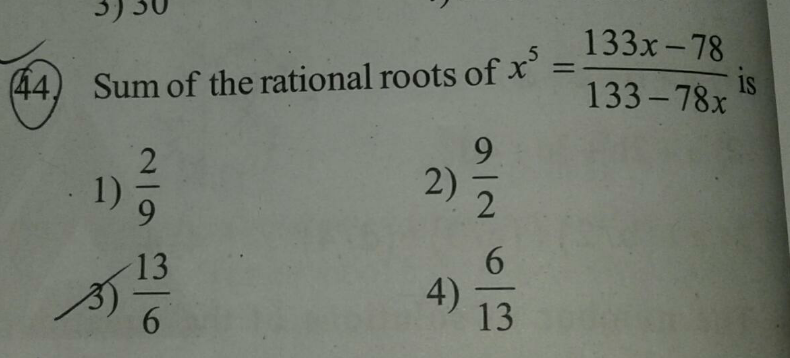
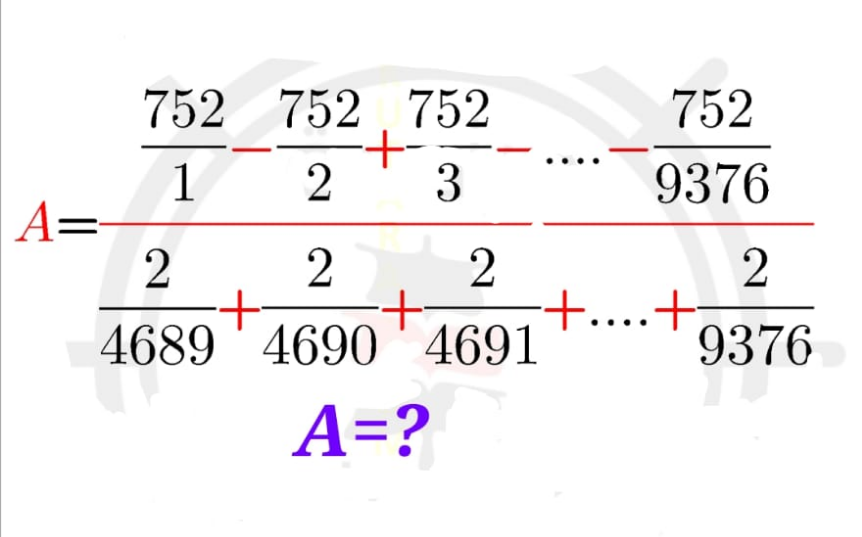
Question Number 125202 Answers: 2 Comments: 0

Solve the reccurence relation a_n = 2(a_(n−1) −a_(n−2) ) ; given a_0 =1 and a_1 = 0.

$$\:{Solve}\:{the}\:{reccurence}\:{relation} \\ $$$${a}_{{n}} \:=\:\mathrm{2}\left({a}_{{n}−\mathrm{1}} −{a}_{{n}−\mathrm{2}} \right)\:;\:{given}\:{a}_{\mathrm{0}} =\mathrm{1}\: \\ $$$${and}\:{a}_{\mathrm{1}} =\:\mathrm{0}. \\ $$

Question Number 125186 Answers: 1 Comments: 0

Question Number 125169 Answers: 0 Comments: 3

algebra

$${algebra} \\ $$

Pg 221 Pg 222 Pg 223 Pg 224 Pg 225 Pg 226 Pg 227 Pg 228 Pg 229 Pg 230

Contact: info@tinkutara.com