Question and Answers Forum

AlgebraQuestion and Answers: Page 222

Question Number 133996 Answers: 2 Comments: 0

show that (√2)<log_2 3<(√3)

$${show}\:{that}\:\sqrt{\mathrm{2}}<\mathrm{log}_{\mathrm{2}} \:\mathrm{3}<\sqrt{\mathrm{3}} \\ $$

Question Number 133991 Answers: 1 Comments: 0

∣ 3x−∣ 4x+2 ∣∣ ≥ 4 > ∣ 5x+8 ∣

$$\:\mid\:\mathrm{3x}−\mid\:\mathrm{4x}+\mathrm{2}\:\mid\mid\:\geqslant\:\mathrm{4}\:>\:\mid\:\mathrm{5x}+\mathrm{8}\:\mid\: \\ $$

Question Number 133903 Answers: 0 Comments: 3

Question Number 133885 Answers: 1 Comments: 2

Consider the equations of two intersecting straight lines { ((ax+by+c=0)),((a_1 x+b_1 y+c_1 =0)) :} Find the equation of straight line passing through a given point (x_0 ,y_0 ) and the intersection point of the given straight lines.

$$\:\mathrm{Consider}\:\mathrm{the}\:\mathrm{equations}\:\mathrm{of}\:\mathrm{two} \\ $$$$\mathrm{intersecting}\:\mathrm{straight}\:\mathrm{lines} \\ $$$$\begin{cases}{{ax}+{by}+{c}=\mathrm{0}}\\{{a}_{\mathrm{1}} {x}+{b}_{\mathrm{1}} {y}+{c}_{\mathrm{1}} =\mathrm{0}}\end{cases} \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{of}\:\mathrm{straight}\:\mathrm{line} \\ $$$$\mathrm{passing}\:\mathrm{through}\:\mathrm{a}\:\mathrm{given}\:\mathrm{point} \\ $$$$\left(\mathrm{x}_{\mathrm{0}} ,\mathrm{y}_{\mathrm{0}} \right)\:\mathrm{and}\:\mathrm{the}\:\mathrm{intersection}\:\mathrm{point} \\ $$$$\mathrm{of}\:\mathrm{the}\:\mathrm{given}\:\mathrm{straight}\:\mathrm{lines}. \\ $$

Question Number 133793 Answers: 0 Comments: 1

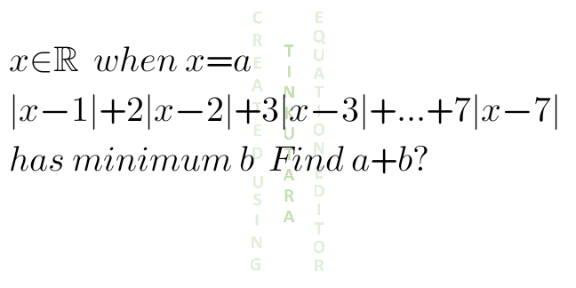
Question Number 133981 Answers: 2 Comments: 0

If x = 5+2(√6) then ((x−1)/( (√x))) =?

$$\mathrm{If}\:{x}\:=\:\mathrm{5}+\mathrm{2}\sqrt{\mathrm{6}}\:\mathrm{then}\:\frac{{x}−\mathrm{1}}{\:\sqrt{{x}}}\:=? \\ $$

Question Number 133783 Answers: 0 Comments: 1

To complete a job, 24 worker are needed in 35 days. After they worked for 8 days , half of the workers stopped working . In order for work to be completed , an additional time of .... days needed (a) 54 (b) 38 (c) 28 (d) 14 (e) 19

$$\mathrm{To}\:\mathrm{complete}\:\mathrm{a}\:\mathrm{job},\:\mathrm{24}\:\mathrm{worker}\:\mathrm{are} \\ $$$$\mathrm{needed}\:\mathrm{in}\:\mathrm{35}\:\mathrm{days}.\:\mathrm{After}\:\mathrm{they}\:\mathrm{worked} \\ $$$$\mathrm{for}\:\mathrm{8}\:\mathrm{days}\:,\:\mathrm{half}\:\mathrm{of}\:\mathrm{the}\:\mathrm{workers}\: \\ $$$$\mathrm{stopped}\:\mathrm{working}\:.\:\mathrm{In}\:\mathrm{order}\:\mathrm{for} \\ $$$$\mathrm{work}\:\mathrm{to}\:\mathrm{be}\:\mathrm{completed}\:,\:\mathrm{an}\:\mathrm{additional} \\ $$$$\mathrm{time}\:\mathrm{of}\:....\:\mathrm{days}\:\mathrm{needed} \\ $$$$\left(\mathrm{a}\right)\:\mathrm{54}\:\:\:\:\:\left(\mathrm{b}\right)\:\mathrm{38}\:\:\:\:\left(\mathrm{c}\right)\:\mathrm{28}\:\:\:\:\:\left(\mathrm{d}\right)\:\mathrm{14} \\ $$$$\left(\mathrm{e}\right)\:\mathrm{19}\: \\ $$

Question Number 133738 Answers: 3 Comments: 0

Question Number 133664 Answers: 1 Comments: 0

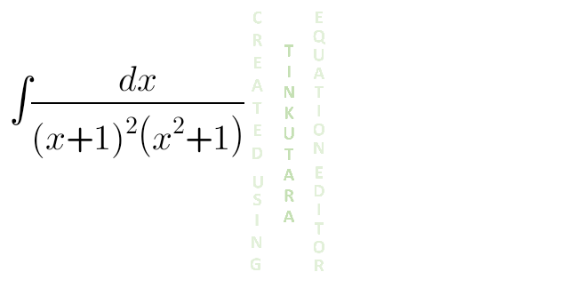
Question Number 133653 Answers: 1 Comments: 0

hi, everybody ! how to prove that 𝛑 is an irrational number ???

$$\boldsymbol{\mathrm{hi}},\:\boldsymbol{\mathrm{everybody}}\:! \\ $$$$\boldsymbol{\mathrm{how}}\:\boldsymbol{\mathrm{to}}\:\boldsymbol{\mathrm{prove}}\:\boldsymbol{\mathrm{that}}\:\boldsymbol{\pi}\:\boldsymbol{\mathrm{is}}\:\boldsymbol{\mathrm{an}}\:\boldsymbol{\mathrm{irrational}}\:\boldsymbol{\mathrm{number}}\:??? \\ $$

Question Number 133612 Answers: 0 Comments: 2

If { ((x+y+2z=k)),((x+2y+z=k)),((2x+y+z=k)) :} ; k≠ 0 then x^2 +y^2 +z^2 =?

$$\mathrm{If}\:\begin{cases}{\mathrm{x}+\mathrm{y}+\mathrm{2z}=\mathrm{k}}\\{\mathrm{x}+\mathrm{2y}+\mathrm{z}=\mathrm{k}}\\{\mathrm{2x}+\mathrm{y}+\mathrm{z}=\mathrm{k}}\end{cases}\:;\:\mathrm{k}\neq\:\mathrm{0} \\ $$$$\mathrm{then}\:\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{z}^{\mathrm{2}} \:=? \\ $$$$ \\ $$

Question Number 133568 Answers: 2 Comments: 0

Proof the series Σ_(n=1) ^∞ (2/(9+2n(ln n)^2 )) convergent

$$\mathrm{Proof}\:\mathrm{the}\:\mathrm{series}\:\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\:\frac{\mathrm{2}}{\mathrm{9}+\mathrm{2n}\left(\mathrm{ln}\:\mathrm{n}\right)^{\mathrm{2}} } \\ $$$$\mathrm{convergent} \\ $$$$ \\ $$

Question Number 133542 Answers: 0 Comments: 1

Question Number 133469 Answers: 1 Comments: 0

find x in terms of a ((1+x−(√(2x+x^2 )))/(1+x+(√(2x+x^2 ))))=a^3 (((√(2+x))+(√x))/( (√(2+x))−(√x)))

$${find}\:{x}\:{in}\:{terms}\:{of}\:{a} \\ $$$$\frac{\mathrm{1}+{x}−\sqrt{\mathrm{2}{x}+{x}^{\mathrm{2}} }}{\mathrm{1}+{x}+\sqrt{\mathrm{2}{x}+{x}^{\mathrm{2}} }}={a}^{\mathrm{3}} \frac{\sqrt{\mathrm{2}+{x}}+\sqrt{{x}}}{\:\sqrt{\mathrm{2}+{x}}−\sqrt{{x}}} \\ $$

Question Number 133450 Answers: 0 Comments: 0

$$ \\ $$

Question Number 133423 Answers: 1 Comments: 4

Question Number 133318 Answers: 1 Comments: 0

(a) Are there any graphs with 5 vertices which have vertices of degrees 1,2,3,4 and 5?

$$\left(\mathrm{a}\right)\:\mathrm{Are}\:\mathrm{there}\:\mathrm{any}\:\mathrm{graphs}\:\mathrm{with}\:\mathrm{5} \\ $$$$\mathrm{vertices}\:\mathrm{which}\:\mathrm{have}\:\mathrm{vertices}\:\mathrm{of}\: \\ $$$$\mathrm{degrees}\:\mathrm{1},\mathrm{2},\mathrm{3},\mathrm{4}\:\mathrm{and}\:\mathrm{5}?\: \\ $$

Question Number 133279 Answers: 0 Comments: 0

hi, everybody ! with n ∈ N, prove that : ∃ n_0 ∈ N / ∀ n ≥ n_0 , n^2 ≤ 2^n .

$$\boldsymbol{\mathrm{hi}},\:\boldsymbol{\mathrm{everybody}}\:! \\ $$$$\boldsymbol{\mathrm{with}}\:\boldsymbol{\mathrm{n}}\:\in\:\mathbb{N}, \\ $$$$\boldsymbol{\mathrm{prove}}\:\boldsymbol{\mathrm{that}}\::\:\exists\:\boldsymbol{\mathrm{n}}_{\mathrm{0}} \:\in\:\mathbb{N}\:/\:\forall\:\boldsymbol{\mathrm{n}}\:\geqslant\:\boldsymbol{\mathrm{n}}_{\mathrm{0}} \:,\:\boldsymbol{\mathrm{n}}^{\mathrm{2}} \:\leqslant\:\mathrm{2}^{\boldsymbol{\mathrm{n}}} . \\ $$

Question Number 133201 Answers: 2 Comments: 0

(√(81))

$$\sqrt{\mathrm{81}} \\ $$

Question Number 133180 Answers: 1 Comments: 3

Question Number 133072 Answers: 1 Comments: 0

Show that (5/(2−(3)^(1/4) )) is in F_2 by expressing the number in form a_1 +b_1 (√k_1 ) where a_1 ,b_1 , k_1 are in F_1

$$\mathrm{Show}\:\mathrm{that}\:\frac{\mathrm{5}}{\mathrm{2}−\sqrt[{\mathrm{4}}]{\mathrm{3}}}\:\mathrm{is}\:\mathrm{in}\:\mathrm{F}_{\mathrm{2}} \:\mathrm{by}\:\mathrm{expressing} \\ $$$$\mathrm{the}\:\mathrm{number}\:\mathrm{in}\:\mathrm{form}\:{a}_{\mathrm{1}} +{b}_{\mathrm{1}} \sqrt{{k}_{\mathrm{1}} }\:\mathrm{where} \\ $$$${a}_{\mathrm{1}} ,{b}_{\mathrm{1}} ,\:{k}_{\mathrm{1}} \:{are}\:{in}\:{F}_{\mathrm{1}} \\ $$

Question Number 133053 Answers: 1 Comments: 0

When the polynomial f(x) is divided by (x−2) the remainder is 4 and when it is divided (x−3) the remainder is 7. Given that f(x) may be written in the formf(x)=(x−2)(x−3)Q(x)+ax+b, find the remainder when f(x) is divided by (x−2)(x−3). If also f(x) is a cubic function in which the coefficient of x^3 is unity and f(1)=1, determine Q(x).

$$\mathrm{When}\:\mathrm{the}\:\mathrm{polynomial}\:\mathrm{f}\left({x}\right)\:\mathrm{is}\:\mathrm{divided}\:\mathrm{by} \\ $$$$\left(\mathrm{x}−\mathrm{2}\right)\:\mathrm{the}\:\mathrm{remainder}\:\mathrm{is}\:\mathrm{4}\:\mathrm{and}\:\mathrm{when}\:\mathrm{it}\:\mathrm{is}\:\mathrm{divided} \\ $$$$\left(\mathrm{x}−\mathrm{3}\right)\:\mathrm{the}\:\mathrm{remainder}\:\mathrm{is}\:\mathrm{7}.\:\mathrm{Given}\:\mathrm{that}\:\mathrm{f}\left({x}\right) \\ $$$$\mathrm{may}\:\mathrm{be}\:\mathrm{written}\:\mathrm{in}\:\mathrm{the}\:\mathrm{formf}\left({x}\right)=\left(\mathrm{x}−\mathrm{2}\right)\left(\mathrm{x}−\mathrm{3}\right)\mathrm{Q}\left(\mathrm{x}\right)+\mathrm{ax}+\mathrm{b}, \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{remainder}\:\mathrm{when}\:\mathrm{f}\left(\mathrm{x}\right)\:\mathrm{is}\:\mathrm{divided} \\ $$$$\mathrm{by}\:\left(\mathrm{x}−\mathrm{2}\right)\left(\mathrm{x}−\mathrm{3}\right).\:\mathrm{If}\:\mathrm{also}\:\mathrm{f}\left(\mathrm{x}\right)\:\mathrm{is}\:\mathrm{a}\:\mathrm{cubic}\:\mathrm{function} \\ $$$$\mathrm{in}\:\mathrm{which}\:\mathrm{the}\:\mathrm{coefficient}\:\mathrm{of}\:\mathrm{x}^{\mathrm{3}} \:\mathrm{is}\:\mathrm{unity}\:\mathrm{and} \\ $$$$\mathrm{f}\left(\mathrm{1}\right)=\mathrm{1},\:\mathrm{determine}\:\mathrm{Q}\left(\mathrm{x}\right). \\ $$

Question Number 133009 Answers: 1 Comments: 1

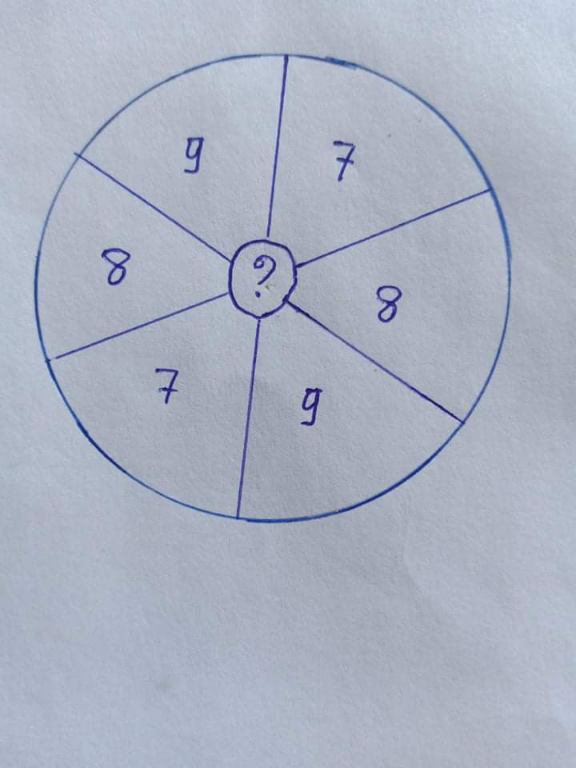
Question Number 132961 Answers: 1 Comments: 0

Question Number 132900 Answers: 0 Comments: 1

a pinhole camera is placed 300cm in front of a building so that the image is formed on a screen 5cm from the pin hole. if the image is 2.5cm high. the height of the building will be?

$${a}\:{pinhole}\:{camera}\:{is}\:{placed}\:\mathrm{300}{cm} \\ $$$${in}\:{front}\:{of}\:{a}\:{building}\:{so}\:{that}\:{the}\:{image} \\ $$$${is}\:{formed}\:{on}\:{a}\:{screen}\:\mathrm{5}{cm}\:{from}\:{the} \\ $$$${pin}\:{hole}.\:{if}\:{the}\:{image}\:{is}\:\mathrm{2}.\mathrm{5}{cm}\:{high}. \\ $$$${the}\:{height}\:{of}\:{the}\:{building}\:{will}\:{be}? \\ $$

Question Number 132697 Answers: 3 Comments: 2

Find the condition that one root of ax^2 +bx+c = 0 ,a≠ 0 is square of the other .

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{condition}\:\mathrm{that}\:\mathrm{one} \\ $$$$\mathrm{root}\:\mathrm{of}\:{ax}^{\mathrm{2}} +{bx}+{c}\:=\:\mathrm{0}\:,{a}\neq\:\mathrm{0} \\ $$$$\mathrm{is}\:\mathrm{square}\:\mathrm{of}\:\mathrm{the}\:\mathrm{other}\:. \\ $$

Pg 217 Pg 218 Pg 219 Pg 220 Pg 221 Pg 222 Pg 223 Pg 224 Pg 225 Pg 226

Contact: info@tinkutara.com