Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 176555 by Shrinava last updated on 21/Sep/22

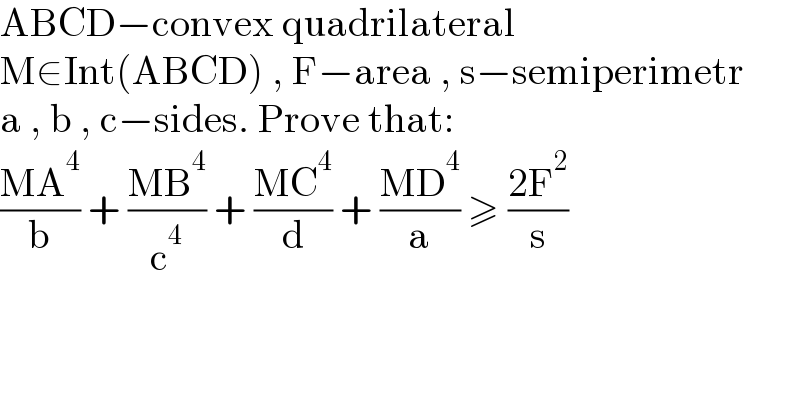
ABCD−convex quadrilateral M∈Int(ABCD) , F−area , s−semiperimetr a , b , c−sides. Prove that: ((MA^4 )/b) + ((MB^4 )/c^4 ) + ((MC^4 )/d) + ((MD^4 )/a) ≥ ((2F^2 )/s)

$$\mathrm{ABCD}−\mathrm{convex}\:\mathrm{quadrilateral} \\ $$$$\mathrm{M}\in\mathrm{Int}\left(\mathrm{ABCD}\right)\:,\:\mathrm{F}−\mathrm{area}\:,\:\mathrm{s}−\mathrm{semiperimetr} \\ $$$$\mathrm{a}\:,\:\mathrm{b}\:,\:\mathrm{c}−\mathrm{sides}.\:\mathrm{Prove}\:\mathrm{that}: \\ $$$$\frac{\mathrm{MA}^{\mathrm{4}} }{\mathrm{b}}\:+\:\frac{\mathrm{MB}^{\mathrm{4}} }{\mathrm{c}^{\mathrm{4}} }\:+\:\frac{\mathrm{MC}^{\mathrm{4}} }{\mathrm{d}}\:+\:\frac{\mathrm{MD}^{\mathrm{4}} }{\mathrm{a}}\:\geqslant\:\frac{\mathrm{2F}^{\mathrm{2}} }{\mathrm{s}} \\ $$

Commented by mr W last updated on 21/Sep/22

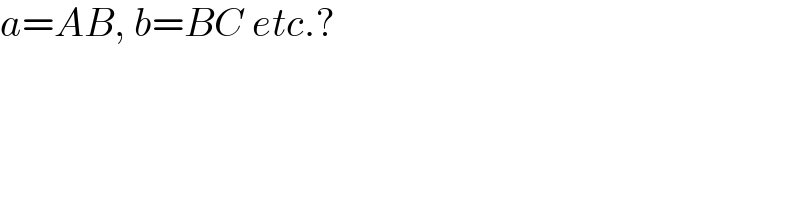
a=AB, b=BC etc.?

$${a}={AB},\:{b}={BC}\:{etc}.? \\ $$

Commented by Shrinava last updated on 21/Sep/22

yes dear professor

$$\mathrm{yes}\:\mathrm{dear}\:\mathrm{professor} \\ $$

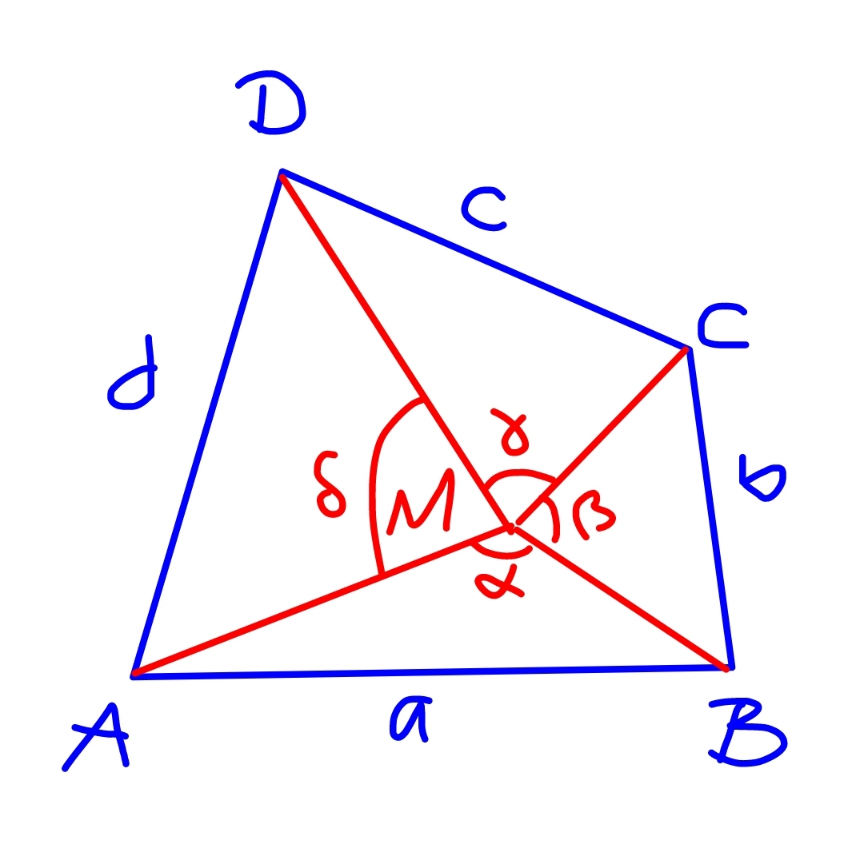
Answered by mr W last updated on 24/Sep/22

Commented by Shrinava last updated on 27/Sep/22

thank you dear professor so that′s it?

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{dear}\:\mathrm{professor} \\ $$$$\mathrm{so}\:\mathrm{that}'\mathrm{s}\:\mathrm{it}? \\ $$

Commented by mr W last updated on 04/Oct/22

s=((a+b+c+d)/2) F=((MA×MB×sin α)/2)+((MB×MC×sin β)/2)+((MC×MD×sin γ)/2)+((MD×MA×sin δ)/2) ≤(1/2)(MA×MB+MB×MC+MC×MD+MD×MA) ≤(1/2)(((MA^2 +MB^2 )/2)+((MB^2 +MC^2 )/2)+((MC^2 +MD^2 )/2)+((MD^2 +MA^2 )/2)) =((MA^2 +MB^2 +MC^2 +MD^2 )/2) ((2F^2 )/s)≤(((MA^2 +MB^2 +MC^2 +MD^2 )^2 )/(a+b+c+d)) ≤((MA^4 )/b)+((MB^4 )/c)+((MC^4 )/d)+((MD^4 )/a) ∗) proved! ✓ ∗) see Chaucy−Schwarz Inequality

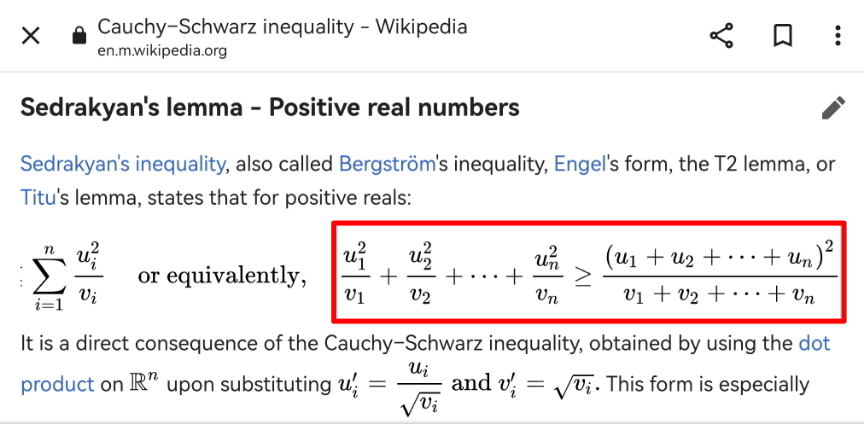
$${s}=\frac{{a}+{b}+{c}+{d}}{\mathrm{2}} \\ $$$${F}=\frac{{MA}×{MB}×\mathrm{sin}\:\alpha}{\mathrm{2}}+\frac{{MB}×{MC}×\mathrm{sin}\:\beta}{\mathrm{2}}+\frac{{MC}×{MD}×\mathrm{sin}\:\gamma}{\mathrm{2}}+\frac{{MD}×{MA}×\mathrm{sin}\:\delta}{\mathrm{2}} \\ $$$$\:\:\:\leqslant\frac{\mathrm{1}}{\mathrm{2}}\left({MA}×{MB}+{MB}×{MC}+{MC}×{MD}+{MD}×{MA}\right) \\ $$$$\:\:\:\leqslant\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{{MA}^{\mathrm{2}} +{MB}^{\mathrm{2}} }{\mathrm{2}}+\frac{{MB}^{\mathrm{2}} +{MC}^{\mathrm{2}} }{\mathrm{2}}+\frac{{MC}^{\mathrm{2}} +{MD}^{\mathrm{2}} }{\mathrm{2}}+\frac{{MD}^{\mathrm{2}} +{MA}^{\mathrm{2}} }{\mathrm{2}}\right) \\ $$$$\:\:\:=\frac{{MA}^{\mathrm{2}} +{MB}^{\mathrm{2}} +{MC}^{\mathrm{2}} +{MD}^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\frac{\mathrm{2}{F}^{\mathrm{2}} }{{s}}\leqslant\frac{\left({MA}^{\mathrm{2}} +{MB}^{\mathrm{2}} +{MC}^{\mathrm{2}} +{MD}^{\mathrm{2}} \right)^{\mathrm{2}} }{{a}+{b}+{c}+{d}} \\ $$$$\left.\:\:\:\:\:\:\:\leqslant\frac{{MA}^{\mathrm{4}} }{{b}}+\frac{{MB}^{\mathrm{4}} }{{c}}+\frac{{MC}^{\mathrm{4}} }{{d}}+\frac{{MD}^{\mathrm{4}} }{{a}}\:\:\:\:\:\:\ast\right) \\ $$$$\:\:\:\:\:\:\:{proved}!\:\checkmark \\ $$$$ \\ $$$$\left.\ast\right)\:\:{see}\:{Chaucy}−{Schwarz}\:{Inequality} \\ $$

Commented by Shrinava last updated on 24/Sep/22

thank you dear professor please

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{dear}\:\mathrm{professor}\:\mathrm{please} \\ $$

Commented by mr W last updated on 04/Oct/22

yes. now the proof is complete.

$${yes}.\:{now}\:{the}\:{proof}\:{is}\:{complete}. \\ $$

Commented by mr W last updated on 04/Oct/22

Commented by Shrinava last updated on 08/Oct/22

perfect dear professor thank you

$$\mathrm{perfect}\:\mathrm{dear}\:\mathrm{professor}\:\mathrm{thank}\:\mathrm{you} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com