
Question Number 115642 by 24224 Opiyo Kamuki last updated on 27/Sep/20
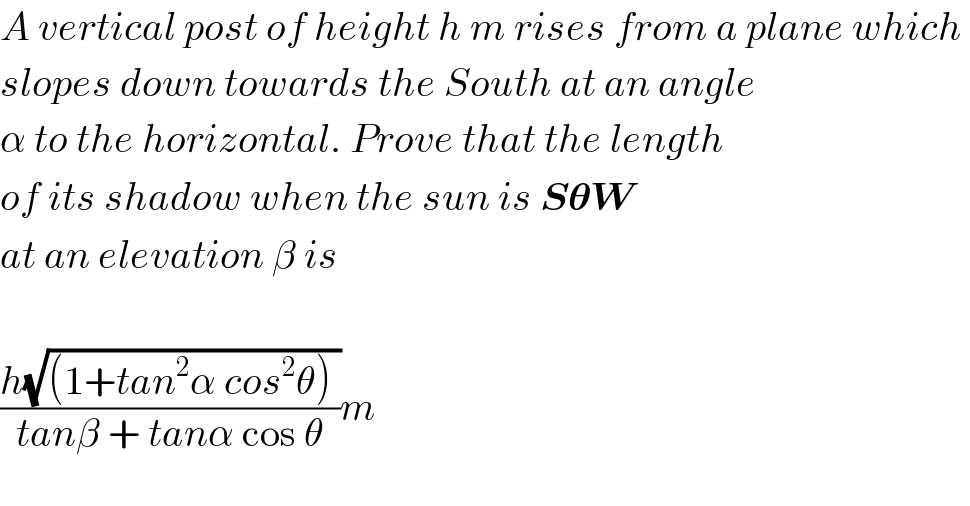
$${A}\:{vertical}\:{post}\:{of}\:{height}\:{h}\:{m}\:{rises}\:{from}\:{a}\:{plane}\:{which}\: \\ $$$${slopes}\:{down}\:{towards}\:{the}\:{South}\:{at}\:{an}\:{angle} \\ $$$$\alpha\:{to}\:{the}\:{horizontal}.\:{Prove}\:{that}\:{the}\:{length} \\ $$$${of}\:{its}\:{shadow}\:{when}\:{the}\:{sun}\:{is}\:\boldsymbol{{S}\theta{W}}\:\: \\ $$$${at}\:{an}\:{elevation}\:\beta\:{is} \\ $$$$ \\ $$$$\frac{{h}\sqrt{\left(\mathrm{1}+{tan}^{\mathrm{2}} \alpha\:{cos}^{\mathrm{2}} \theta\right)\:}}{{tan}\beta\:+\:{tan}\alpha\:\mathrm{cos}\:\theta}{m} \\ $$
