Question and Answers Forum

All Questions      Topic List

Probability and Statistics Questions

Previous in All Question      Next in All Question      

Previous in Probability and Statistics      Next in Probability and Statistics      

Question Number 114875 by bobhans last updated on 21/Sep/20

A man sent 7 letters to his 7 friend . the letters are kept in addressed envelopes at random. the probability that 3 friends receive correct letters and 4 letters go to wrong destination is _ (old question unanswered)

$${A}\:{man}\:{sent}\:\mathrm{7}\:{letters}\:{to}\:{his}\:\mathrm{7}\:{friend}\:. \\ $$$${the}\:{letters}\:{are}\:{kept}\:{in}\:{addressed}\:{envelopes} \\ $$$${at}\:{random}.\:{the}\:{probability}\:{that}\:\mathrm{3}\:{friends} \\ $$$${receive}\:{correct}\:{letters}\:{and}\:\mathrm{4}\:{letters}\:{go} \\ $$$${to}\:{wrong}\:{destination}\:{is}\:\_ \\ $$$$ \\ $$$$\left({old}\:{question}\:{unanswered}\right) \\ $$

Answered by PRITHWISH SEN 2 last updated on 21/Sep/20

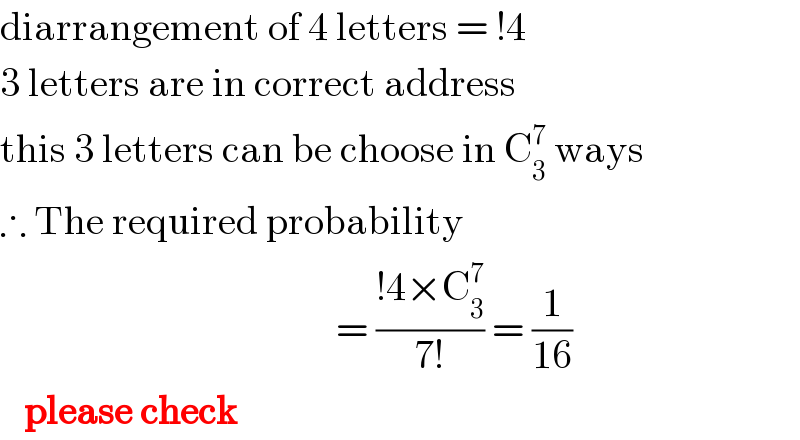
diarrangement of 4 letters = !4 3 letters are in correct address this 3 letters can be choose in C_3 ^7 ways ∴ The required probability = ((!4×C_3 ^7 )/(7!)) = (1/(16)) please check

$$\mathrm{diarrangement}\:\mathrm{of}\:\mathrm{4}\:\mathrm{letters}\:=\:!\mathrm{4} \\ $$$$\mathrm{3}\:\mathrm{letters}\:\mathrm{are}\:\mathrm{in}\:\mathrm{correct}\:\mathrm{address} \\ $$$$\mathrm{this}\:\mathrm{3}\:\mathrm{letters}\:\mathrm{can}\:\mathrm{be}\:\mathrm{choose}\:\mathrm{in}\:\mathrm{C}_{\mathrm{3}} ^{\mathrm{7}} \:\mathrm{ways} \\ $$$$\therefore\:\mathrm{The}\:\mathrm{required}\:\mathrm{probability} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\frac{!\mathrm{4}×\mathrm{C}_{\mathrm{3}} ^{\mathrm{7}} }{\mathrm{7}!}\:=\:\frac{\mathrm{1}}{\mathrm{16}} \\ $$$$\:\:\:\boldsymbol{\mathrm{please}}\:\boldsymbol{\mathrm{check}} \\ $$

Commented by mr W last updated on 21/Sep/20

correct!

$${correct}! \\ $$

Commented by PRITHWISH SEN 2 last updated on 21/Sep/20

thank you sir.

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir}. \\ $$

Commented by mr W last updated on 21/Sep/20

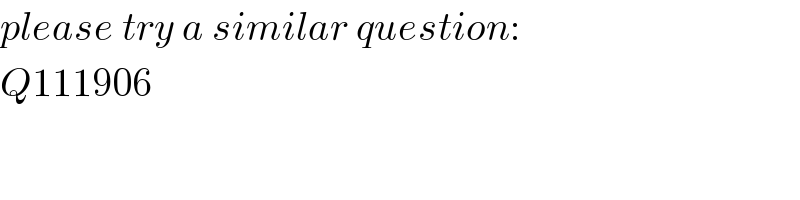
please try a similar question: Q111906

$${please}\:{try}\:{a}\:{similar}\:{question}: \\ $$$${Q}\mathrm{111906} \\ $$

Commented by john santu last updated on 21/Sep/20

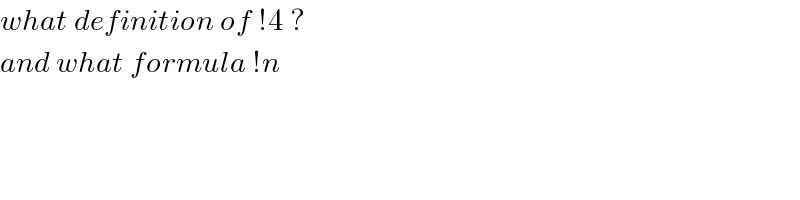
what definition of !4 ? and what formula !n

$${what}\:{definition}\:{of}\:!\mathrm{4}\:? \\ $$$${and}\:{what}\:{formula}\:!{n}\: \\ $$

Commented by PRITHWISH SEN 2 last updated on 22/Sep/20

!n = n![1−(1/(1!))+(1/(2!))−(1/(3!))+.......+(−1)^n (1/(n!))]

$$!\boldsymbol{\mathrm{n}}\:=\:\boldsymbol{\mathrm{n}}!\left[\mathrm{1}−\frac{\mathrm{1}}{\mathrm{1}!}+\frac{\mathrm{1}}{\mathrm{2}!}−\frac{\mathrm{1}}{\mathrm{3}!}+.......+\left(−\mathrm{1}\right)^{\mathrm{n}} \frac{\mathrm{1}}{\mathrm{n}!}\right] \\ $$

Commented by mr W last updated on 27/Sep/20

!n is the number of derangements from n elements.

$$!{n}\:{is}\:{the}\:{number}\:{of}\:{derangements} \\ $$$${from}\:{n}\:{elements}. \\ $$

Commented by PRITHWISH SEN 2 last updated on 22/Sep/20

sir please check Q 111906

$$\mathrm{sir}\:\mathrm{please}\:\mathrm{check}\:\mathrm{Q}\:\mathrm{111906} \\ $$

Answered by soumyasaha last updated on 22/Sep/20

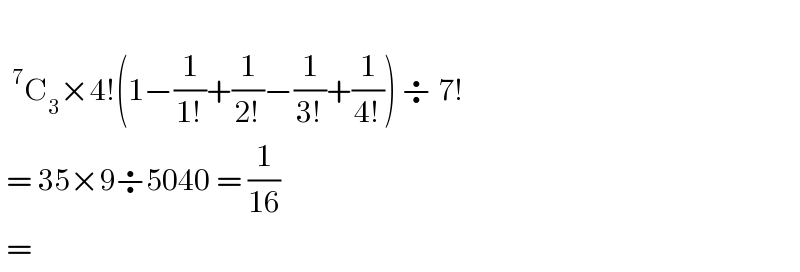
^7 C_3 ×4!(1−(1/(1!))+(1/(2!))−(1/(3!))+(1/(4!))) ÷ 7! = 35×9÷5040 = (1/(16)) =

$$ \\ $$$$\:\:^{\mathrm{7}} \mathrm{C}_{\mathrm{3}} ×\mathrm{4}!\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{1}!}+\frac{\mathrm{1}}{\mathrm{2}!}−\frac{\mathrm{1}}{\mathrm{3}!}+\frac{\mathrm{1}}{\mathrm{4}!}\right)\:\boldsymbol{\div}\:\mathrm{7}! \\ $$$$\:=\:\mathrm{35}×\mathrm{9}\boldsymbol{\div}\mathrm{5040}\:=\:\frac{\mathrm{1}}{\mathrm{16}} \\ $$$$\:=\: \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com