Question and Answers Forum

All Questions      Topic List

Mechanics Questions

Previous in All Question      Next in All Question      

Previous in Mechanics      Next in Mechanics      

Question Number 26450 by Tinkutara last updated on 25/Dec/17

A man of mass M is standing on a platform of mass m_1 holding a string passing over a system of ideal pulleys. Another mass m_2 is hanging as shown (m_2 = 20 kg, m_1 = 10 kg, g = 10 m/s^2 ) Force exerted by man on string to accelerate upwards

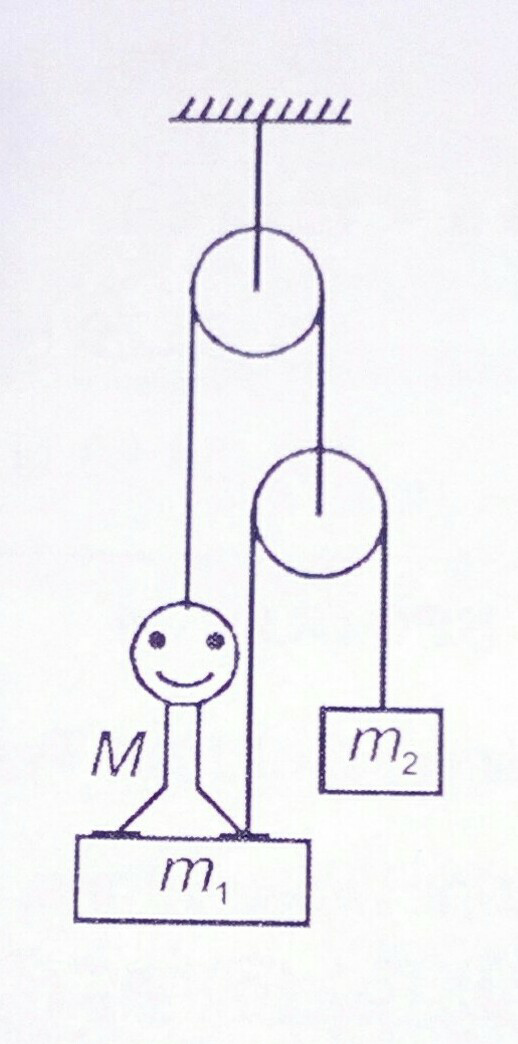
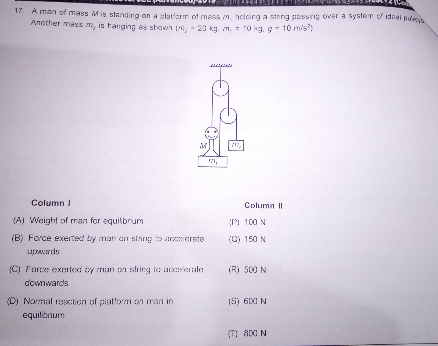
$$\mathrm{A}\:\mathrm{man}\:\mathrm{of}\:\mathrm{mass}\:{M}\:\mathrm{is}\:\mathrm{standing}\:\mathrm{on}\:\mathrm{a} \\ $$$$\mathrm{platform}\:\mathrm{of}\:\mathrm{mass}\:{m}_{\mathrm{1}} \:\mathrm{holding}\:\mathrm{a}\:\mathrm{string} \\ $$$$\mathrm{passing}\:\mathrm{over}\:\mathrm{a}\:\mathrm{system}\:\mathrm{of}\:\mathrm{ideal}\:\mathrm{pulleys}. \\ $$$$\mathrm{Another}\:\mathrm{mass}\:{m}_{\mathrm{2}} \:\mathrm{is}\:\mathrm{hanging}\:\mathrm{as}\:\mathrm{shown} \\ $$$$\left({m}_{\mathrm{2}} \:=\:\mathrm{20}\:\mathrm{kg},\:{m}_{\mathrm{1}} \:=\:\mathrm{10}\:\mathrm{kg},\:{g}\:=\:\mathrm{10}\:\mathrm{m}/\mathrm{s}^{\mathrm{2}} \right) \\ $$$$\mathrm{Force}\:\mathrm{exerted}\:\mathrm{by}\:\mathrm{man}\:\mathrm{on}\:\mathrm{string}\:\mathrm{to} \\ $$$$\mathrm{accelerate}\:\mathrm{upwards} \\ $$

Commented by Tinkutara last updated on 25/Dec/17

Commented by mrW1 last updated on 26/Dec/17

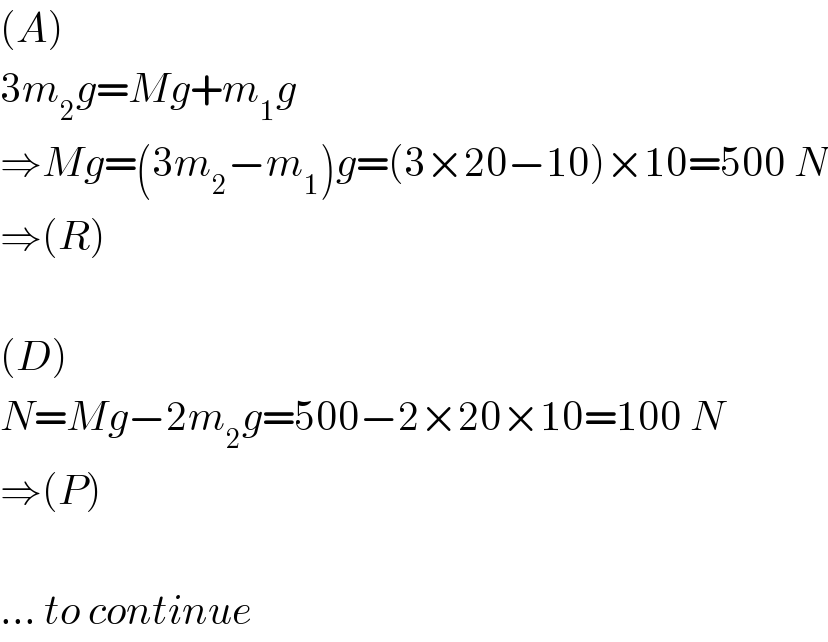
(A) 3m_2 g=Mg+m_1 g ⇒Mg=(3m_2 −m_1 )g=(3×20−10)×10=500 N ⇒(R) (D) N=Mg−2m_2 g=500−2×20×10=100 N ⇒(P) ... to continue

$$\left({A}\right) \\ $$$$\mathrm{3}{m}_{\mathrm{2}} {g}={Mg}+{m}_{\mathrm{1}} {g} \\ $$$$\Rightarrow{Mg}=\left(\mathrm{3}{m}_{\mathrm{2}} −{m}_{\mathrm{1}} \right){g}=\left(\mathrm{3}×\mathrm{20}−\mathrm{10}\right)×\mathrm{10}=\mathrm{500}\:{N} \\ $$$$\Rightarrow\left({R}\right) \\ $$$$ \\ $$$$\left({D}\right) \\ $$$${N}={Mg}−\mathrm{2}{m}_{\mathrm{2}} {g}=\mathrm{500}−\mathrm{2}×\mathrm{20}×\mathrm{10}=\mathrm{100}\:{N} \\ $$$$\Rightarrow\left({P}\right) \\ $$$$ \\ $$$$...\:{to}\:{continue} \\ $$

Commented by Tinkutara last updated on 26/Dec/17

Commented by Tinkutara last updated on 26/Dec/17

Above was the full question. It is a Matrix matching columns. If the image is not clear see it at: http://ibb.co/mVtvPm

Commented by mrW1 last updated on 26/Dec/17

thanks! now it′s clear.

$${thanks}!\:{now}\:{it}'{s}\:{clear}. \\ $$

Answered by ajfour last updated on 26/Dec/17

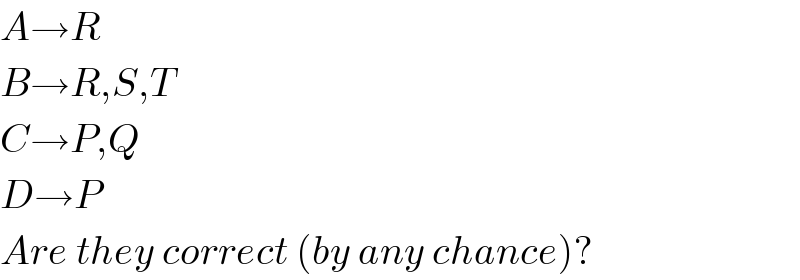
A→R B→R,S,T C→P,Q D→P Are they correct (by any chance)?

$${A}\rightarrow{R}\:\: \\ $$$${B}\rightarrow{R},{S},{T} \\ $$$${C}\rightarrow{P},{Q} \\ $$$${D}\rightarrow{P} \\ $$$${Are}\:{they}\:{correct}\:\left({by}\:{any}\:{chance}\right)? \\ $$

Answered by ajfour last updated on 26/Dec/17

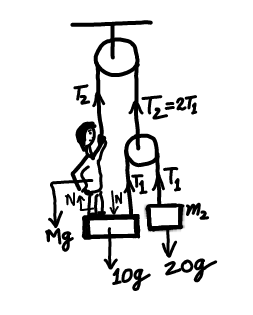
Commented by Tinkutara last updated on 27/Dec/17

So force exerted by man should be N? Right?

Commented by Tinkutara last updated on 27/Dec/17

But N and F are different or not?

Commented by ajfour last updated on 27/Dec/17

under equilibrium of platform T_1 =(T_2 /2)=(F/2)=N+m_1 g so N and F are different.

$${under}\:{equilibrium}\:{of}\:{platform} \\ $$$${T}_{\mathrm{1}} =\frac{{T}_{\mathrm{2}} }{\mathrm{2}}=\frac{{F}}{\mathrm{2}}={N}+{m}_{\mathrm{1}} {g} \\ $$$${so}\:{N}\:{and}\:{F}\:{are}\:{different}. \\ $$

Commented by ajfour last updated on 27/Dec/17

why makes you arrive at this conclusion, do explain.

Commented by Tinkutara last updated on 27/Dec/17

In B question it is asking about force exerted by man on string so it should be the normal reaction that man can exert on string because string is under his feet.

Commented by ajfour last updated on 26/Dec/17

(case I)system boundary includes man and platform: 3T_1 −(M+10)g=0 ...(i) and also T_1 =20g (in equilibrium) ⇒M=50kg ; W=Mg=500 newtons ⇒ A→R when in slight acceleration(↑) F=2T_1 20g−(F/2)> 0 ⇒ F > 40g ⇒ hence B→ R,S,T and for slight acceleration (↓) F < 40g and C→P,Q forces on platform(in equilibrium) T_1 −10g−N =0 or (F/2)−10g−N = 0 ⇒ N= 200−100 =100 newtons so D→P .

$$\:\left({case}\:{I}\right){system}\:{boundary}\:{includes} \\ $$$${man}\:{and}\:{platform}: \\ $$$$\mathrm{3}{T}_{\mathrm{1}} −\left({M}+\mathrm{10}\right){g}=\mathrm{0}\:\:\:\:...\left({i}\right) \\ $$$${and}\:\:{also}\:\:{T}_{\mathrm{1}} =\mathrm{20}{g}\:\:\:\left({in}\:{equilibrium}\right) \\ $$$$\Rightarrow{M}=\mathrm{50}{kg}\:;\:{W}={Mg}=\mathrm{500}\:{newtons} \\ $$$$\Rightarrow\:\:{A}\rightarrow{R} \\ $$$${when}\:{in}\:{slight}\:{acceleration}\left(\uparrow\right) \\ $$$${F}=\mathrm{2}{T}_{\mathrm{1}} \\ $$$$\mathrm{20}{g}−\frac{{F}}{\mathrm{2}}>\:\mathrm{0}\:\:\:\Rightarrow\:\:{F}\:>\:\mathrm{40}{g}\: \\ $$$$\Rightarrow\:\:{hence}\:{B}\rightarrow\:\:{R},{S},{T} \\ $$$${and}\:{for}\:{slight}\:{acceleration}\:\left(\downarrow\right) \\ $$$${F}\:<\:\mathrm{40}{g} \\ $$$${and}\:\:{C}\rightarrow{P},{Q} \\ $$$${forces}\:{on}\:{platform}\left({in}\:{equilibrium}\right) \\ $$$$\:{T}_{\mathrm{1}} −\mathrm{10}{g}−{N}\:=\mathrm{0} \\ $$$${or}\:\:\frac{{F}}{\mathrm{2}}−\mathrm{10}{g}−{N}\:=\:\mathrm{0} \\ $$$$\Rightarrow\:{N}=\:\mathrm{200}−\mathrm{100}\:=\mathrm{100}\:{newtons} \\ $$$${so}\:\:\:{D}\rightarrow{P}\:. \\ $$

Commented by Tinkutara last updated on 27/Dec/17

You wrote for (B) that F>40g. But force exerted by man on string should be N (normal reaction) instead of F =2T_1 . Please explain.

$${You}\:{wrote}\:{for}\:\left({B}\right)\:{that}\:{F}>\mathrm{40}{g}.\:{But} \\ $$$${force}\:{exerted}\:{by}\:{man}\:{on}\:{string}\:{should} \\ $$$${be}\:{N}\:\left({normal}\:{reaction}\right)\:{instead}\:{of}\:{F} \\ $$$$=\mathrm{2}{T}_{\mathrm{1}} .\:{Please}\:{explain}. \\ $$

Commented by ajfour last updated on 27/Dec/17

F=T_2 =2T_1 (Action↔Reaction)

$${F}={T}_{\mathrm{2}} =\mathrm{2}{T}_{\mathrm{1}} \:\:\left({Action}\leftrightarrow{Reaction}\right) \\ $$

Commented by ajfour last updated on 27/Dec/17

no string under his feet, that string is attached to platform m_1 . The question must be asking for the string he must be holding in hand, which in diagram (not correctly drawn) has been attached to his head. so F=T_2 ≠N .

$${no}\:{string}\:{under}\:{his}\:{feet},\:{that}\: \\ $$$${string}\:{is}\:{attached}\:{to}\:{platform} \\ $$$${m}_{\mathrm{1}} .\:{The}\:{question}\:{must}\:{be}\:{asking} \\ $$$${for}\:{the}\:{string}\:{he}\:{must}\:{be}\:{holding} \\ $$$${in}\:{hand},\:{which}\:{in}\:{diagram}\:\left({not}\right. \\ $$$$\left.{correctly}\:{drawn}\right)\:{has}\:{been}\:{attached} \\ $$$${to}\:{his}\:{head}.\:{so}\:{F}={T}_{\mathrm{2}} \:\neq{N}\:. \\ $$

Commented by Tinkutara last updated on 27/Dec/17

Thank you very much Sir! I got the answer.

Terms of Service

Privacy Policy

Contact: info@tinkutara.com