
Question Number 98983 by M±th+et+s last updated on 17/Jun/20
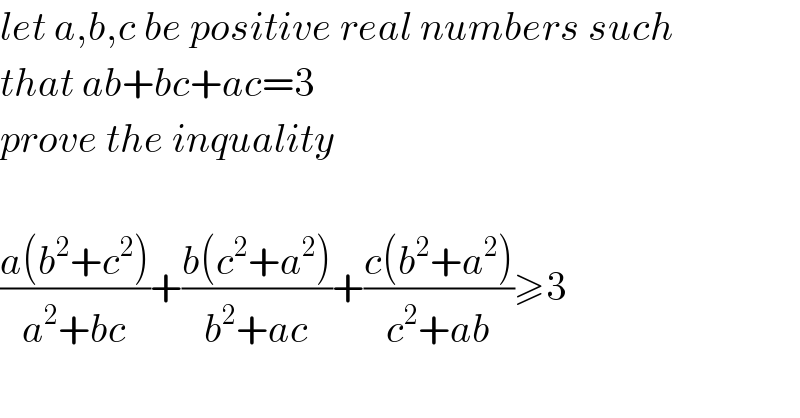
$${let}\:{a},{b},{c}\:{be}\:{positive}\:{real}\:{numbers}\:{such} \\ $$$${that}\:{ab}+{bc}+{ac}=\mathrm{3}\: \\ $$$${prove}\:{the}\:{inquality} \\ $$$$ \\ $$$$\frac{{a}\left({b}^{\mathrm{2}} +{c}^{\mathrm{2}} \right)}{{a}^{\mathrm{2}} +{bc}}+\frac{{b}\left({c}^{\mathrm{2}} +{a}^{\mathrm{2}} \right)}{{b}^{\mathrm{2}} +{ac}}+\frac{{c}\left({b}^{\mathrm{2}} +{a}^{\mathrm{2}} \right)}{{c}^{\mathrm{2}} +{ab}}\geqslant\mathrm{3} \\ $$
Commented by MJS last updated on 17/Jun/20

$$\mathrm{due}\:\mathrm{to}\:\mathrm{symmetry}\:\mathrm{extremes}\:\mathrm{at}\:{a}={b}={c}\:\Rightarrow \\ $$$${ab}+{bc}+{ac}=\mathrm{3}\:\Leftrightarrow\:\mathrm{3}{a}^{\mathrm{2}} =\mathrm{3}\:\Rightarrow\:{a}=\pm\mathrm{1} \\ $$$$\mathrm{but}\:{a}>\mathrm{0}\:\Rightarrow\:{a}={b}={c}=\mathrm{1} \\ $$$$\mathrm{the}\:\mathrm{inequation}\:\mathrm{with}\:{a}={b}={c}\:\mathrm{turns}\:\mathrm{into} \\ $$$$\mathrm{3}{a}\geqslant\mathrm{3}\:\Rightarrow\:\mathrm{true}\:\mathrm{for}\:{a}=\mathrm{1} \\ $$$$\mathrm{now}\:\mathrm{test}\:\mathrm{if}\:\mathrm{this}\:\mathrm{is}\:\mathrm{min}\:\mathrm{or}\:\mathrm{max}\:\mathrm{by}\:\mathrm{putting} \\ $$$${a}=.\mathrm{999};\:{b}=\mathrm{1}.\mathrm{001}\:\Rightarrow\:{c}=\mathrm{1}.\mathrm{0000005} \\ $$$$\Rightarrow\:\mathrm{lhs}\:>\mathrm{3} \\ $$$$\Rightarrow\:\mathrm{proven} \\ $$$$\mathrm{I}\:\mathrm{know}\:\mathrm{you}\:\mathrm{want}\:\mathrm{a}\:\mathrm{different}\:\mathrm{kind}\:\mathrm{of}\:\mathrm{proof} \\ $$$$\mathrm{but}\:\mathrm{this}\:\mathrm{is}\:\mathrm{the}\:\mathrm{easiest}\:\mathrm{path} \\ $$
Commented by M±th+et+s last updated on 17/Jun/20

$${this}\:{is}\:{a}\:{good}\:{proof}\:{thank}\:{you}\: \\ $$
