
Question Number 97361 by student work last updated on 07/Jun/20

$$\int\frac{\mathrm{xdx}}{\mathrm{sin}\:^{\mathrm{2}} \mathrm{x}−\mathrm{3}}=? \\ $$
Commented by student work last updated on 07/Jun/20

$$\mathrm{who}\:\mathrm{is}\:\mathrm{intellagent}? \\ $$
Commented by mr W last updated on 07/Jun/20

$${i}\:{don}'{t}\:{know}\:{who}\:{is}\:{intelligent}. \\ $$$${but}\:{according}\:{to}\:{this}\:{question}\:{of}\:{you}, \\ $$$${i}\:{know}\:{at}\:{least}\:{who}\:{is}\:{not}\:{intelligent}. \\ $$
Commented by som(math1967) last updated on 07/Jun/20
����
Commented by student work last updated on 07/Jun/20

$$\mathrm{solve} \\ $$
Commented by student work last updated on 07/Jun/20

$$\mathrm{who}\:\mathrm{can}? \\ $$
Commented by Tinku Tara last updated on 07/Jun/20

$$\mathrm{Hi}\:\mathrm{student}\:\mathrm{work} \\ $$$$\mathrm{please}\:\mathrm{stop}\:\mathrm{putting}\:\mathrm{comments} \\ $$$$\mathrm{such}\:\mathrm{as}\:\mathrm{who}\:\mathrm{is}\:\mathrm{intelligent},\:\mathrm{solve} \\ $$$$\mathrm{who}\:\mathrm{can}\:\mathrm{etc}. \\ $$$$\mathrm{Polite}\:\mathrm{comments}\:\mathrm{like}\:\mathrm{pls}\:\mathrm{help}, \\ $$$$\mathrm{i}\:\mathrm{need}\:\mathrm{help}\:\mathrm{for}\:\mathrm{assignment} \\ $$$$\mathrm{are}\:\mathrm{fine}. \\ $$
Answered by mathmax by abdo last updated on 07/Jun/20
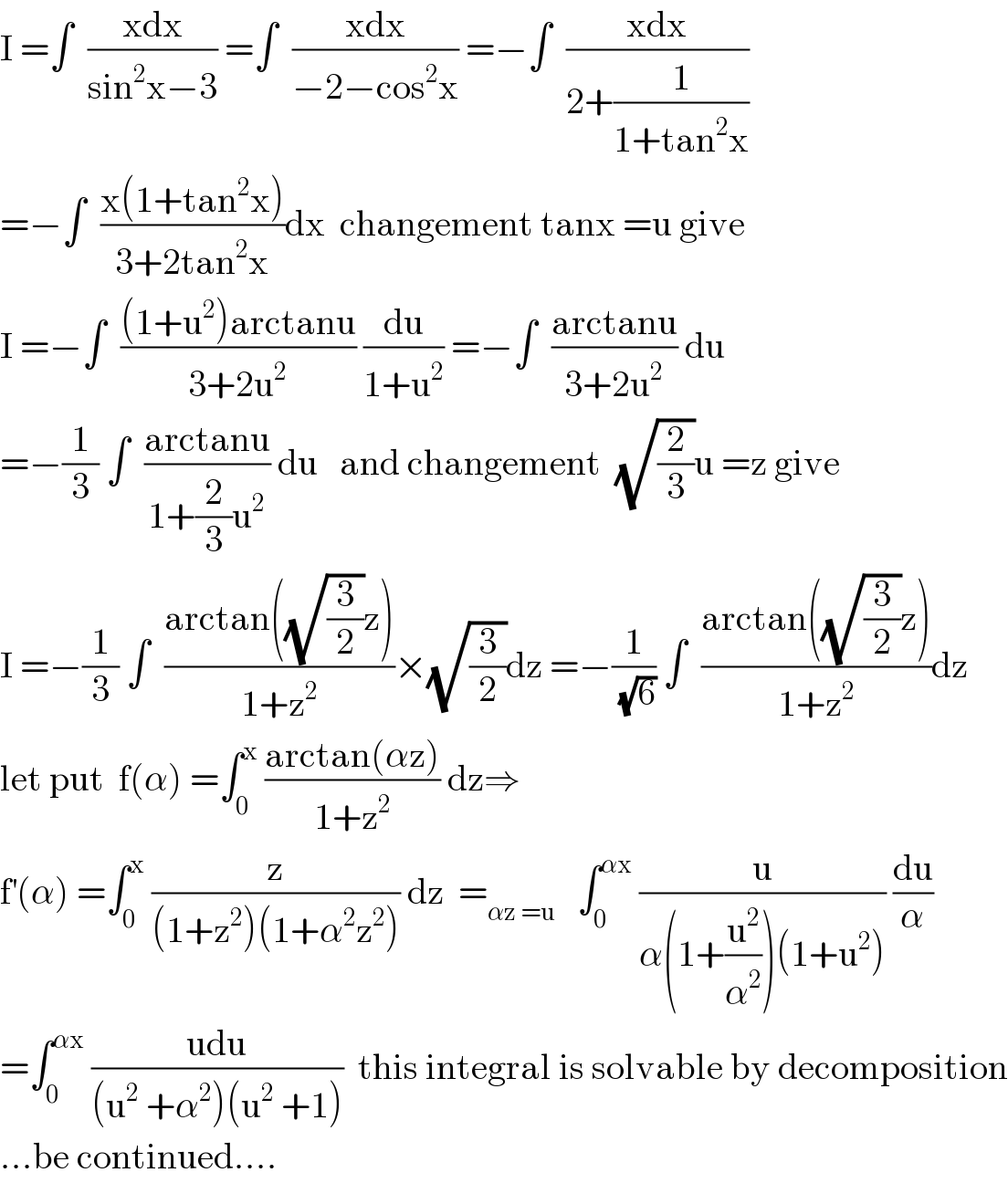
$$\mathrm{I}\:=\int\:\:\frac{\mathrm{xdx}}{\mathrm{sin}^{\mathrm{2}} \mathrm{x}−\mathrm{3}}\:=\int\:\:\frac{\mathrm{xdx}}{−\mathrm{2}−\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\:=−\int\:\:\frac{\mathrm{xdx}}{\mathrm{2}+\frac{\mathrm{1}}{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \mathrm{x}}} \\ $$$$=−\int\:\:\frac{\mathrm{x}\left(\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \mathrm{x}\right)}{\mathrm{3}+\mathrm{2tan}^{\mathrm{2}} \mathrm{x}}\mathrm{dx}\:\:\mathrm{changement}\:\mathrm{tanx}\:=\mathrm{u}\:\mathrm{give} \\ $$$$\mathrm{I}\:=−\int\:\:\frac{\left(\mathrm{1}+\mathrm{u}^{\mathrm{2}} \right)\mathrm{arctanu}}{\mathrm{3}+\mathrm{2u}^{\mathrm{2}} }\:\frac{\mathrm{du}}{\mathrm{1}+\mathrm{u}^{\mathrm{2}} }\:=−\int\:\:\frac{\mathrm{arctanu}}{\mathrm{3}+\mathrm{2u}^{\mathrm{2}} }\:\mathrm{du} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{3}}\:\int\:\:\frac{\mathrm{arctanu}}{\mathrm{1}+\frac{\mathrm{2}}{\mathrm{3}}\mathrm{u}^{\mathrm{2}} }\:\mathrm{du}\:\:\:\mathrm{and}\:\mathrm{changement}\:\:\sqrt{\frac{\mathrm{2}}{\mathrm{3}}}\mathrm{u}\:=\mathrm{z}\:\mathrm{give} \\ $$$$\mathrm{I}\:=−\frac{\mathrm{1}}{\mathrm{3}}\:\int\:\:\frac{\mathrm{arctan}\left(\sqrt{\frac{\mathrm{3}}{\mathrm{2}}}\mathrm{z}\right)}{\mathrm{1}+\mathrm{z}^{\mathrm{2}} }×\sqrt{\frac{\mathrm{3}}{\mathrm{2}}}\mathrm{dz}\:=−\frac{\mathrm{1}}{\sqrt{\mathrm{6}}}\:\int\:\:\frac{\mathrm{arctan}\left(\sqrt{\frac{\mathrm{3}}{\mathrm{2}}}\mathrm{z}\right)}{\mathrm{1}+\mathrm{z}^{\mathrm{2}} }\mathrm{dz} \\ $$$$\mathrm{let}\:\mathrm{put}\:\:\mathrm{f}\left(\alpha\right)\:=\int_{\mathrm{0}} ^{\mathrm{x}} \:\frac{\mathrm{arctan}\left(\alpha\mathrm{z}\right)}{\mathrm{1}+\mathrm{z}^{\mathrm{2}} }\:\mathrm{dz}\Rightarrow \\ $$$$\mathrm{f}^{'} \left(\alpha\right)\:=\int_{\mathrm{0}} ^{\mathrm{x}} \:\frac{\mathrm{z}}{\left(\mathrm{1}+\mathrm{z}^{\mathrm{2}} \right)\left(\mathrm{1}+\alpha^{\mathrm{2}} \mathrm{z}^{\mathrm{2}} \right)}\:\mathrm{dz}\:\:=_{\alpha\mathrm{z}\:=\mathrm{u}} \:\:\:\int_{\mathrm{0}} ^{\alpha\mathrm{x}} \:\frac{\mathrm{u}}{\alpha\left(\mathrm{1}+\frac{\mathrm{u}^{\mathrm{2}} }{\alpha^{\mathrm{2}} }\right)\left(\mathrm{1}+\mathrm{u}^{\mathrm{2}} \right)}\:\frac{\mathrm{du}}{\alpha} \\ $$$$=\int_{\mathrm{0}} ^{\alpha\mathrm{x}} \:\frac{\mathrm{udu}}{\left(\mathrm{u}^{\mathrm{2}} \:+\alpha^{\mathrm{2}} \right)\left(\mathrm{u}^{\mathrm{2}} \:+\mathrm{1}\right)}\:\:\mathrm{this}\:\mathrm{integral}\:\mathrm{is}\:\mathrm{solvable}\:\mathrm{by}\:\mathrm{decomposition} \\ $$$$...\mathrm{be}\:\mathrm{continued}.... \\ $$
