
Previous in Probability and Statistics Next in Probability and Statistics
Question Number 96730 by Ar Brandon last updated on 04/Jun/20

$$\:\:\:\:\:\:\mathcal{A}\:\mathrm{third}\:\mathrm{of}\:\mathrm{a}\:\mathrm{population}\:\mathrm{has}\:\mathrm{been}\:\mathrm{vaccined}\:\mathrm{against} \\ $$$$\mathrm{an}\:\mathrm{illness}.\:\mathcal{D}\mathrm{uring}\:\mathrm{the}\:\mathrm{pandemie}\:\mathrm{it}\:\mathrm{is}\:\mathrm{noticed}\:\mathrm{that}; \\ $$$$\mathrm{out}\:\mathrm{of}\:\mathrm{15}\:\mathrm{patients},\:\mathrm{2}\:\mathrm{have}\:\mathrm{been}\:\mathrm{vaccined}. \\ $$$$\:\:\:\:\:\:\:\mathcal{A}\mathrm{ssuming}\:\mathrm{that}\:\mathrm{out}\:\mathrm{of}\:\mathrm{a}\:\mathrm{hundred}\:\mathrm{vaccined},\:\mathrm{8}\:\mathrm{are}\:\mathrm{ill}. \\ $$$$\mathcal{A}\mathrm{n}\:\mathrm{individual}\:\mathrm{is}\:\mathrm{choosen}\:\mathrm{at}\:\mathrm{random}\:\mathrm{from}\:\mathrm{this}\:\mathrm{population}. \\ $$$$\mathcal{L}\mathrm{et}\:\boldsymbol{\mathrm{M}}\:\mathrm{imply}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{individual}}\:\boldsymbol{\mathrm{is}}\:\boldsymbol{\mathrm{ill}}\:\mathrm{and}\:\boldsymbol{\mathrm{V}}\:\mathrm{imply}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{individual}} \\ $$$$\boldsymbol{\mathrm{has}}\:\boldsymbol{\mathrm{been}}\:\boldsymbol{\mathrm{vaccined}}. \\ $$$$\mathrm{1}\backslash\:\mathcal{D}\mathrm{etermine}\:\mathrm{the}\:\mathrm{probabilities}:\:\mathrm{P}\left(\mathrm{V}\right);\:\mathrm{P}\left(\mathrm{V}/\mathrm{M}\right)\:\mathrm{and}\:\mathrm{P}\left(\mathrm{M}/\mathrm{V}\right) \\ $$$$\mathrm{2}\backslash\:\mathcal{C}\mathrm{alculate}\:\mathrm{P}\left(\mathrm{M}\cap\mathrm{V}\right)\:\mathrm{then}\:\mathrm{P}\left(\mathrm{M}\right).\:\mathcal{D}\mathrm{educe}\:\mathrm{the} \\ $$$$\mathrm{percentage}\:\mathrm{of}\:\mathrm{patients}. \\ $$
Answered by Rio Michael last updated on 04/Jun/20
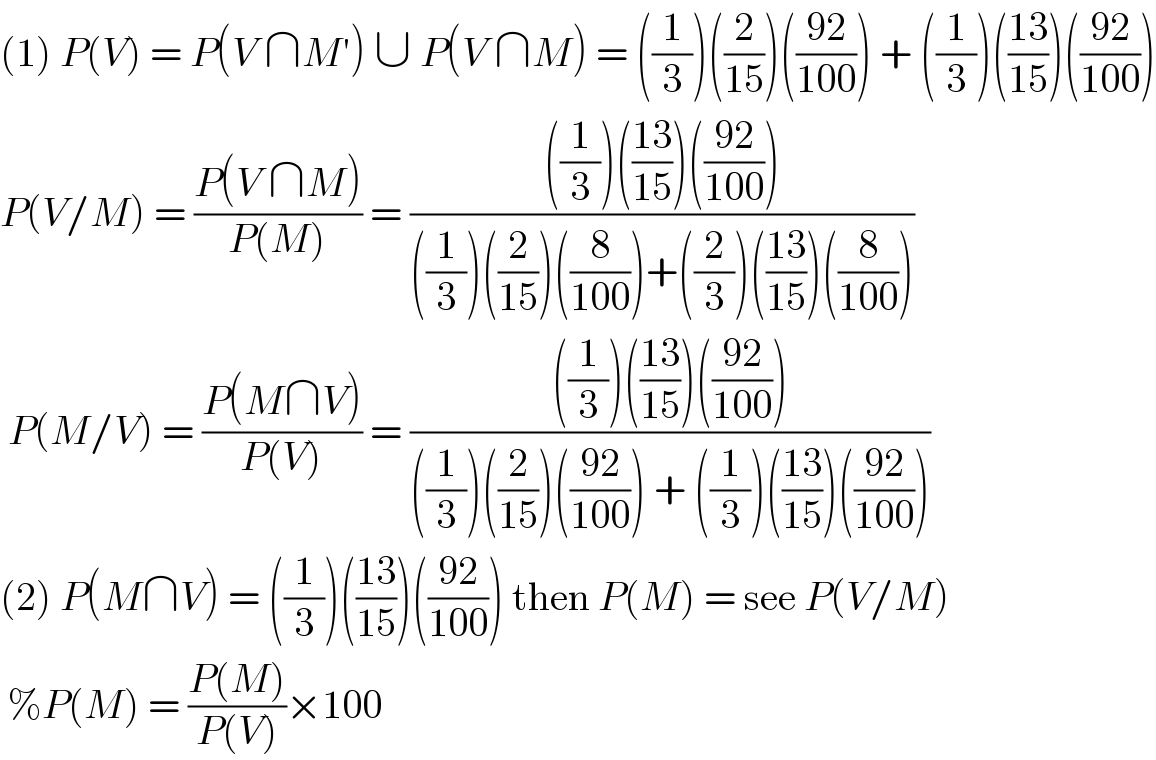
$$\left(\mathrm{1}\right)\:{P}\left({V}\right)\:=\:{P}\left({V}\:\cap{M}'\right)\:\cup\:{P}\left({V}\:\cap{M}\right)\:=\:\left(\frac{\mathrm{1}}{\mathrm{3}}\right)\left(\frac{\mathrm{2}}{\mathrm{15}}\right)\left(\frac{\mathrm{92}}{\mathrm{100}}\right)\:+\:\left(\frac{\mathrm{1}}{\mathrm{3}}\right)\left(\frac{\mathrm{13}}{\mathrm{15}}\right)\left(\frac{\mathrm{92}}{\mathrm{100}}\right) \\ $$$${P}\left({V}/{M}\right)\:=\:\frac{{P}\left({V}\:\cap{M}\right)}{{P}\left({M}\right)}\:=\:\frac{\left(\frac{\mathrm{1}}{\mathrm{3}}\right)\left(\frac{\mathrm{13}}{\mathrm{15}}\right)\left(\frac{\mathrm{92}}{\mathrm{100}}\right)}{\left(\frac{\mathrm{1}}{\mathrm{3}}\right)\left(\frac{\mathrm{2}}{\mathrm{15}}\right)\left(\frac{\mathrm{8}}{\mathrm{100}}\right)+\left(\frac{\mathrm{2}}{\mathrm{3}}\right)\left(\frac{\mathrm{13}}{\mathrm{15}}\right)\left(\frac{\mathrm{8}}{\mathrm{100}}\right)} \\ $$$$\:{P}\left({M}/{V}\right)\:=\:\frac{{P}\left({M}\cap{V}\right)}{{P}\left({V}\right)}\:=\:\frac{\left(\frac{\mathrm{1}}{\mathrm{3}}\right)\left(\frac{\mathrm{13}}{\mathrm{15}}\right)\left(\frac{\mathrm{92}}{\mathrm{100}}\right)}{\left(\frac{\mathrm{1}}{\mathrm{3}}\right)\left(\frac{\mathrm{2}}{\mathrm{15}}\right)\left(\frac{\mathrm{92}}{\mathrm{100}}\right)\:+\:\left(\frac{\mathrm{1}}{\mathrm{3}}\right)\left(\frac{\mathrm{13}}{\mathrm{15}}\right)\left(\frac{\mathrm{92}}{\mathrm{100}}\right)} \\ $$$$\left(\mathrm{2}\right)\:{P}\left({M}\cap{V}\right)\:=\:\left(\frac{\mathrm{1}}{\mathrm{3}}\right)\left(\frac{\mathrm{13}}{\mathrm{15}}\right)\left(\frac{\mathrm{92}}{\mathrm{100}}\right)\:\mathrm{then}\:{P}\left({M}\right)\:=\:\mathrm{see}\:{P}\left({V}/{M}\right) \\ $$$$\:\%{P}\left({M}\right)\:=\:\frac{{P}\left({M}\right)}{{P}\left({V}\right)}×\mathrm{100} \\ $$
Commented by Rio Michael last updated on 04/Jun/20
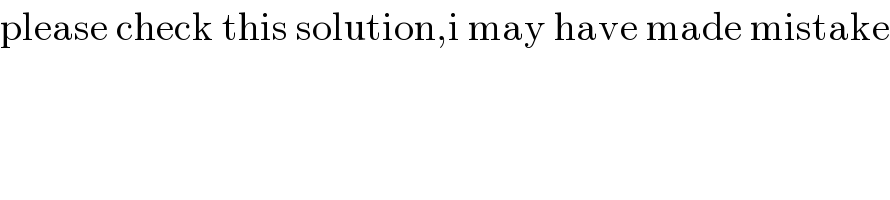
$$\mathrm{please}\:\mathrm{check}\:\mathrm{this}\:\mathrm{solution},\mathrm{i}\:\mathrm{may}\:\mathrm{have}\:\mathrm{made}\:\mathrm{mistake} \\ $$
