
Question Number 96682 by liki last updated on 03/Jun/20
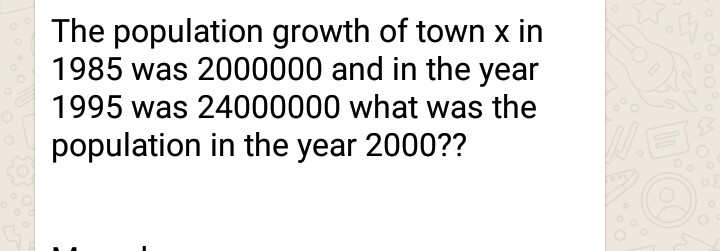
Commented by liki last updated on 03/Jun/20

$$...\mathrm{am}\:\mathrm{i}\:\mathrm{right}\:\mathrm{if}\:\mathrm{use}\:\mathrm{concept}\:\mathrm{of}\:\mathrm{arthmetic}\:\mathrm{progression}?,\mathrm{please}\:\mathrm{if}\:\mathrm{i}\:\mathrm{do}\:\mathrm{mistake}\:\mathrm{need}\:\mathrm{correcton}! \\ $$
Commented by Rio Michael last updated on 03/Jun/20
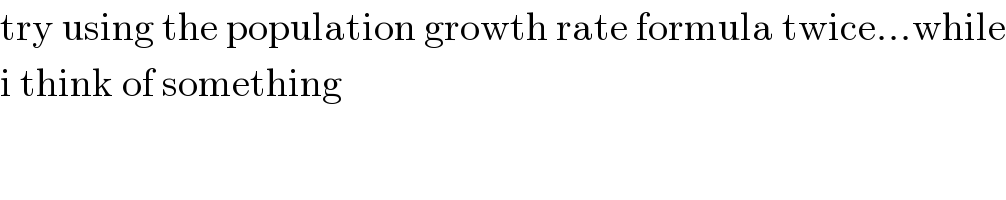
$$\mathrm{try}\:\mathrm{using}\:\mathrm{the}\:\mathrm{population}\:\mathrm{growth}\:\mathrm{rate}\:\mathrm{formula}\:\mathrm{twice}...\mathrm{while} \\ $$$$\mathrm{i}\:\mathrm{think}\:\mathrm{of}\:\mathrm{something} \\ $$
Commented by mr W last updated on 04/Jun/20
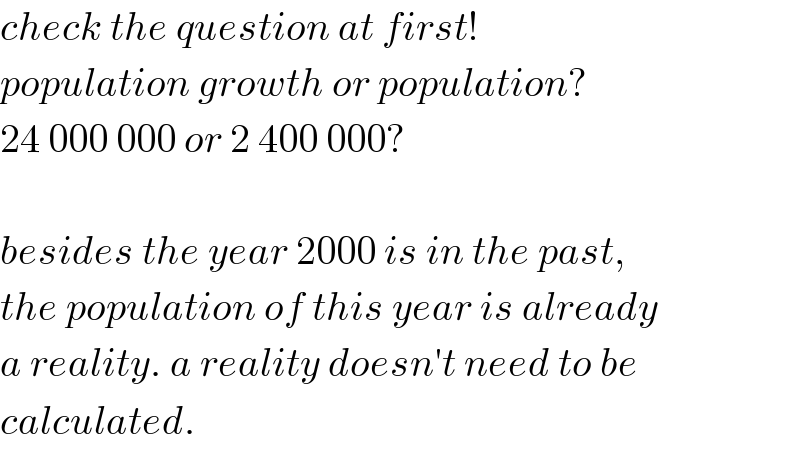
$${check}\:{the}\:{question}\:{at}\:{first}! \\ $$$${population}\:{growth}\:{or}\:{population}? \\ $$$$\mathrm{24}\:\mathrm{000}\:\mathrm{000}\:{or}\:\mathrm{2}\:\mathrm{400}\:\mathrm{000}? \\ $$$$ \\ $$$${besides}\:{the}\:{year}\:\mathrm{2000}\:{is}\:{in}\:{the}\:{past}, \\ $$$${the}\:{population}\:{of}\:{this}\:{year}\:{is}\:{already} \\ $$$${a}\:{reality}.\:{a}\:{reality}\:{doesn}'{t}\:{need}\:{to}\:{be} \\ $$$${calculated}. \\ $$
Commented by Rio Michael last updated on 04/Jun/20
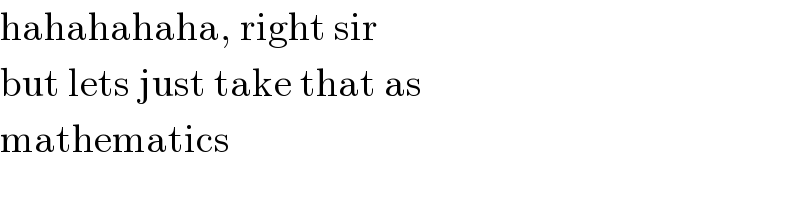
$$\mathrm{hahahahaha},\:\mathrm{right}\:\mathrm{sir} \\ $$$$\mathrm{but}\:\mathrm{lets}\:\mathrm{just}\:\mathrm{take}\:\mathrm{that}\:\mathrm{as} \\ $$$$\mathrm{mathematics} \\ $$
Answered by Rio Michael last updated on 04/Jun/20
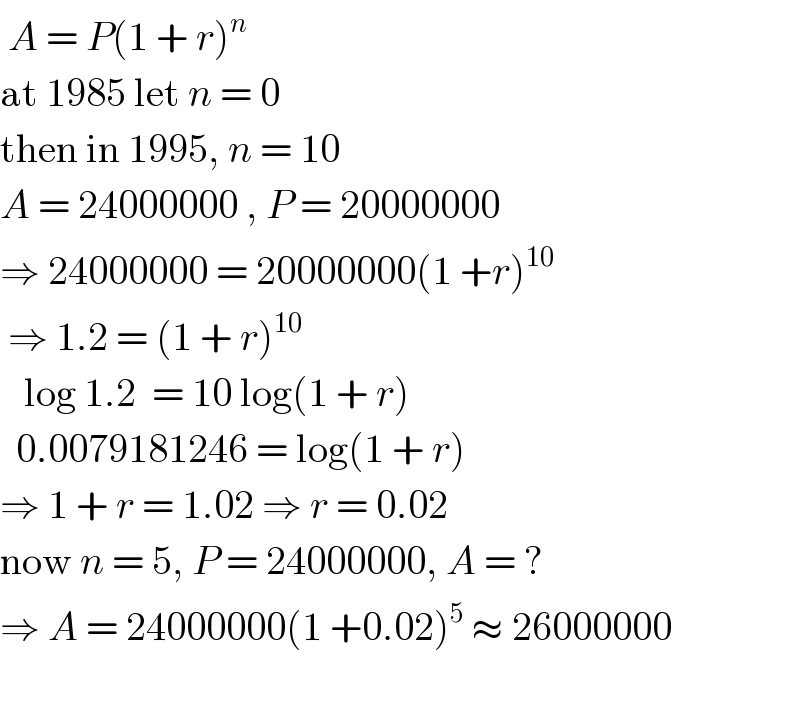
$$\:{A}\:=\:{P}\left(\mathrm{1}\:+\:{r}\right)^{{n}} \\ $$$$\mathrm{at}\:\mathrm{1985}\:\mathrm{let}\:{n}\:=\:\mathrm{0} \\ $$$$\mathrm{then}\:\mathrm{in}\:\mathrm{1995},\:{n}\:=\:\mathrm{10} \\ $$$${A}\:=\:\mathrm{24000000}\:,\:{P}\:=\:\mathrm{20000000} \\ $$$$\Rightarrow\:\mathrm{24000000}\:=\:\mathrm{20000000}\left(\mathrm{1}\:+{r}\right)^{\mathrm{10}} \\ $$$$\:\Rightarrow\:\mathrm{1}.\mathrm{2}\:=\:\left(\mathrm{1}\:+\:{r}\right)^{\mathrm{10}} \\ $$$$\:\:\:\mathrm{log}\:\mathrm{1}.\mathrm{2}\:\:=\:\mathrm{10}\:\mathrm{log}\left(\mathrm{1}\:+\:{r}\right) \\ $$$$\:\:\mathrm{0}.\mathrm{0079181246}\:=\:\mathrm{log}\left(\mathrm{1}\:+\:{r}\right) \\ $$$$\Rightarrow\:\mathrm{1}\:+\:{r}\:=\:\mathrm{1}.\mathrm{02}\:\Rightarrow\:{r}\:=\:\mathrm{0}.\mathrm{02} \\ $$$$\mathrm{now}\:{n}\:=\:\mathrm{5},\:{P}\:=\:\mathrm{24000000},\:{A}\:=\:? \\ $$$$\Rightarrow\:{A}\:=\:\mathrm{24000000}\left(\mathrm{1}\:+\mathrm{0}.\mathrm{02}\right)^{\mathrm{5}} \:\approx\:\mathrm{26000000} \\ $$$$ \\ $$
Commented by mr W last updated on 04/Jun/20
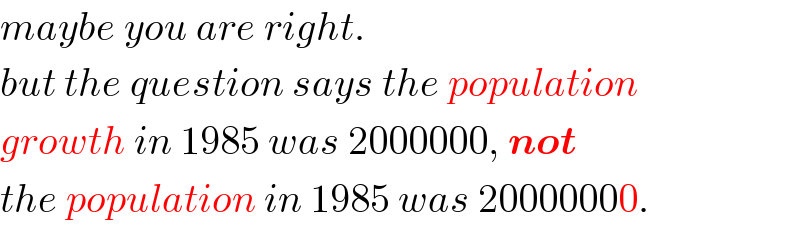
$${maybe}\:{you}\:{are}\:{right}. \\ $$$${but}\:{the}\:{question}\:{says}\:{the}\:{population} \\ $$$${growth}\:{in}\:\mathrm{1985}\:{was}\:\mathrm{2000000},\:\boldsymbol{{not}} \\ $$$${the}\:{population}\:{in}\:\mathrm{1985}\:{was}\:\mathrm{20000000}. \\ $$
Commented by Rio Michael last updated on 04/Jun/20

$$\mathrm{well}\:\mathrm{sir}\:,\:\mathrm{you}\:\mathrm{cannot}\:\mathrm{expect}\:\mathrm{the}\:\mathrm{population} \\ $$$$\mathrm{growth}\:\mathrm{of}\:\mathrm{a}\:\mathrm{country}\:\mathrm{to}\:\mathrm{be}\:\mathrm{20},\mathrm{000},\mathrm{000}\:\mathrm{right} \\ $$$$\mathrm{that}\:\mathrm{is}\:\mathrm{not}\:\mathrm{logical}\:\mathrm{except}\:\mathrm{they}\:\mathrm{are}\:\mathrm{Rabbits} \\ $$$$\mathrm{not}\:\mathrm{humans}. \\ $$$$\mathrm{I}\:\mathrm{assumed}\:\mathrm{the}\:\mathrm{he}/\mathrm{she}\:\mathrm{meant}\:\mathrm{the}\:\mathrm{population} \\ $$$$\mathrm{not}\:\mathrm{population}\:\mathrm{growth}. \\ $$$$\: \\ $$
