
Previous in Relation and Functions Next in Relation and Functions
Question Number 94903 by mathmax by abdo last updated on 21/May/20
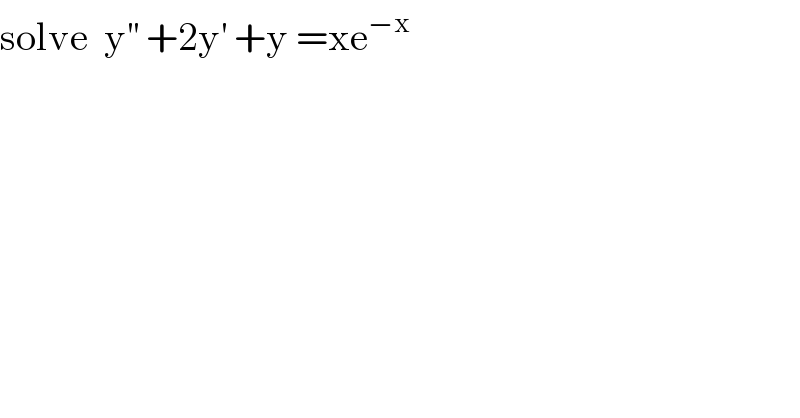
$$\mathrm{solve}\:\:\mathrm{y}^{''} \:+\mathrm{2y}^{'} \:+\mathrm{y}\:=\mathrm{xe}^{−\mathrm{x}} \\ $$
Answered by niroj last updated on 21/May/20
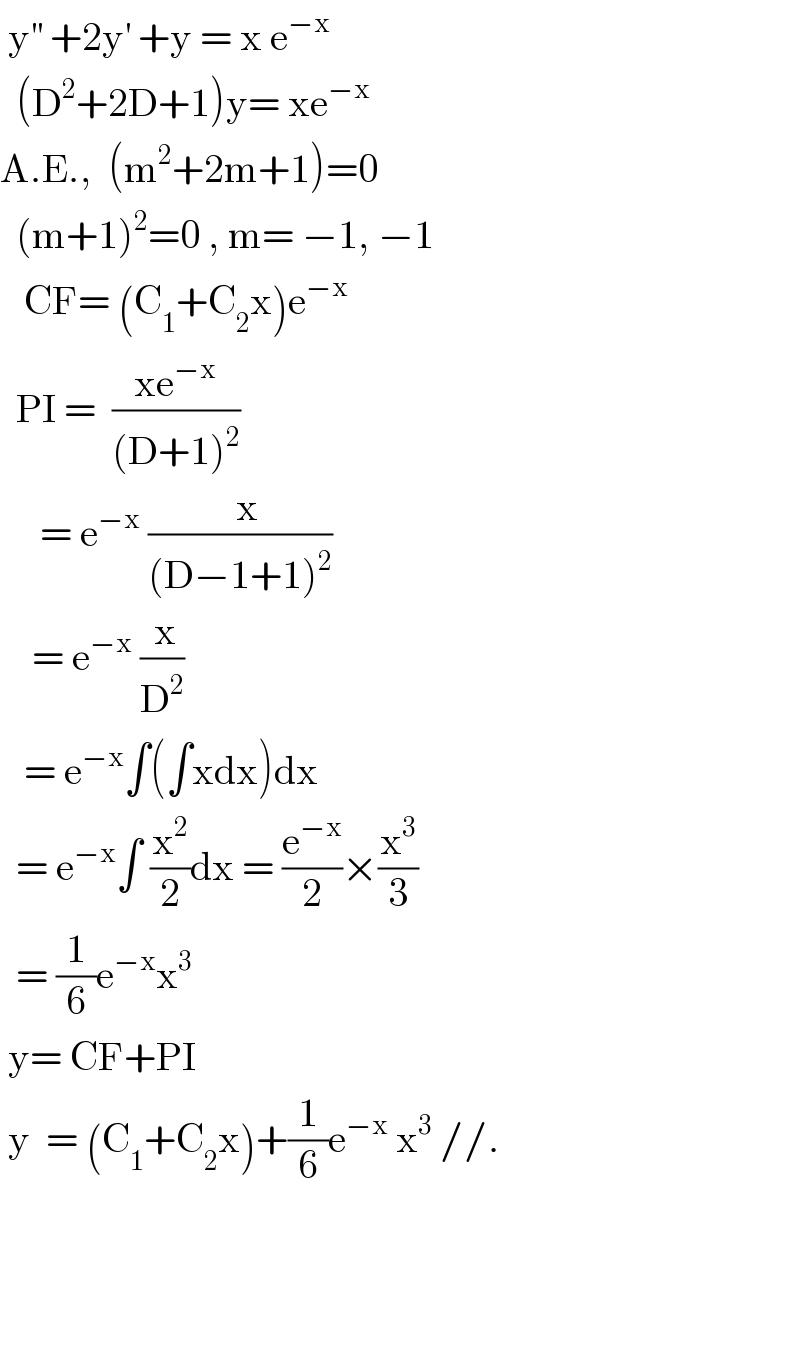
$$\:\mathrm{y}^{''} \:+\mathrm{2y}^{'} \:+\mathrm{y}\:=\:\mathrm{x}\:\mathrm{e}^{−\mathrm{x}} \\ $$$$\:\:\left(\mathrm{D}^{\mathrm{2}} +\mathrm{2D}+\mathrm{1}\right)\mathrm{y}=\:\mathrm{xe}^{−\mathrm{x}} \\ $$$$\mathrm{A}.\mathrm{E}.,\:\:\left(\mathrm{m}^{\mathrm{2}} +\mathrm{2m}+\mathrm{1}\right)=\mathrm{0} \\ $$$$\:\:\left(\mathrm{m}+\mathrm{1}\right)^{\mathrm{2}} =\mathrm{0}\:,\:\mathrm{m}=\:−\mathrm{1},\:−\mathrm{1} \\ $$$$\:\:\:\mathrm{CF}=\:\left(\mathrm{C}_{\mathrm{1}} +\mathrm{C}_{\mathrm{2}} \mathrm{x}\right)\mathrm{e}^{−\mathrm{x}} \\ $$$$\:\:\mathrm{PI}\:=\:\:\frac{\mathrm{xe}^{−\mathrm{x}} }{\left(\mathrm{D}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$\:\:\:\:\:=\:\mathrm{e}^{−\mathrm{x}} \:\frac{\:\:\mathrm{x}}{\left(\mathrm{D}−\mathrm{1}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$\:\:\:\:=\:\mathrm{e}^{−\mathrm{x}} \:\frac{\:\mathrm{x}}{\mathrm{D}^{\mathrm{2}} } \\ $$$$\:\:\:=\:\mathrm{e}^{−\mathrm{x}} \int\left(\int\mathrm{xdx}\right)\mathrm{dx} \\ $$$$\:\:=\:\mathrm{e}^{−\mathrm{x}} \int\:\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}\mathrm{dx}\:=\:\frac{\mathrm{e}^{−\mathrm{x}} }{\mathrm{2}}×\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}} \\ $$$$\:\:=\:\frac{\mathrm{1}}{\mathrm{6}}\mathrm{e}^{−\mathrm{x}} \mathrm{x}^{\mathrm{3}} \\ $$$$\:\mathrm{y}=\:\mathrm{CF}+\mathrm{PI} \\ $$$$\:\mathrm{y}\:\:=\:\left(\mathrm{C}_{\mathrm{1}} +\mathrm{C}_{\mathrm{2}} \mathrm{x}\right)+\frac{\mathrm{1}}{\mathrm{6}}\mathrm{e}^{−\mathrm{x}} \:\mathrm{x}^{\mathrm{3}} \://. \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by mathmax by abdo last updated on 22/May/20

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir}. \\ $$
Commented by niroj last updated on 22/May/20
��
Answered by ElOuafi last updated on 22/May/20

$${let}\:\left({E}\right):\:{y}''+\mathrm{2}{y}'+{y}={xe}^{−{x}} \\ $$$${and}\:\left({E}_{\mathrm{0}} \right):{y}''+\mathrm{2}{y}'+{y}=\mathrm{0}\:{be}\:{its}\:{equation}\:{without}\: \\ $$$${second}\:{member}.\Rightarrow\left({E}_{{r}} \right):{r}^{\mathrm{2}} +\mathrm{2}{r}+\mathrm{1}=\mathrm{0}\Rightarrow{r}=−\mathrm{1} \\ $$$${so}\:{y}_{\mathrm{1}} ={e}^{−{x}} \wedge\:{y}_{\mathrm{2}} ={xe}^{−{x}} \:{are}\:{solutions}\:{of}\:\left({E}_{\mathrm{0}} \right) \\ $$$${the}\:{general}\:{solution}\:{of}\:\left({E}_{\mathrm{0}} \right)\:{is}:\:{y}_{{g}_{\mathrm{0}} } =\lambda_{\mathrm{1}} {e}^{−{x}} +\lambda_{\mathrm{2}} {xe}^{−{x}} \\ $$$${with}\:\lambda_{\mathrm{1}} ,\lambda_{\mathrm{2}} \in\mathbb{R}\:,\:{let}\:{search}\:{now}\:{a}\:{particular}\:{solution} \\ $$$${of}\:\left({E}\right)\:{under}\:{the}\:{forme}:\:{y}_{{p}} =\lambda_{\mathrm{1}} \left({x}\right){y}_{\mathrm{1}} +\lambda_{\mathrm{2}} \left({x}\right){y}_{\mathrm{2}} \: \\ $$$${witch}\:\lambda_{\mathrm{1}} \left({x}\right),\lambda_{\mathrm{2}} \left({x}\right)\:{satisfying}\: \\ $$$$\lambda_{\mathrm{1}} ^{'} \left({x}\right){e}^{−{x}} +\lambda_{\mathrm{2}} ^{'} \left({x}\right).{xe}^{−{x}} =\mathrm{0}\wedge\:\lambda_{\mathrm{1}} ^{'} \left({x}\right).\left({e}^{−{x}} \right)^{'} +\lambda_{\mathrm{2}} ^{'} \left({x}\right).\left({xe}^{−{x}} \right)'={xe}^{−{x}} \\ $$$${after}\:{solving}\:{system}\:{we}\:{find}:\:\lambda_{\mathrm{1}} ^{'} \left({x}\right)=−{x}^{\mathrm{2}} \wedge\:\lambda_{\mathrm{2}} ^{'} \left({x}\right)={x} \\ $$$$\Rightarrow\lambda_{\mathrm{1}} =−\frac{{x}^{\mathrm{3}} }{\mathrm{3}}\:\wedge\:\lambda_{\mathrm{2}} =\frac{{x}^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\Rightarrow{y}_{{p}} =−\frac{{x}^{\mathrm{3}} }{\mathrm{3}}{y}_{\mathrm{1}} +\frac{{x}^{\mathrm{2}} }{\mathrm{2}}{y}_{\mathrm{2}} \Leftrightarrow{y}_{{p}} =−\frac{{x}^{\mathrm{3}} }{\mathrm{3}}{e}^{−{x}} +\frac{{x}^{\mathrm{3}} }{\mathrm{2}}{e}^{−{x}} =\frac{{x}^{\mathrm{3}} }{\mathrm{6}}{e}^{−{x}} \\ $$$${the}\:{general}\:{solution}\:{of}\:\left({E}\right)\:{is}\:: \\ $$$${y}_{{g}} ={y}_{{p}} +{y}_{{g}_{\mathrm{0}} } \\ $$$${y}\left({x}\right)=\frac{{x}^{\mathrm{3}} }{\mathrm{6}}{e}^{−{x}} +\lambda_{\mathrm{1}} {e}^{−{x}} +\lambda_{\mathrm{2}} {xe}^{−{x}} \:;\left(\lambda_{\mathrm{1}} ;\lambda_{\mathrm{2}} \right)\in\mathbb{R}^{\mathrm{2}} \\ $$
Commented by mathmax by abdo last updated on 22/May/20

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir} \\ $$
