
Previous in Relation and Functions Next in Relation and Functions
Question Number 94382 by frc2crc last updated on 18/May/20

$${a}_{{n}+\mathrm{1}} =\sqrt{{k}+\sqrt{{a}_{{n}} }}\:\:\:\:{a}_{\mathrm{0}} =\sqrt{{k}} \\ $$$${how}\:{do}\:{you}\:{solve}\:{for}\:{k}? \\ $$$${Only}\:{Equation}\:{please}\:{no}\:{value} \\ $$$${for}\:{k} \\ $$
Commented by prakash jain last updated on 18/May/20

$$\mathrm{I}\:\mathrm{dont}\:\mathrm{think}\:\mathrm{it}\:\mathrm{possible}\:\mathrm{to}\:\mathrm{solve}\:\mathrm{for}\:{k}. \\ $$$$ \\ $$$$\mathrm{Every}\:\mathrm{value}\:\mathrm{of}\:{k}\:\mathrm{defines}\:\mathrm{a}\:\mathrm{new} \\ $$$$\mathrm{sequence}. \\ $$$$ \\ $$$$\mathrm{For}\:\mathrm{example} \\ $$$${a}_{{n}+\mathrm{1}} =\sqrt{\mathrm{1}+\sqrt{\mathrm{1}}}\:{a}_{\mathrm{0}} =\mathrm{1}\:\mathrm{is}\:\mathrm{one}\:\mathrm{sequence} \\ $$$${a}_{{n}+\mathrm{1}} =\sqrt{\mathrm{2}+\sqrt{\mathrm{2}}}\:{a}_{\mathrm{0}} =\mathrm{2}\:\mathrm{is}\:\mathrm{another}\:\mathrm{sequence} \\ $$$$\mathrm{You}\:\mathrm{need}\:\mathrm{to}\:\mathrm{have}\:\mathrm{at}\:\mathrm{least}\:\mathrm{one} \\ $$$$\mathrm{more}\:\mathrm{constraint}\:\mathrm{on}\:{a}_{{n}} \:\mathrm{to}\:\mathrm{be}\:\mathrm{able} \\ $$$$\mathrm{to}\:\mathrm{solve}\:\mathrm{for}\:{k}. \\ $$
Commented by mr W last updated on 18/May/20
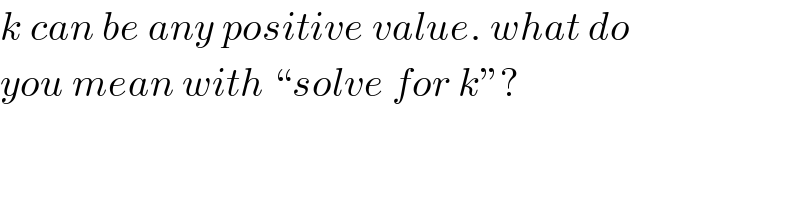
$${k}\:{can}\:{be}\:{any}\:{positive}\:{value}.\:{what}\:{do} \\ $$$${you}\:{mean}\:{with}\:``{solve}\:{for}\:{k}''? \\ $$
Answered by mathmax by abdo last updated on 20/May/20
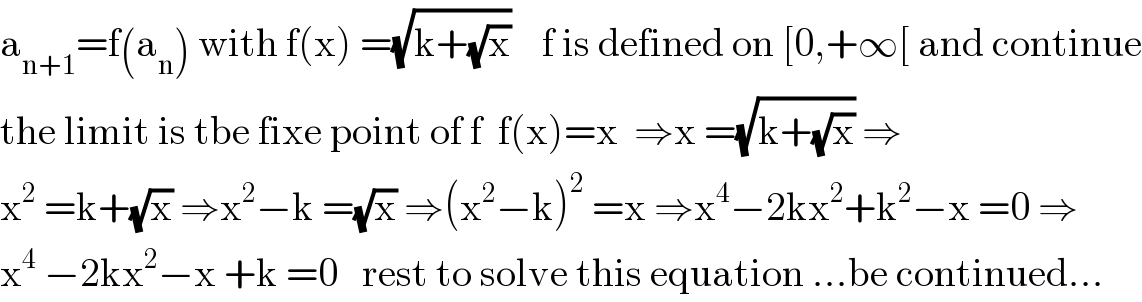
$$\mathrm{a}_{\mathrm{n}+\mathrm{1}} =\mathrm{f}\left(\mathrm{a}_{\mathrm{n}} \right)\:\mathrm{with}\:\mathrm{f}\left(\mathrm{x}\right)\:=\sqrt{\mathrm{k}+\sqrt{\mathrm{x}}}\:\:\:\:\mathrm{f}\:\mathrm{is}\:\mathrm{defined}\:\mathrm{on}\:\left[\mathrm{0},+\infty\left[\:\mathrm{and}\:\mathrm{continue}\right.\right. \\ $$$$\mathrm{the}\:\mathrm{limit}\:\mathrm{is}\:\mathrm{tbe}\:\mathrm{fixe}\:\mathrm{point}\:\mathrm{of}\:\mathrm{f}\:\:\mathrm{f}\left(\mathrm{x}\right)=\mathrm{x}\:\:\Rightarrow\mathrm{x}\:=\sqrt{\mathrm{k}+\sqrt{\mathrm{x}}}\:\Rightarrow \\ $$$$\mathrm{x}^{\mathrm{2}} \:=\mathrm{k}+\sqrt{\mathrm{x}}\:\Rightarrow\mathrm{x}^{\mathrm{2}} −\mathrm{k}\:=\sqrt{\mathrm{x}}\:\Rightarrow\left(\mathrm{x}^{\mathrm{2}} −\mathrm{k}\right)^{\mathrm{2}} \:=\mathrm{x}\:\Rightarrow\mathrm{x}^{\mathrm{4}} −\mathrm{2kx}^{\mathrm{2}} +\mathrm{k}^{\mathrm{2}} −\mathrm{x}\:=\mathrm{0}\:\Rightarrow \\ $$$$\mathrm{x}^{\mathrm{4}} \:−\mathrm{2kx}^{\mathrm{2}} −\mathrm{x}\:+\mathrm{k}\:=\mathrm{0}\:\:\:\mathrm{rest}\:\mathrm{to}\:\mathrm{solve}\:\mathrm{this}\:\mathrm{equation}\:...\mathrm{be}\:\mathrm{continued}... \\ $$
