
Previous in Differential Equation Next in Differential Equation
Question Number 91825 by john santu last updated on 03/May/20
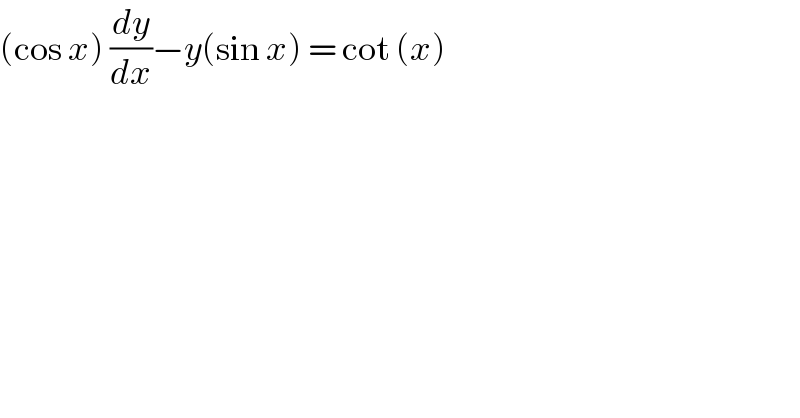
$$\left(\mathrm{cos}\:{x}\right)\:\frac{{dy}}{{dx}}−{y}\left(\mathrm{sin}\:{x}\right)\:=\:\mathrm{cot}\:\left({x}\right) \\ $$
Commented by Tony Lin last updated on 03/May/20
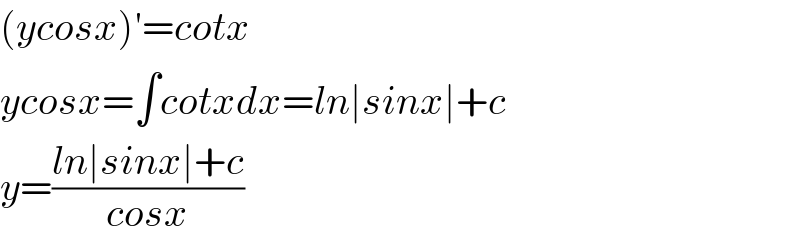
$$\left({ycosx}\right)'={cotx} \\ $$$${ycosx}=\int{cotxdx}={ln}\mid{sinx}\mid+{c} \\ $$$${y}=\frac{{ln}\mid{sinx}\mid+{c}}{{cosx}} \\ $$
Commented by john santu last updated on 03/May/20

$$\mathrm{thank}\:\mathrm{you} \\ $$
Commented by mathmax by abdo last updated on 04/May/20

$${cosx}\:{y}^{'} −{sinx}\:{y}\:={cotanx} \\ $$$$\left({he}\right)\:\rightarrow{cosx}\:{y}^{'} \:−{sinx}\:{y}\:=\mathrm{0}\:\Rightarrow{cosx}\:{y}^{'} \:={sinx}\:{y}\:\Rightarrow \\ $$$$\frac{{y}^{'} }{{y}}\:=\frac{{sinx}}{{cosx}}\:\Rightarrow{ln}\mid{y}\mid\:=−{ln}\left({cosx}\right)+{c}\:\Rightarrow{y}\:=\frac{{k}}{{cosx}} \\ $$$${let}\:{use}\:{mvc}\:{method}\:\:\rightarrow{y}^{'} \:=\frac{{k}^{'} }{{cosx}}+{k}\frac{{sinx}}{{cos}^{\mathrm{2}} {x}} \\ $$$$\left({e}\right)\Rightarrow{k}^{'} +{k}\:\frac{{sinx}}{{cosx}}−\frac{{ksinx}}{{cosx}}\:=\frac{\mathrm{1}}{{tanx}}\:\Rightarrow{k}^{'} \:=\frac{{cosx}}{{sinx}}\:\Rightarrow \\ $$$${k}\:=\int\:\:\frac{{cosx}}{{sinx}}{dx}\:+{c}\:\Rightarrow{k}\left({x}\right)={ln}\left({sinx}\right)\:+{c}\:\Rightarrow \\ $$$${the}\:{general}\:{solution}\:{is}\:{y}\left({x}\right)=\frac{\mathrm{1}}{{cosx}}\left({ln}\left({sinx}\right)\:+{c}\right) \\ $$$$=\frac{{ln}\left({sinx}\right)}{{cosx}}\:+\frac{{c}}{{cosx}} \\ $$
Answered by john santu last updated on 03/May/20
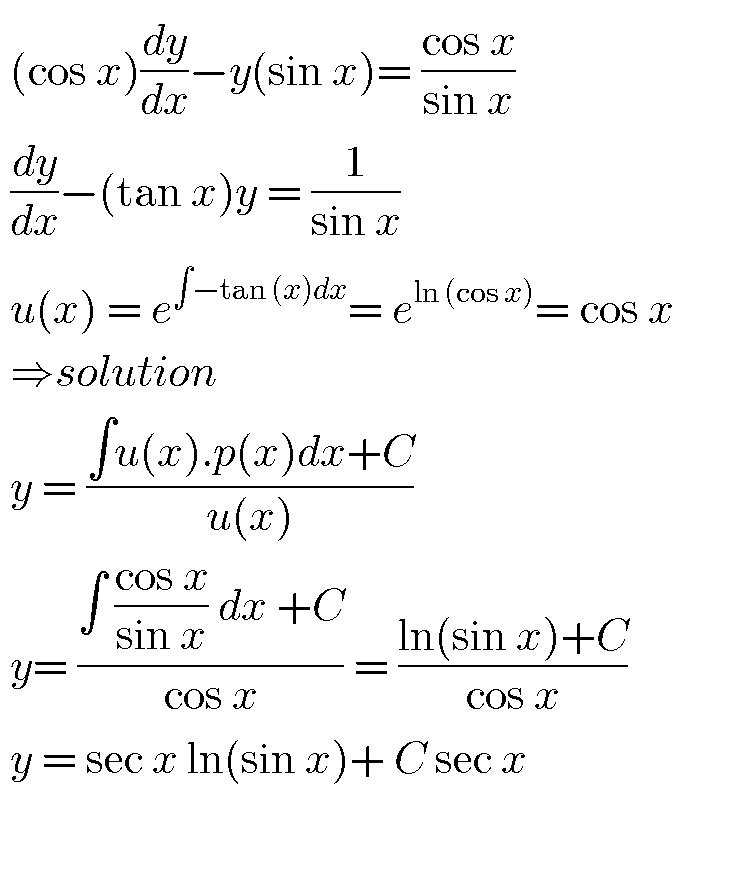
Commented by john santu last updated on 03/May/20
������
Answered by niroj last updated on 03/May/20
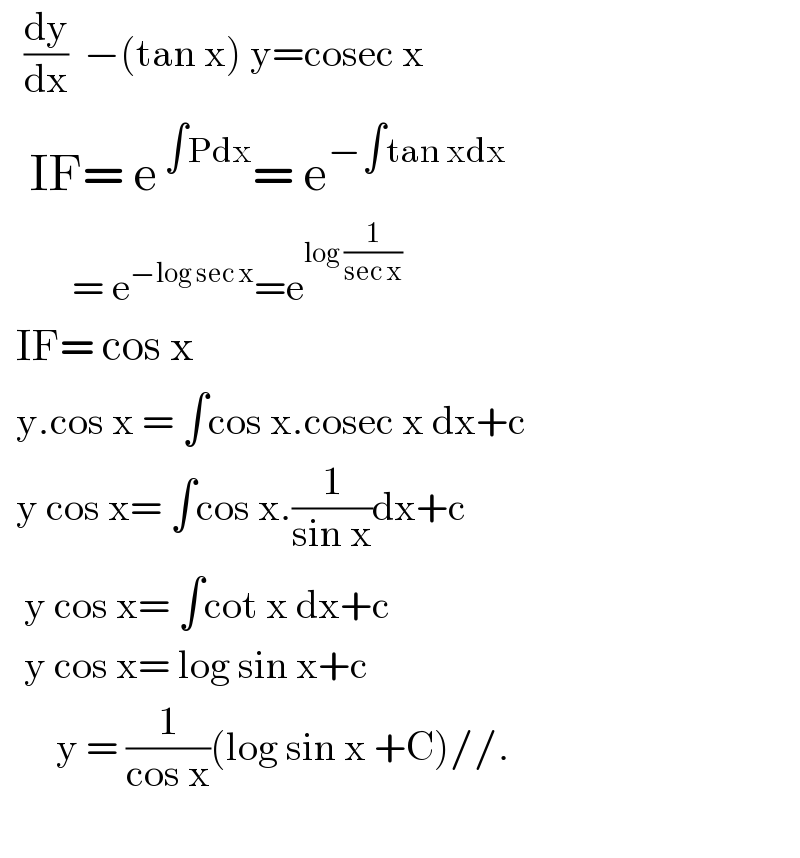
$$\:\:\:\frac{\mathrm{dy}}{\mathrm{dx}}\:\:−\left(\mathrm{tan}\:\mathrm{x}\right)\:\mathrm{y}=\mathrm{cosec}\:\mathrm{x} \\ $$$$\:\:\:\mathrm{IF}=\:\mathrm{e}^{\:\int\mathrm{Pdx}} =\:\mathrm{e}^{−\int\mathrm{tan}\:\mathrm{xdx}} \\ $$$$\:\:\:\:\:\:\:\:\:=\:\mathrm{e}^{−\mathrm{log}\:\mathrm{sec}\:\mathrm{x}} =\mathrm{e}^{\mathrm{log}\:\frac{\mathrm{1}}{\mathrm{sec}\:\mathrm{x}}} \\ $$$$\:\:\mathrm{IF}=\:\mathrm{cos}\:\mathrm{x} \\ $$$$\:\:\mathrm{y}.\mathrm{cos}\:\mathrm{x}\:=\:\int\mathrm{cos}\:\mathrm{x}.\mathrm{cosec}\:\mathrm{x}\:\mathrm{dx}+\mathrm{c} \\ $$$$\:\:\mathrm{y}\:\mathrm{cos}\:\mathrm{x}=\:\int\mathrm{cos}\:\mathrm{x}.\frac{\mathrm{1}}{\mathrm{sin}\:\mathrm{x}}\mathrm{dx}+\mathrm{c} \\ $$$$\:\:\:\mathrm{y}\:\mathrm{cos}\:\mathrm{x}=\:\int\mathrm{cot}\:\mathrm{x}\:\mathrm{dx}+\mathrm{c} \\ $$$$\:\:\:\mathrm{y}\:\mathrm{cos}\:\mathrm{x}=\:\mathrm{log}\:\mathrm{sin}\:\mathrm{x}+\mathrm{c} \\ $$$$\:\:\:\:\:\:\:\mathrm{y}\:=\:\frac{\mathrm{1}}{\mathrm{cos}\:\mathrm{x}}\left(\mathrm{log}\:\mathrm{sin}\:\mathrm{x}\:+\mathrm{C}\right)//. \\ $$$$ \\ $$
Commented by john santu last updated on 03/May/20
����
