
Question Number 89300 by I want to learn more last updated on 16/Apr/20

$$. \\ $$
Commented by I want to learn more last updated on 16/Apr/20
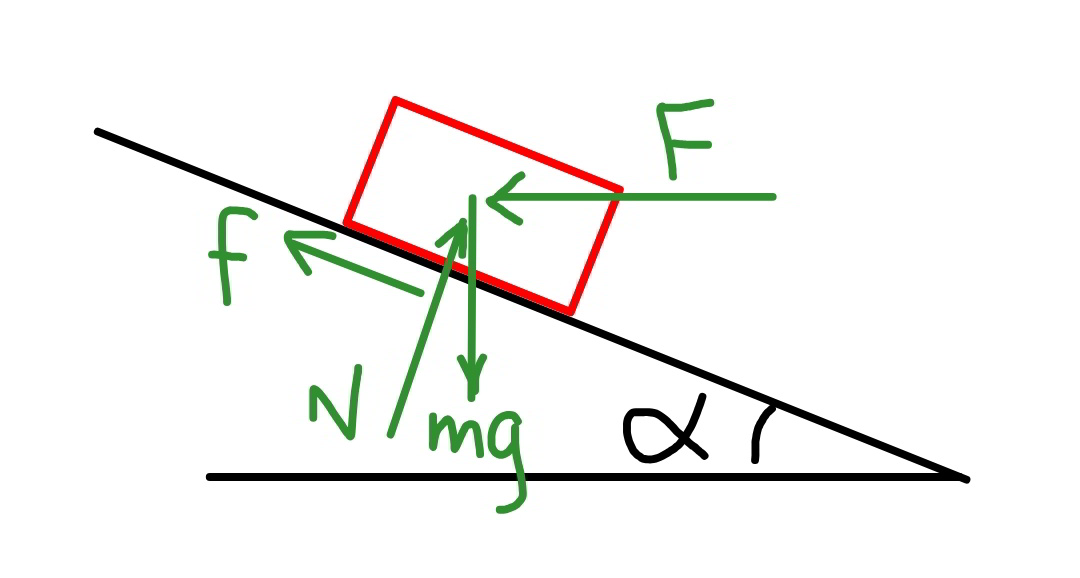
Determine the minimum horizontal force required to maintain a 10kg box on a ramp inclined at 30° to the horizontal if the coefficient of friction is 0.2
Commented by mr W last updated on 17/Apr/20
Commented by mr W last updated on 17/Apr/20
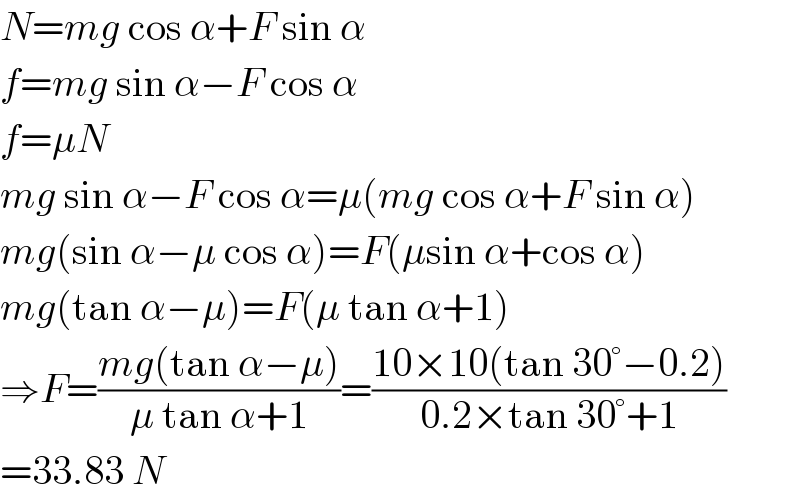
$${N}={mg}\:\mathrm{cos}\:\alpha+{F}\:\mathrm{sin}\:\alpha \\ $$$${f}={mg}\:\mathrm{sin}\:\alpha−{F}\:\mathrm{cos}\:\alpha \\ $$$${f}=\mu{N} \\ $$$${mg}\:\mathrm{sin}\:\alpha−{F}\:\mathrm{cos}\:\alpha=\mu\left({mg}\:\mathrm{cos}\:\alpha+{F}\:\mathrm{sin}\:\alpha\right) \\ $$$${mg}\left(\mathrm{sin}\:\alpha−\mu\:\mathrm{cos}\:\alpha\right)={F}\left(\mu\mathrm{sin}\:\alpha+\mathrm{cos}\:\alpha\right) \\ $$$${mg}\left(\mathrm{tan}\:\alpha−\mu\right)={F}\left(\mu\:\mathrm{tan}\:\alpha+\mathrm{1}\right) \\ $$$$\Rightarrow{F}=\frac{{mg}\left(\mathrm{tan}\:\alpha−\mu\right)}{\mu\:\mathrm{tan}\:\alpha+\mathrm{1}}=\frac{\mathrm{10}×\mathrm{10}\left(\mathrm{tan}\:\mathrm{30}°−\mathrm{0}.\mathrm{2}\right)}{\mathrm{0}.\mathrm{2}×\mathrm{tan}\:\mathrm{30}°+\mathrm{1}} \\ $$$$=\mathrm{33}.\mathrm{83}\:{N} \\ $$
Commented by I want to learn more last updated on 17/Apr/20
$$\mathrm{Thanks}\:\mathrm{sir}.\:\mathrm{I}\:\mathrm{appreciate}. \\ $$
Answered by 242242864 last updated on 17/Apr/20

$$\boldsymbol{{H}}{orizontal}\:{force}\:=\:\boldsymbol{{F}} \\ $$$$\boldsymbol{{R}}{esolving}\:{along}\:{the}\:{plane} \\ $$$$\boldsymbol{{F}}\mathrm{cos}\:\propto+\boldsymbol{{uR}}=\boldsymbol{{mg}}\mathrm{sin}\:\propto.......\left(\boldsymbol{{i}}\right) \\ $$$$\boldsymbol{{V}}{ertically}\:{the}\:{plane}, \\ $$$$\boldsymbol{{F}}\mathrm{sin}\:\propto+\boldsymbol{{mg}}\mathrm{cos}\:\propto=\boldsymbol{{R}} \\ $$$$\Rightarrow\boldsymbol{{F}}\mathrm{cos}\:\propto+\boldsymbol{{u}}\left(\boldsymbol{{F}}\mathrm{sin}\:\propto+\boldsymbol{{mg}}\mathrm{cos}\:\propto\right)=\boldsymbol{{mg}}\mathrm{sin}\:\propto \\ $$$${but}\:\propto=\mathrm{30}^{°} \\ $$$$\boldsymbol{{F}}\mathrm{cos}\:\mathrm{30}^{°} +\mathrm{0}.\mathrm{2}\left(\boldsymbol{{F}}\mathrm{sin}\:\mathrm{30}^{°} +\mathrm{10}×\mathrm{9}.\mathrm{8cos}\:\mathrm{30}°\right)=\mathrm{10}×\mathrm{9}.\mathrm{8sin}\:\mathrm{30}^{°} \\ $$$$\mathrm{0}.\mathrm{87}\boldsymbol{{F}}+\mathrm{0}.\mathrm{1}\boldsymbol{{F}}+\mathrm{16}.\mathrm{97}=\mathrm{49} \\ $$$$\boldsymbol{{F}}=\:\frac{\mathrm{32}.\mathrm{03}}{\mathrm{0}.\mathrm{97}} \\ $$$$\boldsymbol{{F}}=\:\mathrm{33}.\mathrm{02}\boldsymbol{{N}} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by I want to learn more last updated on 17/Apr/20
$$\mathrm{Thanks}\:\mathrm{sir},\:\mathrm{i}\:\mathrm{appreciate} \\ $$
