
Previous in Permutation and Combination Next in Permutation and Combination
Question Number 87175 by john santu last updated on 03/Apr/20
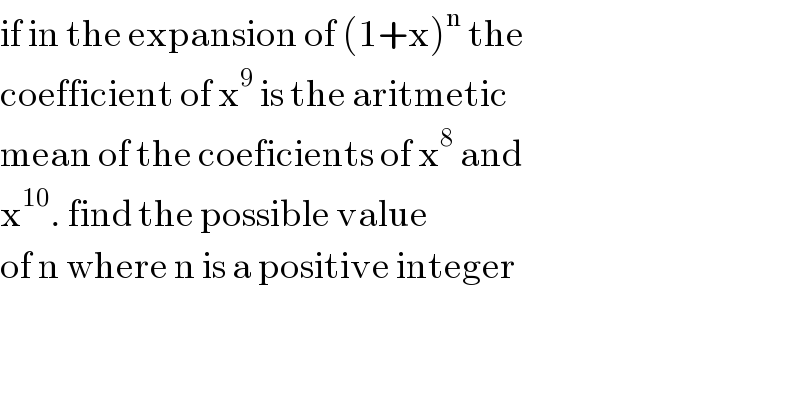
$$\mathrm{if}\:\mathrm{in}\:\mathrm{the}\:\mathrm{expansion}\:\mathrm{of}\:\left(\mathrm{1}+\mathrm{x}\right)^{\mathrm{n}} \:\mathrm{the} \\ $$$$\mathrm{coefficient}\:\mathrm{of}\:\mathrm{x}^{\mathrm{9}} \:\mathrm{is}\:\mathrm{the}\:\mathrm{aritmetic}\: \\ $$$$\mathrm{mean}\:\mathrm{of}\:\mathrm{the}\:\mathrm{coeficients}\:\mathrm{of}\:\mathrm{x}^{\mathrm{8}} \:\mathrm{and}\: \\ $$$$\mathrm{x}^{\mathrm{10}} .\:\mathrm{find}\:\mathrm{the}\:\mathrm{possible}\:\mathrm{value}\: \\ $$$$\mathrm{of}\:\mathrm{n}\:\mathrm{where}\:\mathrm{n}\:\mathrm{is}\:\mathrm{a}\:\mathrm{positive}\:\mathrm{integer} \\ $$
Commented by jagoll last updated on 03/Apr/20

$$\mathrm{i}\:\mathrm{can}\:\mathrm{try}\: \\ $$$$\mathrm{2}\:\mathrm{C}_{\mathrm{9}} ^{\mathrm{n}} \:=\:\mathrm{C}_{\mathrm{8}} ^{\mathrm{n}} \:+\:\mathrm{C}_{\mathrm{10}} ^{\mathrm{n}} \\ $$$$\mathrm{2}×\frac{\mathrm{n}!}{\mathrm{9}!\:\left(\mathrm{n}−\mathrm{9}\right)!}\:=\:\frac{\mathrm{n}!}{\mathrm{8}!\:\left(\mathrm{n}−\mathrm{8}\right)!}\:+\:\frac{\mathrm{n}!}{\mathrm{10}!\:\left(\mathrm{n}−\mathrm{10}\right)!} \\ $$$$\frac{\mathrm{2}}{\mathrm{9}\left(\mathrm{n}−\mathrm{9}\right)}\:=\:\frac{\mathrm{1}}{\mathrm{1}}+\:\frac{\mathrm{1}}{\mathrm{10}.\mathrm{9}.\left(\mathrm{n}−\mathrm{10}\right)\left(\mathrm{n}−\mathrm{9}\right)} \\ $$$$\frac{\mathrm{20}\left(\mathrm{n}−\mathrm{10}\right)−\mathrm{1}}{\mathrm{10}.\mathrm{9}.\left(\mathrm{n}−\mathrm{9}\right)\left(\mathrm{n}−\mathrm{10}\right)}\:=\:\mathrm{1} \\ $$$$\mathrm{20n}\:−\mathrm{201}\:=\:\mathrm{90}\left(\mathrm{n}^{\mathrm{2}} −\mathrm{19n}+\mathrm{90}\right) \\ $$$$\mathrm{90n}^{\mathrm{2}} −\mathrm{1690}\:\mathrm{n}\:+\:\mathrm{8301}\:=\:\mathrm{0} \\ $$
Commented by john santu last updated on 03/Apr/20

$$\mathrm{good}\:\mathrm{sir} \\ $$
Answered by john santu last updated on 03/Apr/20

$$\mathrm{2C}_{\mathrm{9}} ^{\mathrm{n}} \:=\:\mathrm{C}_{\mathrm{8}} ^{\mathrm{n}} \:+\:\mathrm{C}_{\mathrm{10}} ^{\mathrm{n}} \\ $$$$\mathrm{4C}_{\mathrm{9}} ^{\mathrm{n}} \:=\:\left(\mathrm{C}_{\mathrm{8}} ^{\mathrm{n}} \:+\:\mathrm{C}_{\mathrm{9}} ^{\mathrm{n}} \right)\:+\:\left(\:\mathrm{C}_{\mathrm{9}} ^{\mathrm{n}} \:+\:\mathrm{C}_{\mathrm{10}} ^{\mathrm{n}} \right)\: \\ $$$$\mathrm{4C}_{\mathrm{9}} ^{\mathrm{n}} \:=\:\mathrm{C}_{\mathrm{9}} ^{\mathrm{n}+\mathrm{1}} \:+\:\mathrm{C}_{\mathrm{10}} ^{\mathrm{n}+\mathrm{1}} \:=\:\mathrm{C}_{\mathrm{10}} ^{\mathrm{n}+\mathrm{2}} \\ $$$$\frac{\mathrm{4n}!}{\mathrm{9}!\:\left(\mathrm{n}−\mathrm{9}\right)!}\:=\:\frac{\left(\mathrm{n}+\mathrm{2}\right)!}{\mathrm{10}!\:\left(\mathrm{n}−\mathrm{8}\right)!} \\ $$$$\mathrm{4}\:=\:\frac{\mathrm{n}^{\mathrm{2}} +\mathrm{3n}+\mathrm{2}}{\mathrm{10}\left(\mathrm{n}−\mathrm{8}\right)}\:\Rightarrow\:\mathrm{n}^{\mathrm{2}} −\mathrm{37n}\:+\mathrm{322}\:=\:\mathrm{0} \\ $$$$\left(\mathrm{n}−\mathrm{14}\right)\left(\mathrm{n}−\mathrm{23}\right)\:=\:\mathrm{0}\: \\ $$$$\mathrm{n}\:=\:\mathrm{14}\:\mathrm{or}\:\mathrm{n}\:=\:\mathrm{23}\: \\ $$$$ \\ $$
Commented by peter frank last updated on 03/Apr/20

$${good}\: \\ $$
