
Question Number 81432 by abdomathmax last updated on 13/Feb/20
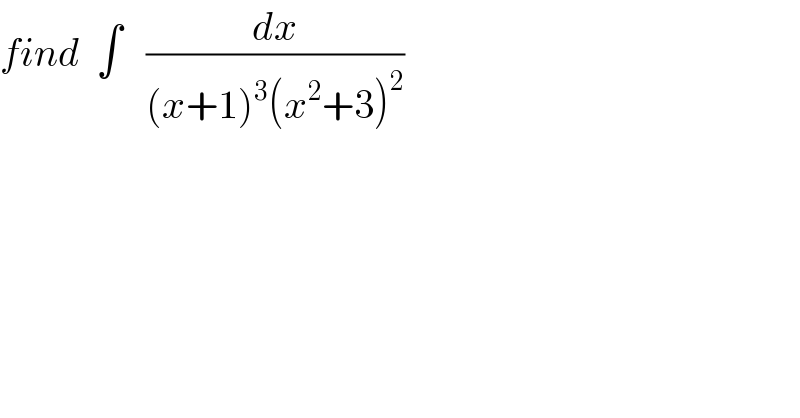
$${find}\:\:\int\:\:\:\frac{{dx}}{\left({x}+\mathrm{1}\right)^{\mathrm{3}} \left({x}^{\mathrm{2}} +\mathrm{3}\right)^{\mathrm{2}} } \\ $$
Commented by abdomathmax last updated on 20/Feb/20

$${let}\:{try}\:{complex}\:{method}\:{I}\:=\int\:\:\frac{{dx}}{\left({x}+\mathrm{1}\right)^{\mathrm{3}} \left({x}^{\mathrm{2}} +\mathrm{3}\right)^{\mathrm{2}} }{dx}\:\Rightarrow{I}=\int\:\:\frac{{dx}}{\left({x}+\mathrm{1}\right)^{\mathrm{3}} \left({x}−{i}\sqrt{\mathrm{3}}\right)^{\mathrm{2}} \left({x}+{i}\sqrt{\mathrm{3}}\right)^{\mathrm{2}} } \\ $$$$=\int\:\:\:\frac{{dx}}{\left(\frac{{x}+\mathrm{1}}{{x}+{i}\sqrt{\mathrm{3}}}\right)^{\mathrm{3}} ×\left({x}+{i}\sqrt{\mathrm{3}}\right)^{\mathrm{5}} \left({x}−{i}\sqrt{\mathrm{3}}\right)^{\mathrm{2}} } \\ $$$${changement}\:\frac{{x}+\mathrm{1}}{{x}+{i}\sqrt{\mathrm{3}}}\:={t}\:{give}\:{x}+\mathrm{1}={tx}+{i}\sqrt{\mathrm{3}}{t}\:\Rightarrow \\ $$$$\left(\mathrm{1}−{t}\right){x}={i}\sqrt{\mathrm{3}}{t}−\mathrm{1}\:\Rightarrow{x}=\frac{{i}\sqrt{\mathrm{3}}{t}−\mathrm{1}}{\mathrm{1}−{t}} \\ $$$${dx}\:=\frac{{i}\sqrt{\mathrm{3}}\left(\mathrm{1}−{t}\right)−\left({i}\sqrt{\mathrm{3}}{t}−\mathrm{1}\right)\left(−\mathrm{1}\right)}{\left(\mathrm{1}−{t}\right)^{\mathrm{2}} }{dt} \\ $$$$=\frac{{i}\sqrt{\mathrm{3}}−{i}\sqrt{\mathrm{3}}{t}+{i}\sqrt{\mathrm{3}}{t}−\mathrm{1}}{\left({t}−\mathrm{1}\right)^{\mathrm{2}} }{dt}\:=\frac{{i}\sqrt{\mathrm{3}}−\mathrm{1}}{\left({t}−\mathrm{1}\right)^{\mathrm{2}} }{dt} \\ $$$${x}+{i}\sqrt{\mathrm{3}}=\frac{{i}\sqrt{\mathrm{3}}{t}−\mathrm{1}}{\mathrm{1}−{t}}\:+{i}\sqrt{\mathrm{3}}=\frac{{i}\sqrt{\mathrm{3}}{t}−\mathrm{1}+{i}\sqrt{\mathrm{3}}−{i}\sqrt{\mathrm{3}}{t}}{\mathrm{1}−{t}} \\ $$$$=\frac{{i}\sqrt{\mathrm{3}}−\mathrm{1}}{\mathrm{1}−{t}}\:=\frac{\mathrm{1}−{i}\sqrt{\mathrm{3}}}{{t}−\mathrm{1}} \\ $$$${x}−{i}\sqrt{\mathrm{3}}=\frac{{i}\sqrt{\mathrm{3}}{t}−\mathrm{1}}{\mathrm{1}−{t}}−{i}\sqrt{\mathrm{3}}=\frac{{i}\sqrt{\mathrm{3}}{t}−\mathrm{1}−{i}\sqrt{\mathrm{3}}+{i}\sqrt{\mathrm{3}}{t}}{\left(\mathrm{1}−{t}\right)} \\ $$$$=\frac{\mathrm{2}{i}\sqrt{\mathrm{3}}{t}−\mathrm{1}−{i}\sqrt{\mathrm{3}}}{\left(\mathrm{1}−{t}\right)}\:=\frac{−\mathrm{2}{i}\sqrt{\mathrm{3}}{t}+\mathrm{1}+{i}\sqrt{\mathrm{3}}}{\left({t}−\mathrm{1}\right)}\:\Rightarrow \\ $$$${I}\:=\left({i}\sqrt{\mathrm{3}}−\mathrm{1}\right)\:\int\:\:\:\:\frac{{dt}}{\left({t}−\mathrm{1}\right)^{\mathrm{2}} \:{t}^{\mathrm{3}} \left(\frac{\mathrm{1}−{i}\sqrt{\mathrm{3}}}{{t}−\mathrm{1}}\right)^{\mathrm{5}} \left(\frac{−\mathrm{2}{i}\sqrt{\mathrm{3}}{t}+\mathrm{1}+{i}\sqrt{\mathrm{3}}}{{t}−\mathrm{1}}\right)^{\mathrm{2}} } \\ $$$$=\left({i}\sqrt{\mathrm{3}}−\mathrm{1}\right)\:\int\:\:\frac{\left({t}−\mathrm{1}\right)^{\mathrm{7}} \:{dt}}{\left({t}−\mathrm{1}\right)^{\mathrm{2}} {t}^{\mathrm{3}} \left(−\mathrm{2}{i}\sqrt{\mathrm{3}}{t}\:+\mathrm{1}+{i}\sqrt{\mathrm{3}}\right)^{\mathrm{2}} \left(\mathrm{1}−{i}\sqrt{\mathrm{3}}\right)^{\mathrm{5}} } \\ $$$$=−\frac{\mathrm{1}}{\left({i}\sqrt{\mathrm{3}}−\mathrm{1}\right)^{\mathrm{4}} }\:\int\:\:\:\frac{\left({t}−\mathrm{1}\right)^{\mathrm{5}} }{{t}^{\mathrm{3}} \left(−\mathrm{2}{i}\sqrt{\mathrm{3}}{t}\:+{i}\sqrt{\mathrm{3}}\right)^{\mathrm{2}} }{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}\left({i}\sqrt{\mathrm{3}}−\mathrm{1}\right)^{\mathrm{4}} }\:\int\:\:\:\frac{\left({t}−\mathrm{1}\right)^{\mathrm{5}} }{{t}^{\mathrm{2}} \left(−\mathrm{2}{t}+\mathrm{1}\right)^{\mathrm{2}} }{dt} \\ $$$$=\lambda\:\int\:\:\frac{\sum_{{k}=\mathrm{0}} ^{\mathrm{5}} \:{C}_{\mathrm{5}} ^{{k}} \:{t}^{{k}} \left(−\mathrm{1}\right)^{\mathrm{5}−{k}} }{{t}^{\mathrm{2}} \left(\mathrm{2}{t}−\mathrm{1}\right)^{\mathrm{2}} }{dt}\:\:\:\:\left(\lambda=\frac{\mathrm{1}}{\mathrm{3}\left({i}\sqrt{\mathrm{3}}−\mathrm{1}\right)^{\mathrm{4}} }\right) \\ $$$$=\lambda\int\:\:\frac{−\:{C}_{\mathrm{5}} ^{\mathrm{0}} \:+{C}_{\mathrm{5}} ^{\mathrm{1}} \:{t}−{C}_{\mathrm{5}} ^{\mathrm{2}} \:{t}^{\mathrm{2}} \:+{C}_{\mathrm{5}} ^{\mathrm{3}} \:{t}^{\mathrm{3}} \:−{C}_{\mathrm{5}} ^{\mathrm{4}} \:{t}^{\mathrm{4}} \:+{C}_{\mathrm{5}} ^{\mathrm{5}} \:{t}^{\mathrm{5}} }{{t}^{\mathrm{2}} \left(\mathrm{2}{t}−\mathrm{1}\right)^{\mathrm{2}} }{dt} \\ $$$$=\lambda\:\int\:\left\{−\frac{{C}_{\mathrm{5}} ^{\mathrm{0}} }{{t}^{\mathrm{2}} \left(\mathrm{2}{t}−\mathrm{1}\right)^{\mathrm{2}} }+\frac{{C}_{\mathrm{5}} ^{\mathrm{1}} }{{t}\left(\mathrm{2}{t}−\mathrm{1}\right)^{\mathrm{2}} }−\frac{{C}_{\mathrm{5}} ^{\mathrm{2}} }{\left(\mathrm{2}{t}−\mathrm{1}\right)^{\mathrm{2}} }\:+\frac{{C}_{\mathrm{5}} ^{\mathrm{3}} {t}}{\left(\mathrm{2}{t}−\mathrm{1}\right)^{\mathrm{2}} }\right. \\ $$$$\left.−\frac{{C}_{\mathrm{5}} ^{\mathrm{4}} \:{t}^{\mathrm{2}} }{\left(\mathrm{2}{t}−\mathrm{1}\right)^{\mathrm{2}} }\:+\frac{{C}_{\mathrm{5}} ^{\mathrm{5}} {t}^{\mathrm{5}} }{\left(\mathrm{2}{t}−\mathrm{1}\right)^{\mathrm{2}} }\right\}{dt}\:...{be}\:{continued}... \\ $$
Answered by MJS last updated on 13/Feb/20
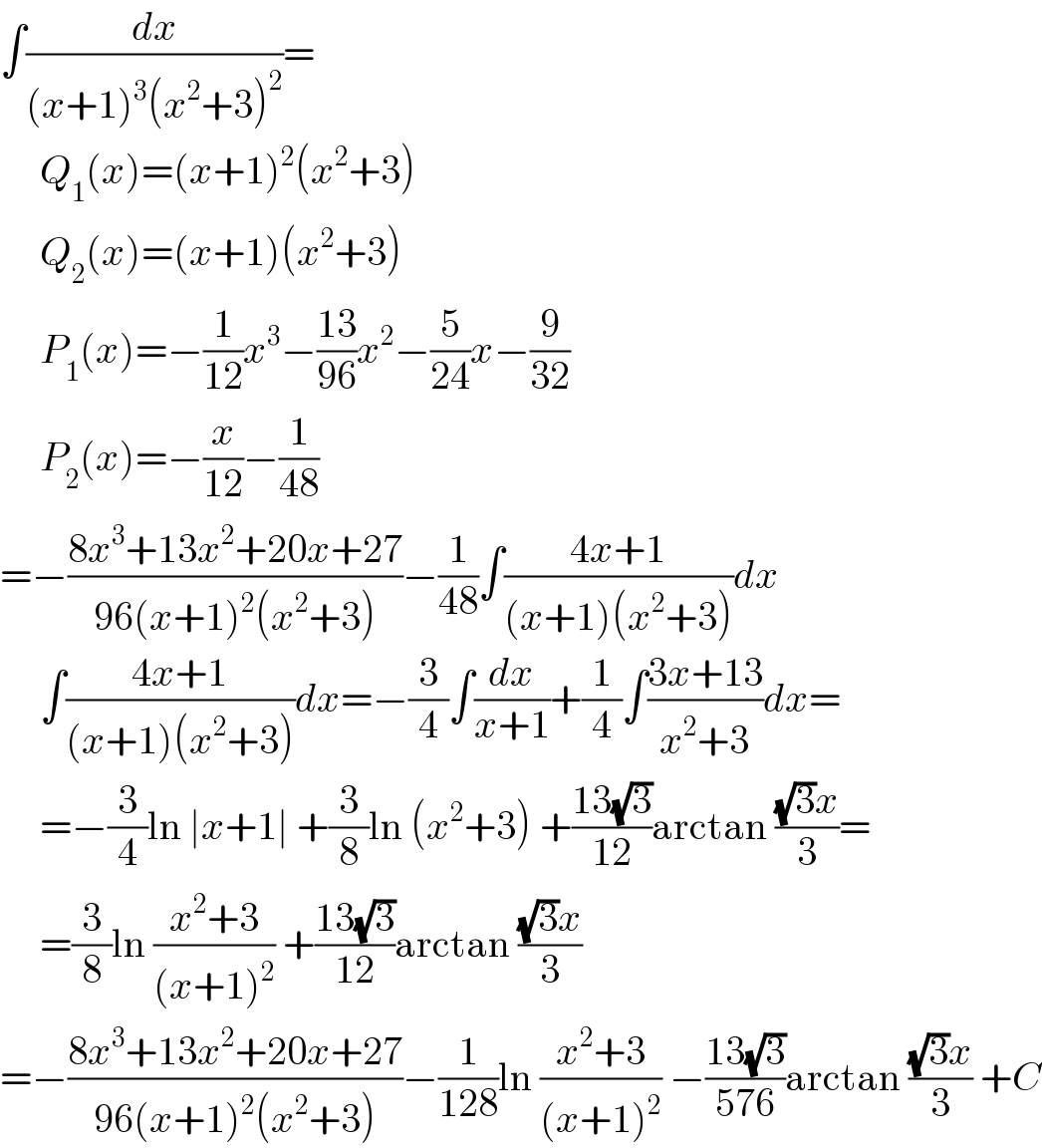
$$\int\frac{{dx}}{\left({x}+\mathrm{1}\right)^{\mathrm{3}} \left({x}^{\mathrm{2}} +\mathrm{3}\right)^{\mathrm{2}} }= \\ $$$$\:\:\:\:\:{Q}_{\mathrm{1}} \left({x}\right)=\left({x}+\mathrm{1}\right)^{\mathrm{2}} \left({x}^{\mathrm{2}} +\mathrm{3}\right) \\ $$$$\:\:\:\:\:{Q}_{\mathrm{2}} \left({x}\right)=\left({x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} +\mathrm{3}\right) \\ $$$$\:\:\:\:\:{P}_{\mathrm{1}} \left({x}\right)=−\frac{\mathrm{1}}{\mathrm{12}}{x}^{\mathrm{3}} −\frac{\mathrm{13}}{\mathrm{96}}{x}^{\mathrm{2}} −\frac{\mathrm{5}}{\mathrm{24}}{x}−\frac{\mathrm{9}}{\mathrm{32}} \\ $$$$\:\:\:\:\:{P}_{\mathrm{2}} \left({x}\right)=−\frac{{x}}{\mathrm{12}}−\frac{\mathrm{1}}{\mathrm{48}} \\ $$$$=−\frac{\mathrm{8}{x}^{\mathrm{3}} +\mathrm{13}{x}^{\mathrm{2}} +\mathrm{20}{x}+\mathrm{27}}{\mathrm{96}\left({x}+\mathrm{1}\right)^{\mathrm{2}} \left({x}^{\mathrm{2}} +\mathrm{3}\right)}−\frac{\mathrm{1}}{\mathrm{48}}\int\frac{\mathrm{4}{x}+\mathrm{1}}{\left({x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} +\mathrm{3}\right)}{dx} \\ $$$$\:\:\:\:\:\int\frac{\mathrm{4}{x}+\mathrm{1}}{\left({x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} +\mathrm{3}\right)}{dx}=−\frac{\mathrm{3}}{\mathrm{4}}\int\frac{{dx}}{{x}+\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{4}}\int\frac{\mathrm{3}{x}+\mathrm{13}}{{x}^{\mathrm{2}} +\mathrm{3}}{dx}= \\ $$$$\:\:\:\:\:=−\frac{\mathrm{3}}{\mathrm{4}}\mathrm{ln}\:\mid{x}+\mathrm{1}\mid\:+\frac{\mathrm{3}}{\mathrm{8}}\mathrm{ln}\:\left({x}^{\mathrm{2}} +\mathrm{3}\right)\:+\frac{\mathrm{13}\sqrt{\mathrm{3}}}{\mathrm{12}}\mathrm{arctan}\:\frac{\sqrt{\mathrm{3}}{x}}{\mathrm{3}}= \\ $$$$\:\:\:\:\:=\frac{\mathrm{3}}{\mathrm{8}}\mathrm{ln}\:\frac{{x}^{\mathrm{2}} +\mathrm{3}}{\left({x}+\mathrm{1}\right)^{\mathrm{2}} }\:+\frac{\mathrm{13}\sqrt{\mathrm{3}}}{\mathrm{12}}\mathrm{arctan}\:\frac{\sqrt{\mathrm{3}}{x}}{\mathrm{3}} \\ $$$$=−\frac{\mathrm{8}{x}^{\mathrm{3}} +\mathrm{13}{x}^{\mathrm{2}} +\mathrm{20}{x}+\mathrm{27}}{\mathrm{96}\left({x}+\mathrm{1}\right)^{\mathrm{2}} \left({x}^{\mathrm{2}} +\mathrm{3}\right)}−\frac{\mathrm{1}}{\mathrm{128}}\mathrm{ln}\:\frac{{x}^{\mathrm{2}} +\mathrm{3}}{\left({x}+\mathrm{1}\right)^{\mathrm{2}} }\:−\frac{\mathrm{13}\sqrt{\mathrm{3}}}{\mathrm{576}}\mathrm{arctan}\:\frac{\sqrt{\mathrm{3}}{x}}{\mathrm{3}}\:+{C} \\ $$
