
Question Number 77340 by BK last updated on 05/Jan/20
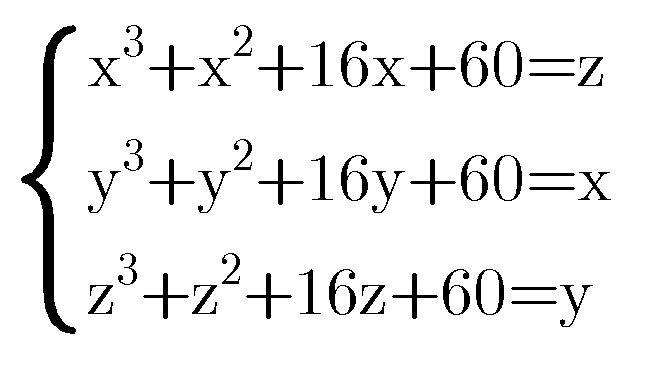
Answered by mind is power last updated on 05/Jan/20
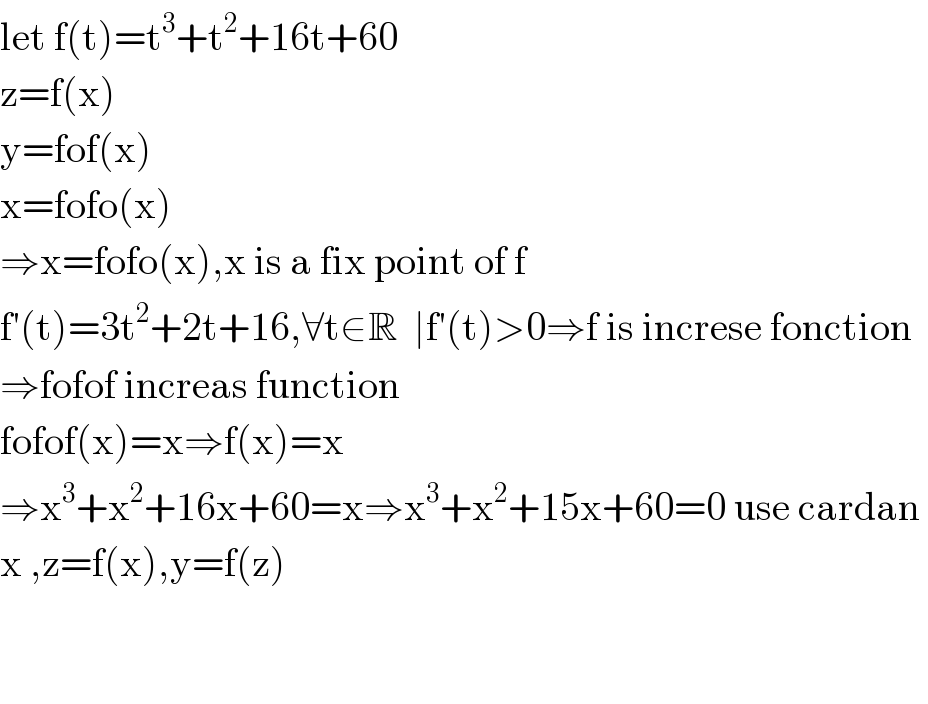
$$\mathrm{let}\:\mathrm{f}\left(\mathrm{t}\right)=\mathrm{t}^{\mathrm{3}} +\mathrm{t}^{\mathrm{2}} +\mathrm{16t}+\mathrm{60} \\ $$$$\mathrm{z}=\mathrm{f}\left(\mathrm{x}\right) \\ $$$$\mathrm{y}=\mathrm{fof}\left(\mathrm{x}\right) \\ $$$$\mathrm{x}=\mathrm{fofo}\left(\mathrm{x}\right) \\ $$$$\Rightarrow\mathrm{x}=\mathrm{fofo}\left(\mathrm{x}\right),\mathrm{x}\:\mathrm{is}\:\mathrm{a}\:\mathrm{fix}\:\mathrm{point}\:\mathrm{of}\:\mathrm{f}\: \\ $$$$\mathrm{f}'\left(\mathrm{t}\right)=\mathrm{3t}^{\mathrm{2}} +\mathrm{2t}+\mathrm{16},\forall\mathrm{t}\in\mathbb{R}\:\:\mid\mathrm{f}'\left(\mathrm{t}\right)>\mathrm{0}\Rightarrow\mathrm{f}\:\mathrm{is}\:\mathrm{increse}\:\mathrm{fonction} \\ $$$$\Rightarrow\mathrm{fofof}\:\mathrm{increas}\:\mathrm{function} \\ $$$$\mathrm{fofof}\left(\mathrm{x}\right)=\mathrm{x}\Rightarrow\mathrm{f}\left(\mathrm{x}\right)=\mathrm{x} \\ $$$$\Rightarrow\mathrm{x}^{\mathrm{3}} +\mathrm{x}^{\mathrm{2}} +\mathrm{16x}+\mathrm{60}=\mathrm{x}\Rightarrow\mathrm{x}^{\mathrm{3}} +\mathrm{x}^{\mathrm{2}} +\mathrm{15x}+\mathrm{60}=\mathrm{0}\:\mathrm{use}\:\mathrm{cardan}\: \\ $$$$\mathrm{x}\:,\mathrm{z}=\mathrm{f}\left(\mathrm{x}\right),\mathrm{y}=\mathrm{f}\left(\mathrm{z}\right) \\ $$$$ \\ $$$$ \\ $$
Commented by BK last updated on 05/Jan/20
$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir}\:\mathrm{great}! \\ $$
