
Question Number 75066 by ~blr237~ last updated on 06/Dec/19
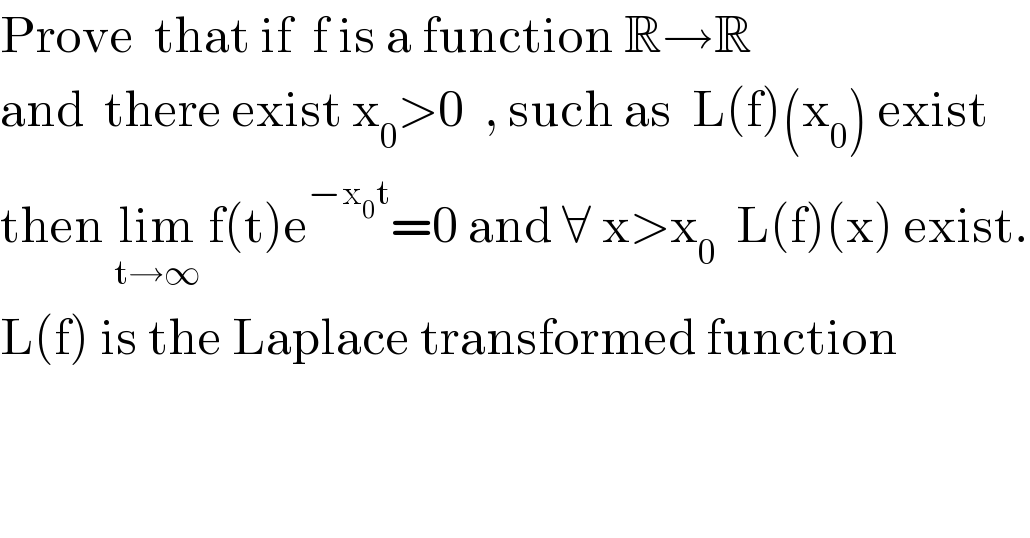
$$\mathrm{Prove}\:\:\mathrm{that}\:\mathrm{if}\:\:\mathrm{f}\:\mathrm{is}\:\mathrm{a}\:\mathrm{function}\:\mathbb{R}\rightarrow\mathbb{R}\: \\ $$ $$\mathrm{and}\:\:\mathrm{there}\:\mathrm{exist}\:\mathrm{x}_{\mathrm{0}} >\mathrm{0}\:\:,\:\mathrm{such}\:\mathrm{as}\:\:\mathrm{L}\left(\mathrm{f}\right)\left(\mathrm{x}_{\mathrm{0}} \right)\:\mathrm{exist}\: \\ $$ $$\mathrm{then}\:\underset{\mathrm{t}\rightarrow\infty} {\mathrm{lim}}\:\mathrm{f}\left(\mathrm{t}\right)\mathrm{e}^{−\mathrm{x}_{\mathrm{0}} \mathrm{t}} =\mathrm{0}\:\mathrm{and}\:\forall\:\mathrm{x}>\mathrm{x}_{\mathrm{0}} \:\:\mathrm{L}\left(\mathrm{f}\right)\left(\mathrm{x}\right)\:\mathrm{exist}. \\ $$ $$\mathrm{L}\left(\mathrm{f}\right)\:\mathrm{is}\:\mathrm{the}\:\mathrm{Laplace}\:\mathrm{transformed}\:\mathrm{function} \\ $$
Answered by mind is power last updated on 07/Dec/19

$$\mathrm{L}\left(\mathrm{f}\right)\left(\mathrm{s}\right)=\int_{\mathrm{0}} ^{+\infty} \mathrm{e}^{−\mathrm{st}} \mathrm{f}\left(\mathrm{t}\right)\mathrm{dt} \\ $$ $$\mathrm{L}\left(\mathrm{f}\right)\left(\mathrm{x}_{\mathrm{0}} \right)=\int_{\mathrm{0}} ^{+\infty} \mid\mathrm{e}^{−\mathrm{x}_{\mathrm{0}} \mathrm{t}} \mathrm{f}\left(\mathrm{t}\right)\mid\mathrm{dt}<\infty \\ $$ $$\Rightarrow\mathrm{e}^{−\mathrm{x}_{\mathrm{0}} \mathrm{t}} \mathrm{f}\left(\mathrm{t}\right)\:\mathrm{is}\:\mathrm{integrabl}\:\:\:\mathrm{in}+\infty \\ $$ $$\Rightarrow\mathrm{e}^{−\mathrm{x}_{\mathrm{0}} \mathrm{t}} \mathrm{f}\left(\mathrm{t}\right)\rightarrow\mathrm{0}\:\mathrm{by}\:\mathrm{cv}\: \\ $$ $$\mathrm{for}\:\mathrm{x}>\mathrm{x}_{\mathrm{0}} \\ $$ $$\mathrm{x}=\mathrm{x}_{\mathrm{0}} +\mathrm{n}\:,\mathrm{n}\in\mathbb{R}_{+} \\ $$ $$\mathrm{L}\left(\mathrm{f}\right)\left(\mathrm{x}\right)=\int_{\mathrm{0}} ^{+\infty} \mathrm{e}^{−\left(\mathrm{x}_{\mathrm{0}} +\mathrm{n}\right)\mathrm{t}} \mathrm{f}\left(\mathrm{t}\right)\mathrm{dt} \\ $$ $$=\int_{\mathrm{0}} ^{+\infty} \mathrm{e}^{−\mathrm{x}_{\mathrm{0}} \mathrm{t}} \mathrm{f}\left(\mathrm{t}\right).\mathrm{e}^{−\mathrm{nt}} \mathrm{dt} \\ $$ $$\mathrm{since}\:\mathrm{L}\left(\mathrm{f}\right)\left(\mathrm{x}_{\mathrm{0}} \right)\:\mathrm{exist}\Rightarrow\:\mathrm{lim}\:\mathrm{f}\left(\mathrm{t}\right)\mathrm{e}^{−\mathrm{x}_{\mathrm{0}} \mathrm{t}} =\mathrm{o}\Rightarrow\exists\mathrm{A}\in\mathbb{R}\:\:\forall\mathrm{x}>\mathrm{A} \\ $$ $$\mid\mathrm{f}\left(\mathrm{t}\right)\mathrm{e}^{−\mathrm{x}_{\mathrm{0}} \mathrm{t}} \mid<\mathrm{1}\Rightarrow\:\:\:\:\:\mid\mathrm{f}\left(\mathrm{t}\right)\mathrm{e}^{−\mathrm{x}_{\mathrm{0}} \mathrm{t}} .\mathrm{e}^{−\mathrm{nt}} \mid<\mathrm{e}^{−\mathrm{nt}} \\ $$ $$\mathrm{so}\:\mid\mathrm{f}\left(\mathrm{t}\right)\mathrm{e}^{−\left(\mathrm{x}_{\mathrm{0}} +\mathrm{n}\right)\mathrm{t}} \mid<\mathrm{e}^{−\mathrm{nt}} \:\:\:\mathrm{t}\rightarrow\mathrm{e}^{−\mathrm{nt}} \:\mathrm{is}\:\mathrm{integral}\:\mathrm{n}>\mathrm{0} \\ $$ $$\forall\mathrm{t}>\mathrm{A} \\ $$ $$\mathrm{so}\:\int_{\mathrm{0}} ^{+\infty} \mid\mathrm{f}\left(\mathrm{t}\right)\mathrm{e}^{−\left(\mathrm{x}_{\mathrm{0}} +\mathrm{n}\right)\mathrm{t}} \mid\mathrm{dt}\:\mathrm{exist}\Rightarrow\int_{\mathrm{0}} ^{+\infty} \mathrm{f}\left(\mathrm{t}\right)\mathrm{e}^{−\left(\mathrm{x}_{\mathrm{0}} +\mathrm{n}\right)\mathrm{t}\:} \mathrm{dt}\:\mathrm{exist} \\ $$ $$ \\ $$
Commented by~blr237~ last updated on 06/Dec/19

$$\mathrm{sir}\:\:\mathrm{L}\left(\mathrm{f}\right)\left(\mathrm{x}_{\mathrm{0}} \right)\:\mathrm{exist}\:\:\mathrm{just}\:\mathrm{mean}\:\mathrm{that}\:\int_{\mathrm{0}} ^{\infty} \mathrm{f}\left(\mathrm{t}\right)\mathrm{e}^{−\mathrm{x}_{\mathrm{0}} \mathrm{t}} \mathrm{dt}\:<+\infty\:\: \\ $$ $$\mathrm{that}\:\mathrm{condition}\:\mathrm{you}\:\mathrm{used}\:\mathrm{is}\:\mathrm{just}\:\mathrm{sufficient} \\ $$ $$\mathrm{cause}\:\mathrm{all}\:\:\mathrm{if}\:\:\mathrm{f}\in\:\mathrm{L}^{\mathrm{1}} \left(\mathbb{R}_{+} \right)\left(\int_{\mathrm{0}} ^{\infty} \mid\mathrm{f}\left(\mathrm{t}\right)\mid\mathrm{dt}<+\infty\right)\:\:\mathrm{then}\:\mathrm{L}\left(\mathrm{f}\right)\left(\mathrm{s}\right)\:\mathrm{exist}\:\mathrm{for}\:\mathrm{all}\:\mathrm{s}>\mathrm{0} \\ $$ $$ \\ $$
Commented bymind is power last updated on 07/Dec/19
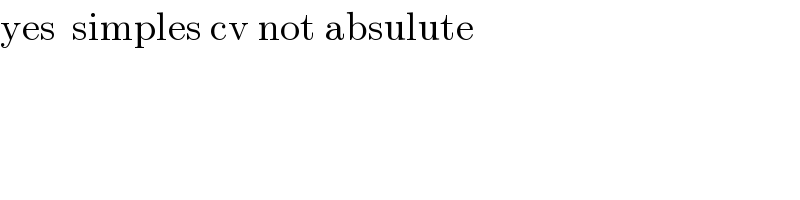
$$\mathrm{yes}\:\:\mathrm{simples}\:\mathrm{cv}\:\mathrm{not}\:\mathrm{absulute} \\ $$
