
Question Number 70757 by MJS last updated on 08/Oct/19

$$. \\ $$
Commented by TawaTawa last updated on 07/Oct/19
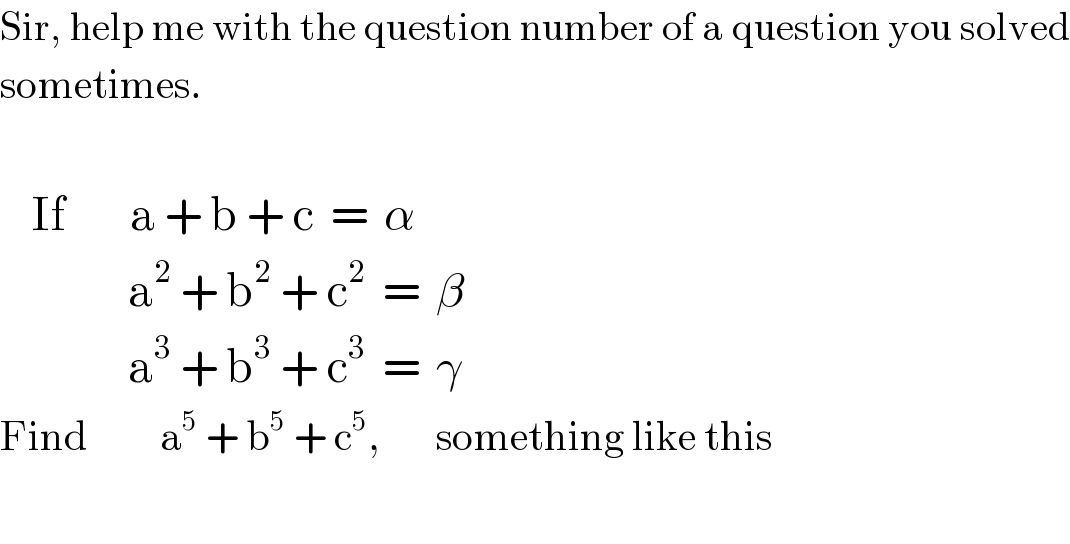
$$\mathrm{Sir},\:\mathrm{help}\:\mathrm{me}\:\mathrm{with}\:\mathrm{the}\:\mathrm{question}\:\mathrm{number}\:\mathrm{of}\:\mathrm{a}\:\mathrm{question}\:\mathrm{you}\:\mathrm{solved} \\ $$$$\mathrm{sometimes}. \\ $$$$ \\ $$$$\:\:\:\:\mathrm{If}\:\:\:\:\:\:\:\:\mathrm{a}\:+\:\mathrm{b}\:+\:\mathrm{c}\:\:=\:\:\alpha \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{a}^{\mathrm{2}} \:+\:\mathrm{b}^{\mathrm{2}} \:+\:\mathrm{c}^{\mathrm{2}} \:\:=\:\:\beta \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{a}^{\mathrm{3}} \:+\:\mathrm{b}^{\mathrm{3}} \:+\:\mathrm{c}^{\mathrm{3}} \:\:=\:\:\gamma \\ $$$$\mathrm{Find}\:\:\:\:\:\:\:\:\:\mathrm{a}^{\mathrm{5}} \:+\:\mathrm{b}^{\mathrm{5}} \:+\:\mathrm{c}^{\mathrm{5}} ,\:\:\:\:\:\:\:\mathrm{something}\:\mathrm{like}\:\mathrm{this} \\ $$$$ \\ $$
Commented by MJS last updated on 07/Oct/19
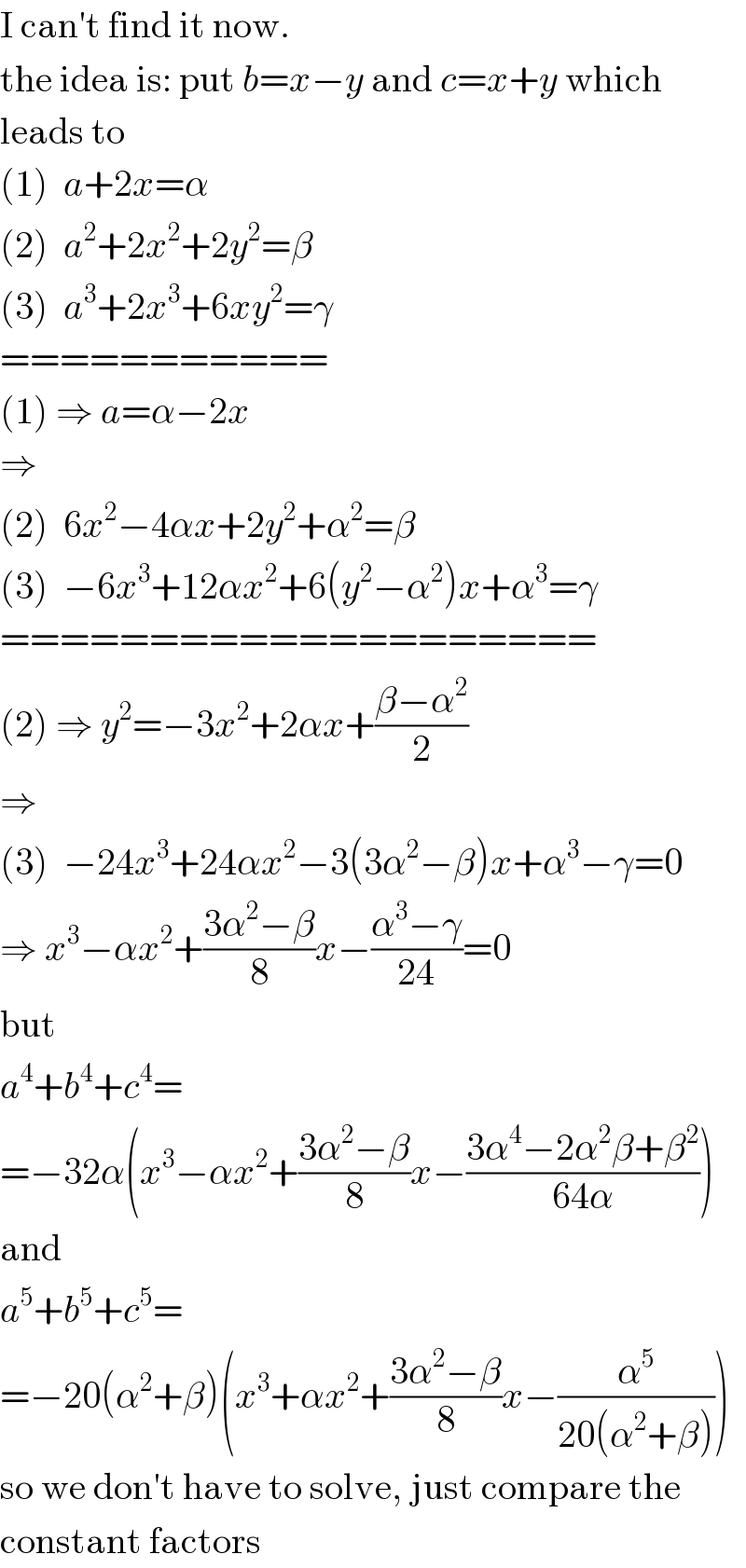
$$\mathrm{I}\:\mathrm{can}'\mathrm{t}\:\mathrm{find}\:\mathrm{it}\:\mathrm{now}. \\ $$$$\mathrm{the}\:\mathrm{idea}\:\mathrm{is}:\:\mathrm{put}\:{b}={x}−{y}\:\mathrm{and}\:{c}={x}+{y}\:\mathrm{which} \\ $$$$\mathrm{leads}\:\mathrm{to} \\ $$$$\left(\mathrm{1}\right)\:\:{a}+\mathrm{2}{x}=\alpha \\ $$$$\left(\mathrm{2}\right)\:\:{a}^{\mathrm{2}} +\mathrm{2}{x}^{\mathrm{2}} +\mathrm{2}{y}^{\mathrm{2}} =\beta \\ $$$$\left(\mathrm{3}\right)\:\:{a}^{\mathrm{3}} +\mathrm{2}{x}^{\mathrm{3}} +\mathrm{6}{xy}^{\mathrm{2}} =\gamma \\ $$$$=========== \\ $$$$\left(\mathrm{1}\right)\:\Rightarrow\:{a}=\alpha−\mathrm{2}{x} \\ $$$$\Rightarrow \\ $$$$\left(\mathrm{2}\right)\:\:\mathrm{6}{x}^{\mathrm{2}} −\mathrm{4}\alpha{x}+\mathrm{2}{y}^{\mathrm{2}} +\alpha^{\mathrm{2}} =\beta \\ $$$$\left(\mathrm{3}\right)\:\:−\mathrm{6}{x}^{\mathrm{3}} +\mathrm{12}\alpha{x}^{\mathrm{2}} +\mathrm{6}\left({y}^{\mathrm{2}} −\alpha^{\mathrm{2}} \right){x}+\alpha^{\mathrm{3}} =\gamma \\ $$$$==================== \\ $$$$\left(\mathrm{2}\right)\:\Rightarrow\:{y}^{\mathrm{2}} =−\mathrm{3}{x}^{\mathrm{2}} +\mathrm{2}\alpha{x}+\frac{\beta−\alpha^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\Rightarrow \\ $$$$\left(\mathrm{3}\right)\:\:−\mathrm{24}{x}^{\mathrm{3}} +\mathrm{24}\alpha{x}^{\mathrm{2}} −\mathrm{3}\left(\mathrm{3}\alpha^{\mathrm{2}} −\beta\right){x}+\alpha^{\mathrm{3}} −\gamma=\mathrm{0} \\ $$$$\Rightarrow\:{x}^{\mathrm{3}} −\alpha{x}^{\mathrm{2}} +\frac{\mathrm{3}\alpha^{\mathrm{2}} −\beta}{\mathrm{8}}{x}−\frac{\alpha^{\mathrm{3}} −\gamma}{\mathrm{24}}=\mathrm{0} \\ $$$$\mathrm{but} \\ $$$${a}^{\mathrm{4}} +{b}^{\mathrm{4}} +{c}^{\mathrm{4}} = \\ $$$$=−\mathrm{32}\alpha\left({x}^{\mathrm{3}} −\alpha{x}^{\mathrm{2}} +\frac{\mathrm{3}\alpha^{\mathrm{2}} −\beta}{\mathrm{8}}{x}−\frac{\mathrm{3}\alpha^{\mathrm{4}} −\mathrm{2}\alpha^{\mathrm{2}} \beta+\beta^{\mathrm{2}} }{\mathrm{64}\alpha}\right) \\ $$$$\mathrm{and} \\ $$$${a}^{\mathrm{5}} +{b}^{\mathrm{5}} +{c}^{\mathrm{5}} = \\ $$$$=−\mathrm{20}\left(\alpha^{\mathrm{2}} +\beta\right)\left({x}^{\mathrm{3}} +\alpha{x}^{\mathrm{2}} +\frac{\mathrm{3}\alpha^{\mathrm{2}} −\beta}{\mathrm{8}}{x}−\frac{\alpha^{\mathrm{5}} }{\mathrm{20}\left(\alpha^{\mathrm{2}} +\beta\right)}\right) \\ $$$$\mathrm{so}\:\mathrm{we}\:\mathrm{don}'\mathrm{t}\:\mathrm{have}\:\mathrm{to}\:\mathrm{solve},\:\mathrm{just}\:\mathrm{compare}\:\mathrm{the} \\ $$$$\mathrm{constant}\:\mathrm{factors} \\ $$
Commented by TawaTawa last updated on 07/Oct/19

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir},\:\:\mathrm{i}\:\mathrm{appreciate} \\ $$
