Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 68598 by Abdo msup. last updated on 14/Sep/19

$$find\int \frac{dx}{x^3-4x+3}$$

Commented by turbo msup by abdo last updated on 14/Sep/19

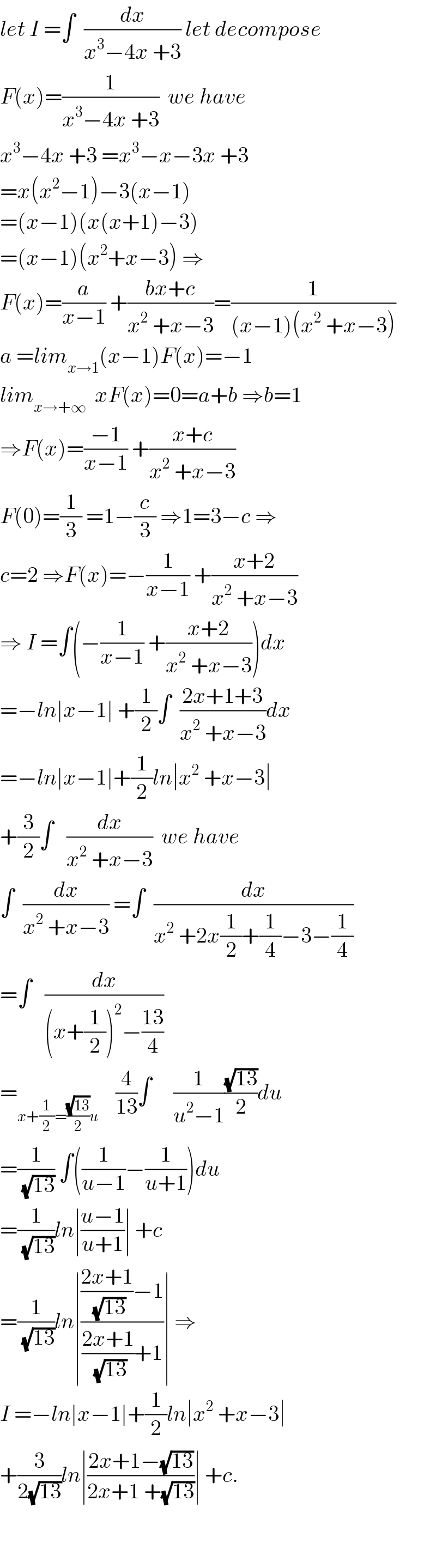
$$letI=\int \frac{dx}{x^3-4x+3}letdecompose$$$$F\left(x\right)=\frac{1}{x^3-4x+3}wehave$$$$x^3-4x+3=x^3-x-3x+3$$$$=x(x^2-1)-3(x-1)$$$$=(x-1)(x(x+1)-3)$$$$=(x-1)(x^2+x-3)\Rightarrow$$$$F\left(x\right)=\frac{a}{x-1}+\frac{bx+c}{x^2+x-3}=\frac{1}{(x-1)(x^2+x-3)}$$$$a={lim}_{x\to 1}(x-1)F\left(x\right)=-1$$$${lim}_{x\to +\mathrm{\infty }}xF\left(x\right)=0=a+b\Rightarrow b=1$$$$\Rightarrow F\left(x\right)=\frac{-1}{x-1}+\frac{x+c}{x^2+x-3}$$$$F\left(0\right)=\frac{1}{3}=1-\frac{c}{3}\Rightarrow 1=3-c\Rightarrow$$$$c=2\Rightarrow F\left(x\right)=-\frac{1}{x-1}+\frac{x+2}{x^2+x-3}$$$$\Rightarrow I=\int (-\frac{1}{x-1}+\frac{x+2}{x^2+x-3})dx$$$$=-ln\mid x-1\mid +\frac{1}{2}\int \frac{2x+1+3}{x^2+x-3}dx$$$$=-ln\mid x-1\mid +\frac{1}{2}ln\mid x^2+x-3\mid$$$$+\frac{3}{2}\int \frac{dx}{x^2+x-3}wehave$$$$\int \frac{dx}{x^2+x-3}=\int \frac{dx}{x^2+2x\frac{1}{2}+\frac{1}{4}-3-\frac{1}{4}}$$$$=\int \frac{dx}{{(x+\frac{1}{2})}^2-\frac{13}{4}}$$$${=}_{x+\frac{1}{2}=\frac{\sqrt{13}}{2}u}\frac{4}{13}\int \frac{1}{u^2-1}\frac{\sqrt{13}}{2}du$$$$=\frac{1}{\sqrt{13}}\int (\frac{1}{u-1}-\frac{1}{u+1})du$$$$=\frac{1}{\sqrt{13}}ln\mid \frac{u-1}{u+1}\mid +c$$$$=\frac{1}{\sqrt{13}}ln\mid \frac{\frac{2x+1}{\sqrt{13}}-1}{\frac{2x+1}{\sqrt{13}}+1}\mid \Rightarrow$$$$I=-ln\mid x-1\mid +\frac{1}{2}ln\mid x^2+x-3\mid$$$$+\frac{3}{2\sqrt{13}}ln\mid \frac{2x+1-\sqrt{13}}{2x+1+\sqrt{13}}\mid +c.$$$$$$$$$$

Commented by som(math1967) last updated on 14/Sep/19

$$\int \frac{dx}{(x-1)(x^2+x-3)}$$$$let(x-1)(Ax+B)+C(x^2+x-3)=1$$$$puttingx=1.....C=-1$$$$x=0........B=2$$$$x=-1........A=1$$$$\therefore \int \frac{\{(x-1)(x+2)-(x^2+x-3)\}dx}{(x-1)(x^2+x-3)}$$$$=\int \frac{(x+2)dx}{(x^2+x-3)}-\int \frac{dx}{(x-1)}$$$$=\frac{1}{2}\int \frac{(2x+4)dx}{x^2+x-3}-ln(x-1)$$$$=\frac{1}{2}\int \frac{d(x^2+x-3)}{(x^2+x-3)}+\frac{3}{2}\int \frac{dx}{{(x+\frac{1}{2})}^2-{\left(\frac{\sqrt{13}}{2}\right)}^2}-lnx$$$$=\frac{1}{2}ln(x^2+x-3)+\frac{3}{2}\times \frac{1}{\sqrt{13}}ln\left(\frac{x+\frac{1}{2}-\frac{\sqrt{13}}{2}}{x+\frac{1}{2}+\frac{\sqrt{13}}{2}}\right)-ln(x-1)+K$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com