
Question Number 68220 by ~ À ® @ 237 ~ last updated on 07/Sep/19
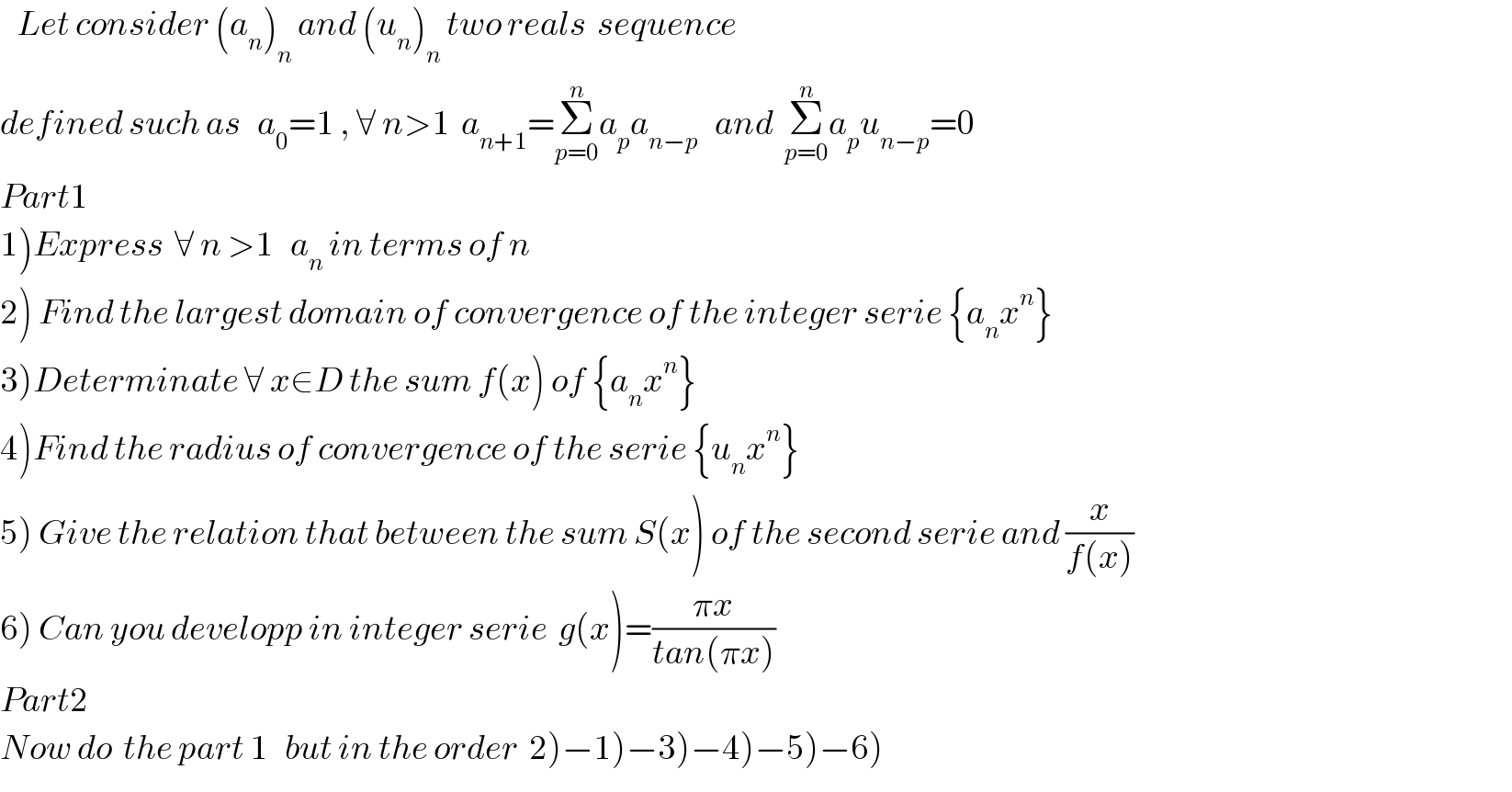
$$\:\:\:{Let}\:{consider}\:\left({a}_{{n}} \right)_{{n}} \:{and}\:\left({u}_{{n}} \right)_{{n}} \:{two}\:{reals}\:\:{sequence}\:\: \\ $$ $${defined}\:{such}\:{as}\:\:\:{a}_{\mathrm{0}} =\mathrm{1}\:,\:\forall\:{n}>\mathrm{1}\:\:{a}_{{n}+\mathrm{1}} =\underset{{p}=\mathrm{0}} {\overset{{n}} {\sum}}{a}_{{p}} {a}_{{n}−{p}} \:\:\:{and}\:\:\underset{{p}=\mathrm{0}} {\overset{{n}} {\sum}}{a}_{{p}} {u}_{{n}−{p}} =\mathrm{0} \\ $$ $${Part}\mathrm{1} \\ $$ $$\left.\mathrm{1}\right){Express}\:\:\forall\:{n}\:>\mathrm{1}\:\:\:{a}_{{n}} \:{in}\:{terms}\:{of}\:{n} \\ $$ $$\left.\mathrm{2}\right)\:{Find}\:{the}\:{largest}\:{domain}\:{of}\:{convergence}\:{of}\:{the}\:{integer}\:{serie}\:\left\{{a}_{{n}} {x}^{{n}} \right\} \\ $$ $$\left.\mathrm{3}\right){Determinate}\:\forall\:{x}\in{D}\:{the}\:{sum}\:{f}\left({x}\right)\:{of}\:\left\{{a}_{{n}} {x}^{{n}} \right\} \\ $$ $$\left.\mathrm{4}\right){Find}\:{the}\:{radius}\:{of}\:{convergence}\:{of}\:{the}\:{serie}\:\left\{{u}_{{n}} {x}^{{n}} \right\}\: \\ $$ $$\left.\mathrm{5}\right)\:{Give}\:{the}\:{relation}\:{that}\:{between}\:{the}\:{sum}\:{S}\left({x}\right)\:{of}\:{the}\:{second}\:{serie}\:{and}\:\frac{{x}}{{f}\left({x}\right)}\: \\ $$ $$\left.\mathrm{6}\right)\:{Can}\:{you}\:{developp}\:{in}\:{integer}\:{serie}\:\:{g}\left({x}\right)=\frac{\pi{x}}{{tan}\left(\pi{x}\right)} \\ $$ $${Part}\mathrm{2} \\ $$ $$\left.{N}\left.{o}\left.{w}\left.\:\left.{d}\left.{o}\:\:{the}\:{part}\:\mathrm{1}\:\:\:{but}\:{in}\:{the}\:{order}\:\:\mathrm{2}\right)−\mathrm{1}\right)−\mathrm{3}\right)−\mathrm{4}\right)−\mathrm{5}\right)−\mathrm{6}\right) \\ $$
