
Previous in Relation and Functions Next in Relation and Functions
Question Number 68019 by mathmax by abdo last updated on 03/Sep/19
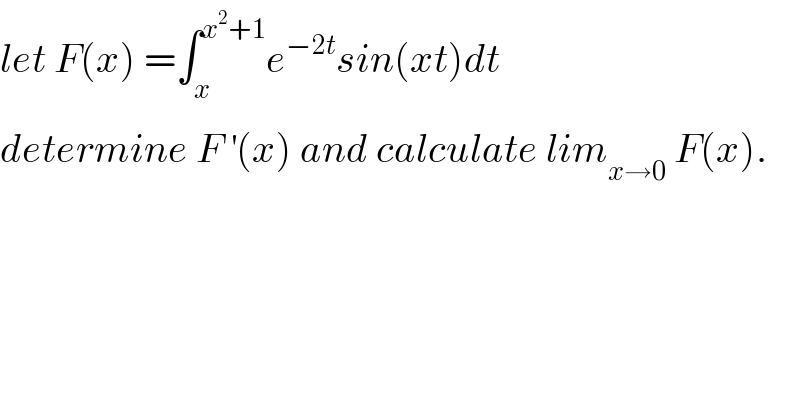
$${let}\:{F}\left({x}\right)\:=\int_{{x}} ^{{x}^{\mathrm{2}} +\mathrm{1}} {e}^{−\mathrm{2}{t}} {sin}\left({xt}\right){dt} \\ $$$${determine}\:{F}\:^{'} \left({x}\right)\:{and}\:{calculate}\:{lim}_{{x}\rightarrow\mathrm{0}} \:{F}\left({x}\right). \\ $$$$ \\ $$
Commented by kaivan.ahmadi last updated on 05/Sep/19

$${thank}\:{you}\:{sir}\:{abdo} \\ $$
Commented by mathmax by abdo last updated on 05/Sep/19
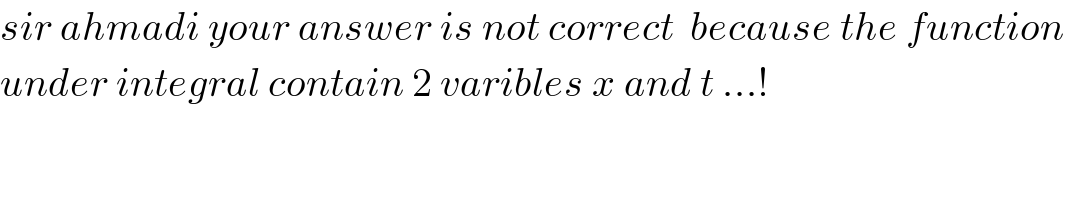
$${sir}\:{ahmadi}\:{your}\:{answer}\:{is}\:{not}\:{correct}\:\:{because}\:{the}\:{function}\: \\ $$$${under}\:{integral}\:{contain}\:\mathrm{2}\:{varibles}\:{x}\:{and}\:{t}\:...! \\ $$
Commented by mathmax by abdo last updated on 05/Sep/19
![F(x) =∫_x ^(x^2 +1) e^(−2t) sin(xt)dt we have u(x)=x and v(x)=x^2 +1and g(x,t) =e^(−2t) sin(xt) we use the formulae F^, (x) =∫_(u(x)) ^(v(x)) (∂g/∂x)(x,t)dt +v^′ g(x,v)−u^′ g(x,u) =∫_x ^(x^2 +1) t e^(−2t) cos(xt)dt +2x g(x,x^2 +1)−g(x,x^2 ) =∫_x ^(x^2 +1) t e^(−2t) cos(xt)dt +2xe^(−2(x^2 +1)) sin(x(x^2 +1))−e^(−2x^2 ) sin(x^3 ) we have ∫_x ^(x^2 +1 ) t e^(−2t) cos(xt)dt =Re(∫_x ^(x^2 +1) t e^(−2t+ixt) dt) ∫_x ^(x^2 +1) t e^((−2+ix)t) dt =[(t/(−2+ix)) e^((−2+ix)t) ]_(t=x) ^(t=x^2 +1) −∫_x ^(x^2 +1) (1/(−2+ix))e^((−2+ix)t) dt =(1/(−2+ix)){(x^2 +1)e^((−2+ix)(x^2 +1)) −xe^((−2+ix)x) } −(1/(−2+ix)) ∫_x ^(x^2 +1) e^((−2+ix)t) dt ....rest to finich the calculus.](Q68103.png)
$${F}\left({x}\right)\:=\int_{{x}} ^{{x}^{\mathrm{2}} \:+\mathrm{1}} \:{e}^{−\mathrm{2}{t}} \:{sin}\left({xt}\right){dt}\:\:{we}\:{have}\:{u}\left({x}\right)={x}\:{and}\:{v}\left({x}\right)={x}^{\mathrm{2}} \:+\mathrm{1}{and} \\ $$$${g}\left({x},{t}\right)\:={e}^{−\mathrm{2}{t}} {sin}\left({xt}\right) \\ $$$${we}\:{use}\:{the}\:{formulae}\:\:\:{F}\:^{,} \left({x}\right)\:=\int_{{u}\left({x}\right)} ^{{v}\left({x}\right)} \:\frac{\partial{g}}{\partial{x}}\left({x},{t}\right){dt}\:+{v}^{'} {g}\left({x},{v}\right)−{u}^{'} {g}\left({x},{u}\right) \\ $$$$=\int_{{x}} ^{{x}^{\mathrm{2}} \:+\mathrm{1}} \:\:{t}\:{e}^{−\mathrm{2}{t}} \:{cos}\left({xt}\right){dt}\:+\mathrm{2}{x}\:{g}\left({x},{x}^{\mathrm{2}} \:+\mathrm{1}\right)−{g}\left({x},{x}^{\mathrm{2}} \right) \\ $$$$=\int_{{x}} ^{{x}^{\mathrm{2}} \:+\mathrm{1}} \:{t}\:{e}^{−\mathrm{2}{t}} \:{cos}\left({xt}\right){dt}\:+\mathrm{2}{xe}^{−\mathrm{2}\left({x}^{\mathrm{2}} +\mathrm{1}\right)} \:{sin}\left({x}\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)\right)−{e}^{−\mathrm{2}{x}^{\mathrm{2}} } {sin}\left({x}^{\mathrm{3}} \right) \\ $$$${we}\:{have}\:\int_{{x}} ^{{x}^{\mathrm{2}} \:+\mathrm{1}\:} \:{t}\:{e}^{−\mathrm{2}{t}} \:{cos}\left({xt}\right){dt}\:={Re}\left(\int_{{x}} ^{{x}^{\mathrm{2}} +\mathrm{1}} \:{t}\:{e}^{−\mathrm{2}{t}+{ixt}} \:{dt}\right) \\ $$$$\int_{{x}} ^{{x}^{\mathrm{2}} \:+\mathrm{1}} \:{t}\:{e}^{\left(−\mathrm{2}+{ix}\right){t}} \:{dt}\:=\left[\frac{{t}}{−\mathrm{2}+{ix}}\:{e}^{\left(−\mathrm{2}+{ix}\right){t}} \right]_{{t}={x}} ^{{t}={x}^{\mathrm{2}} \:+\mathrm{1}} −\int_{{x}} ^{{x}^{\mathrm{2}} \:+\mathrm{1}} \frac{\mathrm{1}}{−\mathrm{2}+{ix}}{e}^{\left(−\mathrm{2}+{ix}\right){t}} {dt} \\ $$$$=\frac{\mathrm{1}}{−\mathrm{2}+{ix}}\left\{\left({x}^{\mathrm{2}} \:+\mathrm{1}\right){e}^{\left(−\mathrm{2}+{ix}\right)\left({x}^{\mathrm{2}} +\mathrm{1}\right)} −{xe}^{\left(−\mathrm{2}+{ix}\right){x}} \right\} \\ $$$$−\frac{\mathrm{1}}{−\mathrm{2}+{ix}}\:\int_{{x}} ^{{x}^{\mathrm{2}} +\mathrm{1}} \:{e}^{\left(−\mathrm{2}+{ix}\right){t}} {dt}\:....{rest}\:{to}\:{finich}\:{the}\:{calculus}. \\ $$
Commented by mathmax by abdo last updated on 06/Sep/19
$${you}\:{are}\:{welcome}\:{sir}. \\ $$
Commented by mathmax by abdo last updated on 08/Sep/19
![we have F(x)=∫_x ^(x^2 +1) e^(−2t) sin(xt)dt ⇒F(x)=Im(∫_x ^(x^2 +1) e^(−2t+ixt) dt) ∫_x ^(x^2 +1) e^((−2+ix)t) dt =[(1/(−2+ix)) e^((−2+ix)t) ]_x ^(x^2 +1) =((−1)/(2−ix)){ e^((−2+ix)(x^2 +1)) −e^((−2+ix)x) } =((−1)/(2−ix)){ e^(−2(x^2 +1)) e^(i(x^3 +x)) −e^(−2x) e^(ix^2 ) } =((−1)/(2−ix)){ e^(−2x^2 −2) (cos(x^3 +x)+isin(x^3 +x)−e^(−2x) (cos(x^2 )+isin(x^2 )} =−((2+ix)/(4+x^2 )){ e^(−2x^2 −2) cos(x^3 +x)−e^(−2x) cos(x^2 ) +i( e^(−2x^2 −2) sin(x^3 +x)−e^(−2x) sin(x^2 )} =−(1/(4+x^2 ))(2+ix){......} =−(1/(4+x^2 )){ 2( e^(−2x^2 −2) cos(x^3 +x)−e^(−2x) cos(x^2 )) +2i(e^(−2x^2 −2) sin(x^3 +x)−e^(−2x) sin(x^2 )) +ix( e^(−2x^2 −2) cos(x^3 +x) −e^(−2x) cos(x^2 )−x(e^(−2x^2 −2) sin(x^3 +x)−e^(−2x) sin(x^2 )} ⇒ F(x) =−(1/(4+x^2 )){ 2(e^(−2x^2 −2) sin(x^3 +x)−e^(−2x) sin(x^2 )) +x(e^(−2x^2 −2) cos(x^3 +x)−e^(−2x) cos(x^2 )} ⇒lim_(x→0) F(x) =0](Q68258.png)
$${we}\:{have}\:{F}\left({x}\right)=\int_{{x}} ^{{x}^{\mathrm{2}} +\mathrm{1}} \:{e}^{−\mathrm{2}{t}} \:{sin}\left({xt}\right){dt}\:\Rightarrow{F}\left({x}\right)={Im}\left(\int_{{x}} ^{{x}^{\mathrm{2}} +\mathrm{1}} \:{e}^{−\mathrm{2}{t}+{ixt}} {dt}\right) \\ $$$$\int_{{x}} ^{{x}^{\mathrm{2}} +\mathrm{1}} \:{e}^{\left(−\mathrm{2}+{ix}\right){t}} {dt}\:=\left[\frac{\mathrm{1}}{−\mathrm{2}+{ix}}\:{e}^{\left(−\mathrm{2}+{ix}\right){t}} \right]_{{x}} ^{{x}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$$=\frac{−\mathrm{1}}{\mathrm{2}−{ix}}\left\{\:\:{e}^{\left(−\mathrm{2}+{ix}\right)\left({x}^{\mathrm{2}} +\mathrm{1}\right)} −{e}^{\left(−\mathrm{2}+{ix}\right){x}} \right\} \\ $$$$=\frac{−\mathrm{1}}{\mathrm{2}−{ix}}\left\{\:{e}^{−\mathrm{2}\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)} \:{e}^{{i}\left({x}^{\mathrm{3}} \:+{x}\right)} \:−{e}^{−\mathrm{2}{x}} \:{e}^{{ix}^{\mathrm{2}} } \right\} \\ $$$$=\frac{−\mathrm{1}}{\mathrm{2}−{ix}}\left\{\:{e}^{−\mathrm{2}{x}^{\mathrm{2}} −\mathrm{2}} \left({cos}\left({x}^{\mathrm{3}} +{x}\right)+{isin}\left({x}^{\mathrm{3}} +{x}\right)−{e}^{−\mathrm{2}{x}} \left({cos}\left({x}^{\mathrm{2}} \right)+{isin}\left({x}^{\mathrm{2}} \right)\right\}\right.\right. \\ $$$$=−\frac{\mathrm{2}+{ix}}{\mathrm{4}+{x}^{\mathrm{2}} }\left\{\:\:{e}^{−\mathrm{2}{x}^{\mathrm{2}} −\mathrm{2}} {cos}\left({x}^{\mathrm{3}} \:+{x}\right)−{e}^{−\mathrm{2}{x}} {cos}\left({x}^{\mathrm{2}} \right)\right. \\ $$$$+{i}\left(\:{e}^{−\mathrm{2}{x}^{\mathrm{2}} −\mathrm{2}} \:{sin}\left({x}^{\mathrm{3}} \:+{x}\right)−{e}^{−\mathrm{2}{x}} {sin}\left({x}^{\mathrm{2}} \right)\right\} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}+{x}^{\mathrm{2}} }\left(\mathrm{2}+{ix}\right)\left\{......\right\} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}+{x}^{\mathrm{2}} }\left\{\:\:\mathrm{2}\left(\:{e}^{−\mathrm{2}{x}^{\mathrm{2}} −\mathrm{2}} \:{cos}\left({x}^{\mathrm{3}} \:+{x}\right)−{e}^{−\mathrm{2}{x}} {cos}\left({x}^{\mathrm{2}} \right)\right)\right. \\ $$$$+\mathrm{2}{i}\left({e}^{−\mathrm{2}{x}^{\mathrm{2}} −\mathrm{2}} \:{sin}\left({x}^{\mathrm{3}} \:+{x}\right)−{e}^{−\mathrm{2}{x}} {sin}\left({x}^{\mathrm{2}} \right)\right)\:+{ix}\left(\:{e}^{−\mathrm{2}{x}^{\mathrm{2}} −\mathrm{2}} {cos}\left({x}^{\mathrm{3}} \:+{x}\right)\right. \\ $$$$−{e}^{−\mathrm{2}{x}} \:{cos}\left({x}^{\mathrm{2}} \right)−{x}\left({e}^{−\mathrm{2}{x}^{\mathrm{2}} −\mathrm{2}} {sin}\left({x}^{\mathrm{3}} \:+{x}\right)−{e}^{−\mathrm{2}{x}} {sin}\left({x}^{\mathrm{2}} \right)\right\}\:\Rightarrow \\ $$$${F}\left({x}\right)\:=−\frac{\mathrm{1}}{\mathrm{4}+{x}^{\mathrm{2}} }\left\{\:\:\mathrm{2}\left({e}^{−\mathrm{2}{x}^{\mathrm{2}} −\mathrm{2}} {sin}\left({x}^{\mathrm{3}} +{x}\right)−{e}^{−\mathrm{2}{x}} {sin}\left({x}^{\mathrm{2}} \right)\right)\right. \\ $$$$+{x}\left({e}^{−\mathrm{2}{x}^{\mathrm{2}} −\mathrm{2}} \:{cos}\left({x}^{\mathrm{3}} +{x}\right)−{e}^{−\mathrm{2}{x}} {cos}\left({x}^{\mathrm{2}} \right)\right\}\:\Rightarrow{lim}_{{x}\rightarrow\mathrm{0}} {F}\left({x}\right)\:=\mathrm{0} \\ $$
