
Previous in Relation and Functions Next in Relation and Functions
Question Number 67540 by mathmax by abdo last updated on 28/Aug/19
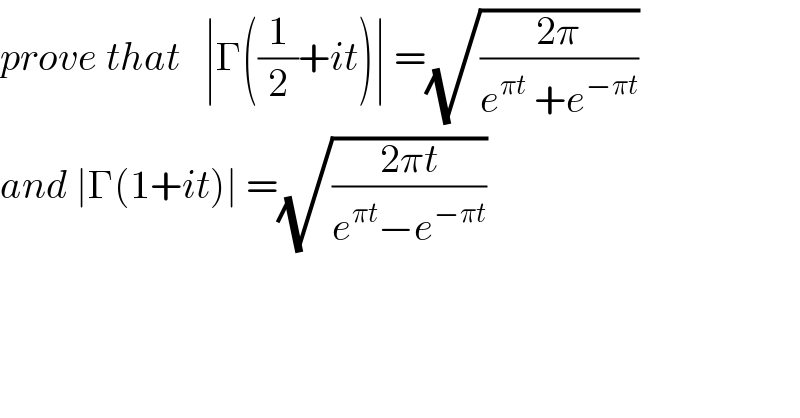
$${prove}\:{that}\:\:\:\mid\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}+{it}\right)\mid\:=\sqrt{\frac{\mathrm{2}\pi}{{e}^{\pi{t}} \:+{e}^{−\pi{t}} }} \\ $$$${and}\:\mid\Gamma\left(\mathrm{1}+{it}\right)\mid\:=\sqrt{\frac{\mathrm{2}\pi{t}}{{e}^{\pi{t}} −{e}^{−\pi{t}} }} \\ $$
Commented by ~ À ® @ 237 ~ last updated on 28/Aug/19
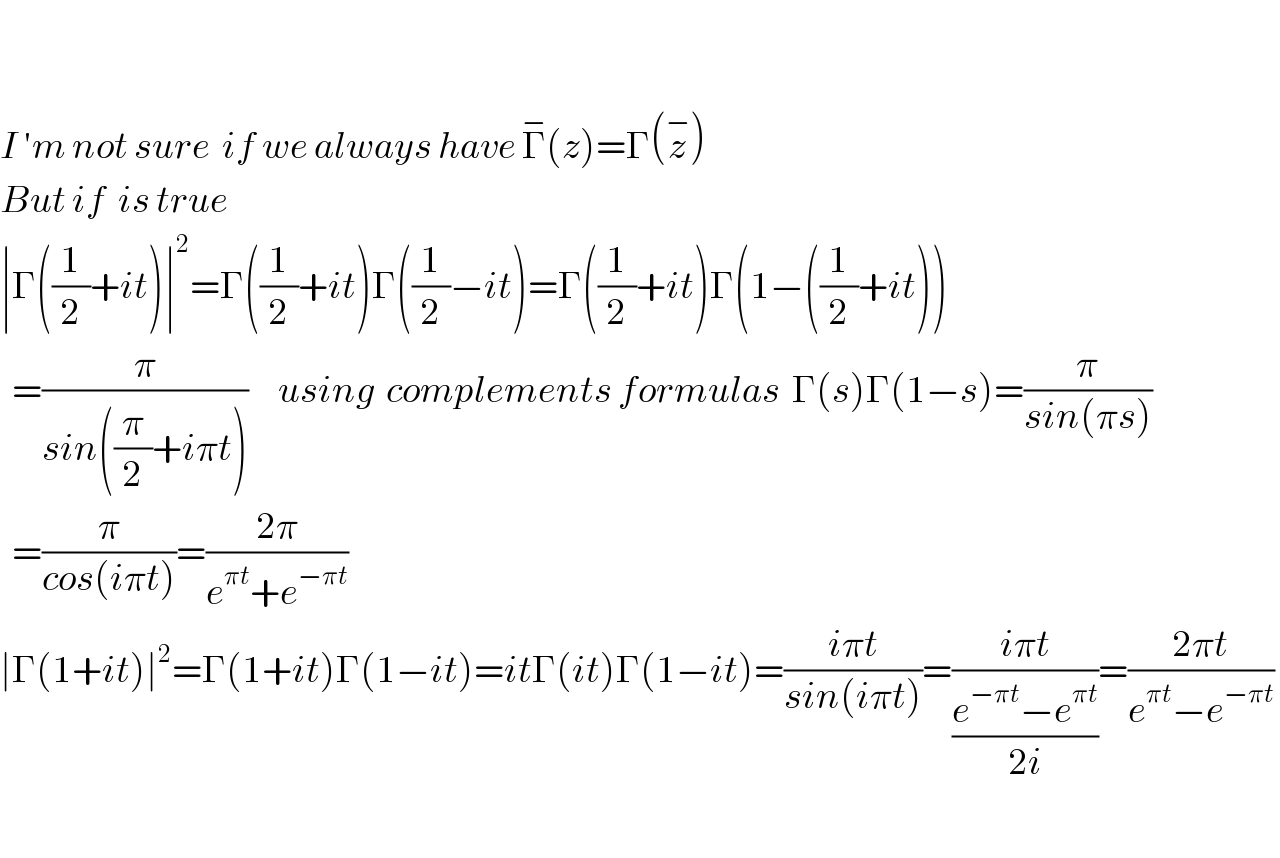
$$ \\ $$$$ \\ $$$${I}\:'{m}\:{not}\:{sure}\:\:{if}\:{we}\:{always}\:{have}\:\overset{−} {\Gamma}\left({z}\right)=\Gamma\left(\overset{−} {{z}}\right) \\ $$$${But}\:{if}\:\:{is}\:{true}\: \\ $$$$\mid\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}+{it}\right)\mid^{\mathrm{2}} =\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}+{it}\right)\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}−{it}\right)=\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}+{it}\right)\Gamma\left(\mathrm{1}−\left(\frac{\mathrm{1}}{\mathrm{2}}+{it}\right)\right) \\ $$$$\:\:=\frac{\pi}{{sin}\left(\frac{\pi}{\mathrm{2}}+{i}\pi{t}\right)}\:\:\:\:\:{using}\:\:{complements}\:{formulas}\:\:\Gamma\left({s}\right)\Gamma\left(\mathrm{1}−{s}\right)=\frac{\pi}{{sin}\left(\pi{s}\right)} \\ $$$$\:\:=\frac{\pi}{{cos}\left({i}\pi{t}\right)}=\frac{\mathrm{2}\pi}{{e}^{\pi{t}} +{e}^{−\pi{t}} }\: \\ $$$$\mid\Gamma\left(\mathrm{1}+{it}\right)\mid^{\mathrm{2}} =\Gamma\left(\mathrm{1}+{it}\right)\Gamma\left(\mathrm{1}−{it}\right)={it}\Gamma\left({it}\right)\Gamma\left(\mathrm{1}−{it}\right)=\frac{{i}\pi{t}}{{sin}\left({i}\pi{t}\right)}=\frac{{i}\pi{t}}{\frac{{e}^{−\pi{t}} −{e}^{\pi{t}} }{\mathrm{2}{i}}}=\frac{\mathrm{2}\pi{t}}{{e}^{\pi{t}} −{e}^{−\pi{t}} }\: \\ $$$$ \\ $$
Commented by mathmax by abdo last updated on 29/Aug/19

$${thank}\:{you}\:{sir}. \\ $$
