
Question Number 64735 by mmkkmm000m last updated on 20/Jul/19
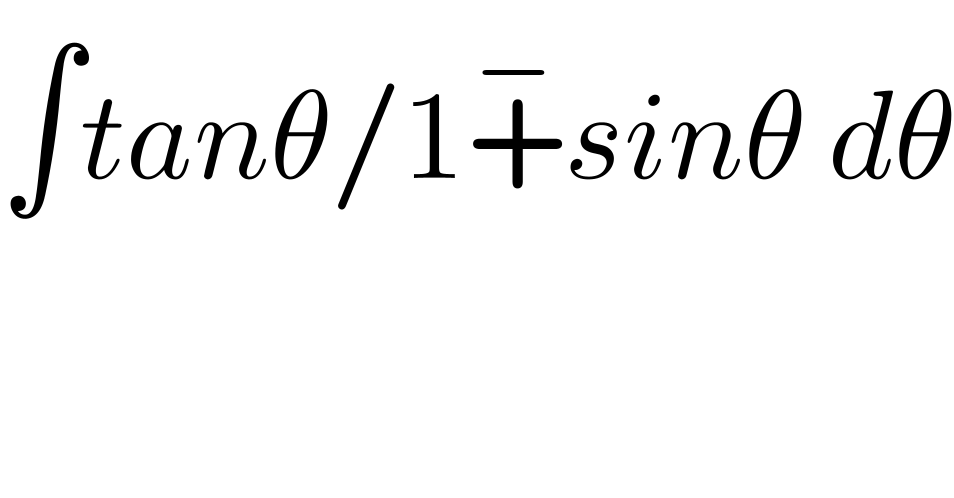
$$\int{tan}\theta/\mathrm{1}\overset{−} {+}{sin}\theta\:{d}\theta \\ $$
Commented by mr W last updated on 21/Jul/19

$${dear}\:{sir}: \\ $$$${in}\:{Q}\mathrm{64238}\:{i}\:{asked}\:{if}\:{you}\:{could}\:{use} \\ $$$${a}\:{smaller}\:{font}\:{such}\:{that}\:{your}\:{posts} \\ $$$${become}\:{better}\:{readable}\:{for}\:{other} \\ $$$${people}.\:{it}\:{seems}\:{that}\:{you}\:{wouldn}'{t} \\ $$$${change}\:{this},\:{what}\:{a}\:{pity}!\:{that}'{s}\:{ok}, \\ $$$${i}\:{don}'{t}\:{care}.\:{but}\:{i}\:{find}\:{it}\:{very}\:{unkind} \\ $$$${that}\:{you}\:{just}\:{ignore}\:{my}\:{kind}\:{request} \\ $$$${without}\:{any}\:{reply}.\:{i}\:{think},\:{being}\:{polite} \\ $$$${to}\:{each}\:{other}\:{is}\:{the}\:{foundation}\:{of} \\ $$$${this}\:{forum},\:{otherwise}\:{why}\:{should} \\ $$$${people}\:{help}\:{other}\:{people}? \\ $$
Commented by mathmax by abdo last updated on 21/Jul/19
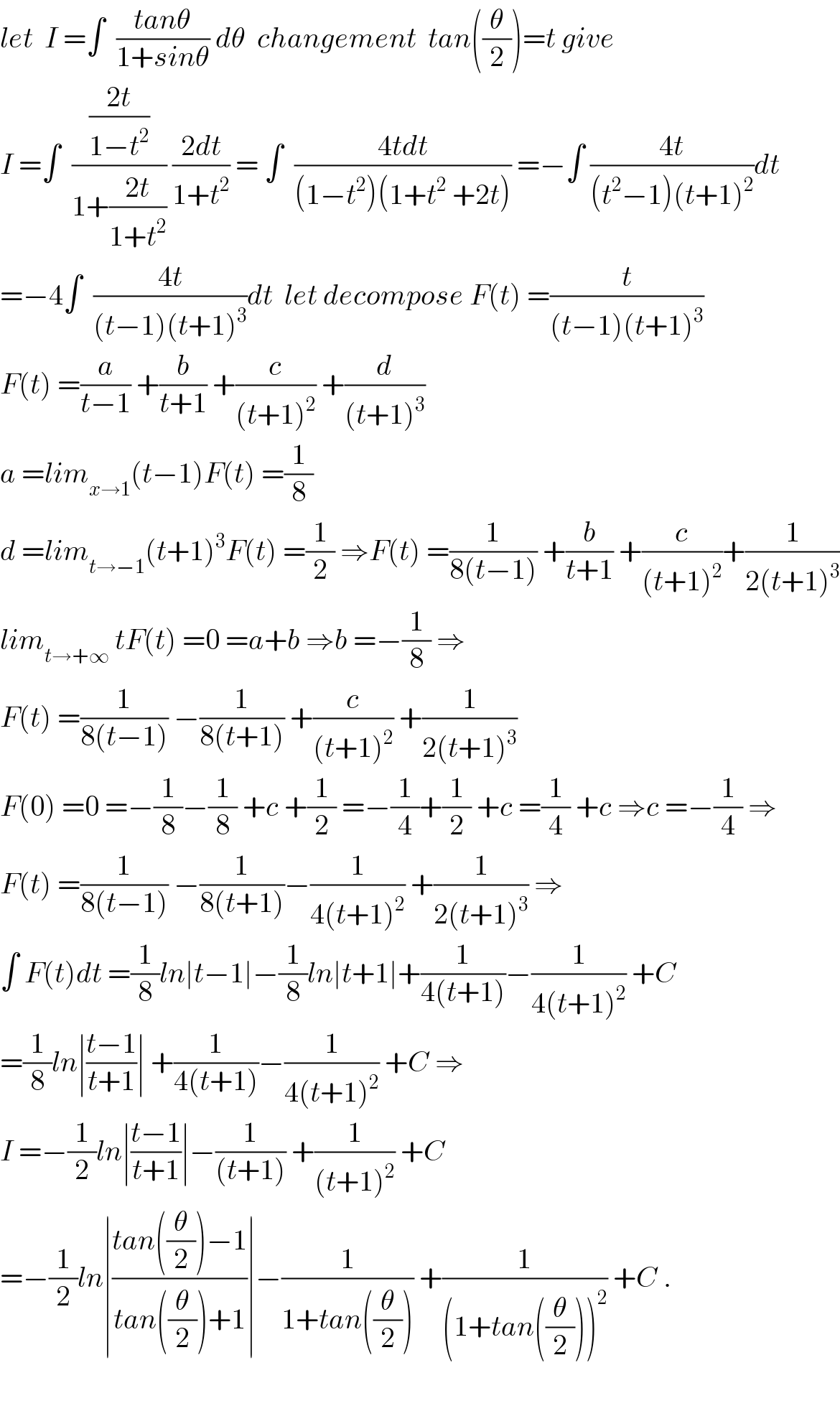
$${let}\:\:{I}\:=\int\:\:\frac{{tan}\theta}{\mathrm{1}+{sin}\theta}\:{d}\theta\:\:{changement}\:\:{tan}\left(\frac{\theta}{\mathrm{2}}\right)={t}\:{give} \\ $$$${I}\:=\int\:\:\frac{\frac{\mathrm{2}{t}}{\mathrm{1}−{t}^{\mathrm{2}} }}{\mathrm{1}+\frac{\mathrm{2}{t}}{\mathrm{1}+{t}^{\mathrm{2}} }}\:\frac{\mathrm{2}{dt}}{\mathrm{1}+{t}^{\mathrm{2}} }\:=\:\int\:\:\frac{\mathrm{4}{tdt}}{\left(\mathrm{1}−{t}^{\mathrm{2}} \right)\left(\mathrm{1}+{t}^{\mathrm{2}} \:+\mathrm{2}{t}\right)}\:=−\int\:\frac{\mathrm{4}{t}}{\left({t}^{\mathrm{2}} −\mathrm{1}\right)\left({t}+\mathrm{1}\right)^{\mathrm{2}} }{dt} \\ $$$$=−\mathrm{4}\int\:\:\frac{\mathrm{4}{t}}{\left({t}−\mathrm{1}\right)\left({t}+\mathrm{1}\right)^{\mathrm{3}} }{dt}\:\:{let}\:{decompose}\:{F}\left({t}\right)\:=\frac{{t}}{\left({t}−\mathrm{1}\right)\left({t}+\mathrm{1}\right)^{\mathrm{3}} } \\ $$$${F}\left({t}\right)\:=\frac{{a}}{{t}−\mathrm{1}}\:+\frac{{b}}{{t}+\mathrm{1}}\:+\frac{{c}}{\left({t}+\mathrm{1}\right)^{\mathrm{2}} }\:+\frac{{d}}{\left({t}+\mathrm{1}\right)^{\mathrm{3}} } \\ $$$${a}\:={lim}_{{x}\rightarrow\mathrm{1}} \left({t}−\mathrm{1}\right){F}\left({t}\right)\:=\frac{\mathrm{1}}{\mathrm{8}} \\ $$$${d}\:={lim}_{{t}\rightarrow−\mathrm{1}} \left({t}+\mathrm{1}\right)^{\mathrm{3}} {F}\left({t}\right)\:=\frac{\mathrm{1}}{\mathrm{2}}\:\Rightarrow{F}\left({t}\right)\:=\frac{\mathrm{1}}{\mathrm{8}\left({t}−\mathrm{1}\right)}\:+\frac{{b}}{{t}+\mathrm{1}}\:+\frac{{c}}{\left({t}+\mathrm{1}\right)^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{2}\left({t}+\mathrm{1}\right)^{\mathrm{3}} } \\ $$$${lim}_{{t}\rightarrow+\infty} \:{tF}\left({t}\right)\:=\mathrm{0}\:={a}+{b}\:\Rightarrow{b}\:=−\frac{\mathrm{1}}{\mathrm{8}}\:\Rightarrow \\ $$$${F}\left({t}\right)\:=\frac{\mathrm{1}}{\mathrm{8}\left({t}−\mathrm{1}\right)}\:−\frac{\mathrm{1}}{\mathrm{8}\left({t}+\mathrm{1}\right)}\:+\frac{{c}}{\left({t}+\mathrm{1}\right)^{\mathrm{2}} }\:+\frac{\mathrm{1}}{\mathrm{2}\left({t}+\mathrm{1}\right)^{\mathrm{3}} } \\ $$$${F}\left(\mathrm{0}\right)\:=\mathrm{0}\:=−\frac{\mathrm{1}}{\mathrm{8}}−\frac{\mathrm{1}}{\mathrm{8}}\:+{c}\:+\frac{\mathrm{1}}{\mathrm{2}}\:=−\frac{\mathrm{1}}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{2}}\:+{c}\:=\frac{\mathrm{1}}{\mathrm{4}}\:+{c}\:\Rightarrow{c}\:=−\frac{\mathrm{1}}{\mathrm{4}}\:\Rightarrow \\ $$$${F}\left({t}\right)\:=\frac{\mathrm{1}}{\mathrm{8}\left({t}−\mathrm{1}\right)}\:−\frac{\mathrm{1}}{\mathrm{8}\left({t}+\mathrm{1}\right)}−\frac{\mathrm{1}}{\mathrm{4}\left({t}+\mathrm{1}\right)^{\mathrm{2}} }\:+\frac{\mathrm{1}}{\mathrm{2}\left({t}+\mathrm{1}\right)^{\mathrm{3}} }\:\Rightarrow \\ $$$$\int\:{F}\left({t}\right){dt}\:=\frac{\mathrm{1}}{\mathrm{8}}{ln}\mid{t}−\mathrm{1}\mid−\frac{\mathrm{1}}{\mathrm{8}}{ln}\mid{t}+\mathrm{1}\mid+\frac{\mathrm{1}}{\mathrm{4}\left({t}+\mathrm{1}\right)}−\frac{\mathrm{1}}{\mathrm{4}\left({t}+\mathrm{1}\right)^{\mathrm{2}} }\:+{C} \\ $$$$=\frac{\mathrm{1}}{\mathrm{8}}{ln}\mid\frac{{t}−\mathrm{1}}{{t}+\mathrm{1}}\mid\:+\frac{\mathrm{1}}{\mathrm{4}\left({t}+\mathrm{1}\right)}−\frac{\mathrm{1}}{\mathrm{4}\left({t}+\mathrm{1}\right)^{\mathrm{2}} }\:+{C}\:\Rightarrow \\ $$$${I}\:=−\frac{\mathrm{1}}{\mathrm{2}}{ln}\mid\frac{{t}−\mathrm{1}}{{t}+\mathrm{1}}\mid−\frac{\mathrm{1}}{\left({t}+\mathrm{1}\right)}\:+\frac{\mathrm{1}}{\left({t}+\mathrm{1}\right)^{\mathrm{2}} }\:+{C} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{2}}{ln}\mid\frac{{tan}\left(\frac{\theta}{\mathrm{2}}\right)−\mathrm{1}}{{tan}\left(\frac{\theta}{\mathrm{2}}\right)+\mathrm{1}}\mid−\frac{\mathrm{1}}{\mathrm{1}+{tan}\left(\frac{\theta}{\mathrm{2}}\right)}\:+\frac{\mathrm{1}}{\left(\mathrm{1}+{tan}\left(\frac{\theta}{\mathrm{2}}\right)\right)^{\mathrm{2}} }\:+{C}\:. \\ $$$$ \\ $$
