
Question Number 64382 by Chi Mes Try last updated on 17/Jul/19
![please help with workings ∫Ln[(√)(1−x)+(√)(1+x)]dx](Q64382.png)
$${please}\:\:{help}\:{with}\:{workings} \\ $$$$ \\ $$$$\int{Ln}\left[\sqrt{}\left(\mathrm{1}−{x}\right)+\sqrt{}\left(\mathrm{1}+{x}\right)\right]{dx} \\ $$
Answered by MJS last updated on 17/Jul/19
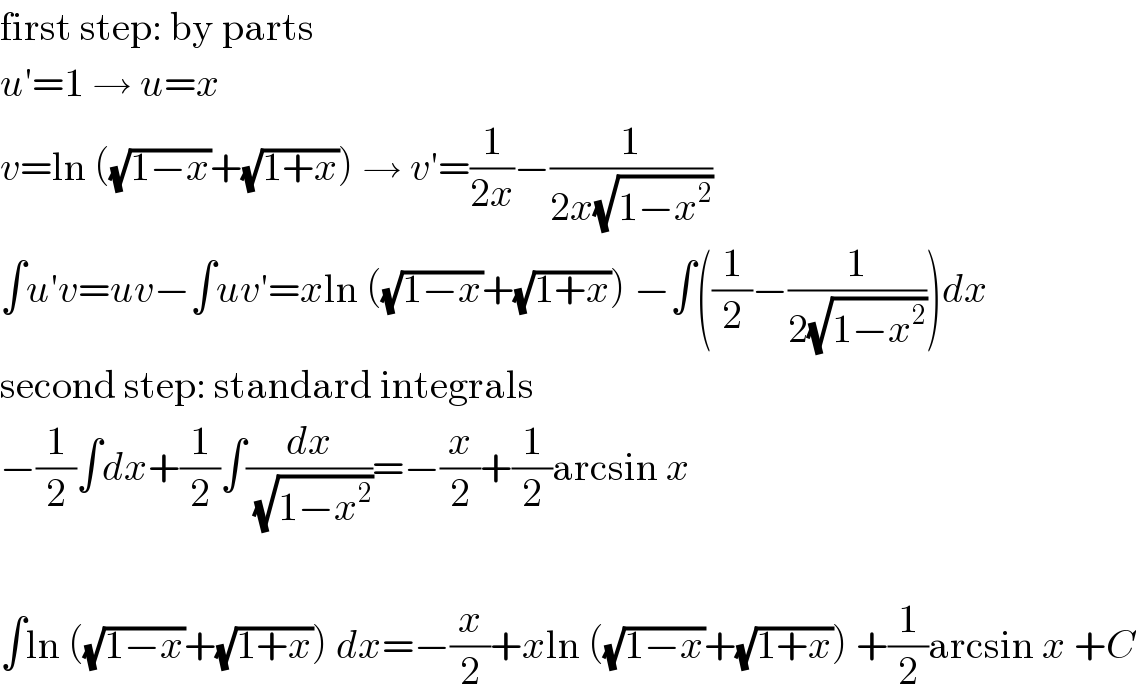
$$\mathrm{first}\:\mathrm{step}:\:\mathrm{by}\:\mathrm{parts} \\ $$$${u}'=\mathrm{1}\:\rightarrow\:{u}={x} \\ $$$${v}=\mathrm{ln}\:\left(\sqrt{\mathrm{1}−{x}}+\sqrt{\mathrm{1}+{x}}\right)\:\rightarrow\:{v}'=\frac{\mathrm{1}}{\mathrm{2}{x}}−\frac{\mathrm{1}}{\mathrm{2}{x}\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }} \\ $$$$\int{u}'{v}={uv}−\int{uv}'={x}\mathrm{ln}\:\left(\sqrt{\mathrm{1}−{x}}+\sqrt{\mathrm{1}+{x}}\right)\:−\int\left(\frac{\mathrm{1}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}\right){dx} \\ $$$$\mathrm{second}\:\mathrm{step}:\:\mathrm{standard}\:\mathrm{integrals} \\ $$$$−\frac{\mathrm{1}}{\mathrm{2}}\int{dx}+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{dx}}{\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}=−\frac{{x}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{arcsin}\:{x} \\ $$$$ \\ $$$$\int\mathrm{ln}\:\left(\sqrt{\mathrm{1}−{x}}+\sqrt{\mathrm{1}+{x}}\right)\:{dx}=−\frac{{x}}{\mathrm{2}}+{x}\mathrm{ln}\:\left(\sqrt{\mathrm{1}−{x}}+\sqrt{\mathrm{1}+{x}}\right)\:+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{arcsin}\:{x}\:+{C} \\ $$
