
Question Number 63720 by mathmax by abdo last updated on 08/Jul/19
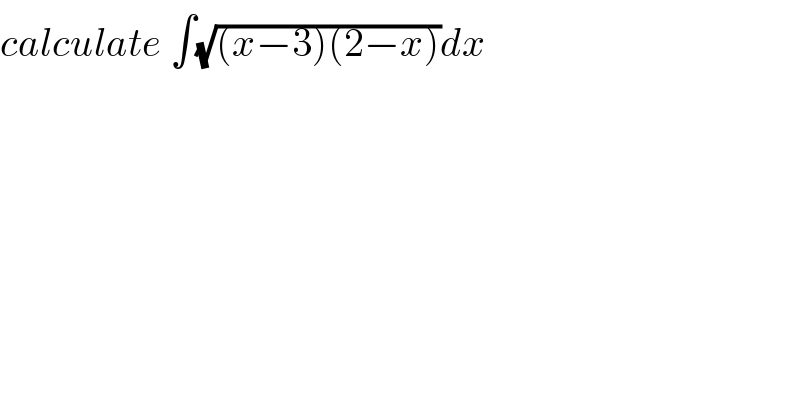
$${calculate}\:\int\sqrt{\left({x}−\mathrm{3}\right)\left(\mathrm{2}−{x}\right)}{dx} \\ $$
Commented by Prithwish sen last updated on 08/Jul/19
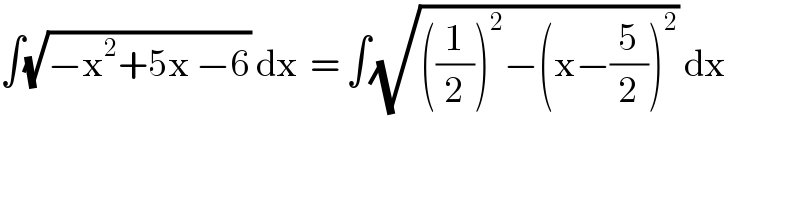
$$\int\sqrt{−\mathrm{x}^{\mathrm{2}} +\mathrm{5x}\:−\mathrm{6}}\:\mathrm{dx}\:\:=\:\int\sqrt{\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} −\left(\mathrm{x}−\frac{\mathrm{5}}{\mathrm{2}}\right)^{\mathrm{2}} }\:\mathrm{dx} \\ $$
Commented by mathmax by abdo last updated on 08/Jul/19
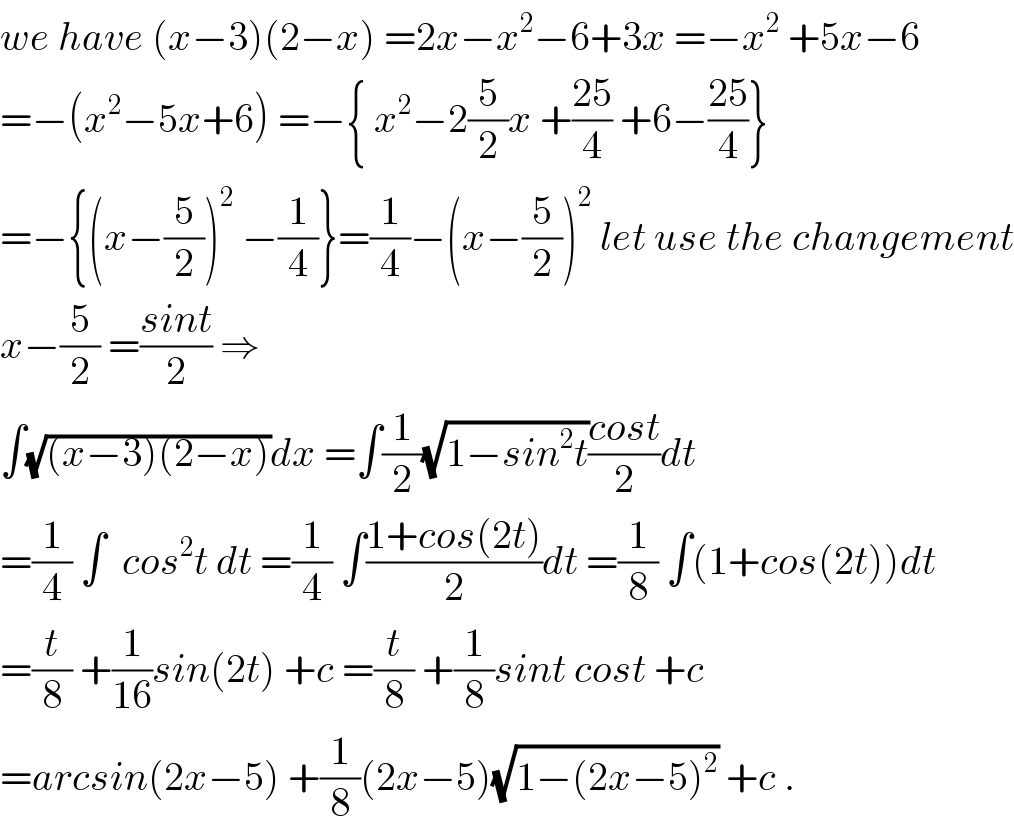
$${we}\:{have}\:\left({x}−\mathrm{3}\right)\left(\mathrm{2}−{x}\right)\:=\mathrm{2}{x}−{x}^{\mathrm{2}} −\mathrm{6}+\mathrm{3}{x}\:=−{x}^{\mathrm{2}} \:+\mathrm{5}{x}−\mathrm{6} \\ $$$$=−\left({x}^{\mathrm{2}} −\mathrm{5}{x}+\mathrm{6}\right)\:=−\left\{\:{x}^{\mathrm{2}} −\mathrm{2}\frac{\mathrm{5}}{\mathrm{2}}{x}\:+\frac{\mathrm{25}}{\mathrm{4}}\:+\mathrm{6}−\frac{\mathrm{25}}{\mathrm{4}}\right\} \\ $$$$=−\left\{\left({x}−\frac{\mathrm{5}}{\mathrm{2}}\right)^{\mathrm{2}} \:−\frac{\mathrm{1}}{\mathrm{4}}\right\}=\frac{\mathrm{1}}{\mathrm{4}}−\left({x}−\frac{\mathrm{5}}{\mathrm{2}}\right)^{\mathrm{2}} \:{let}\:{use}\:{the}\:{changement} \\ $$$${x}−\frac{\mathrm{5}}{\mathrm{2}}\:=\frac{{sint}}{\mathrm{2}}\:\Rightarrow \\ $$$$\int\sqrt{\left({x}−\mathrm{3}\right)\left(\mathrm{2}−{x}\right)}{dx}\:=\int\frac{\mathrm{1}}{\mathrm{2}}\sqrt{\mathrm{1}−{sin}^{\mathrm{2}} {t}}\frac{{cost}}{\mathrm{2}}{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}\:\int\:\:{cos}^{\mathrm{2}} {t}\:{dt}\:=\frac{\mathrm{1}}{\mathrm{4}}\:\int\frac{\mathrm{1}+{cos}\left(\mathrm{2}{t}\right)}{\mathrm{2}}{dt}\:=\frac{\mathrm{1}}{\mathrm{8}}\:\int\left(\mathrm{1}+{cos}\left(\mathrm{2}{t}\right)\right){dt} \\ $$$$=\frac{{t}}{\mathrm{8}}\:+\frac{\mathrm{1}}{\mathrm{16}}{sin}\left(\mathrm{2}{t}\right)\:+{c}\:=\frac{{t}}{\mathrm{8}}\:+\frac{\mathrm{1}}{\mathrm{8}}{sint}\:{cost}\:+{c} \\ $$$$={arcsin}\left(\mathrm{2}{x}−\mathrm{5}\right)\:+\frac{\mathrm{1}}{\mathrm{8}}\left(\mathrm{2}{x}−\mathrm{5}\right)\sqrt{\mathrm{1}−\left(\mathrm{2}{x}−\mathrm{5}\right)^{\mathrm{2}} }\:+{c}\:. \\ $$
Answered by MJS last updated on 08/Jul/19
![∫(√((x−3)(2−x)))dx= [t=arccos (2x−5) → dx=−(√((x−3)(2−x)))dt] =−(1/4)∫sin^2 t dt=−(1/8)∫dt+(1/8)∫cos 2t dt= =−(1/8)t+(1/(16))sin 2t =−(1/8)t+(1/8)sin t cos t = =−(1/8)arccos (2x−5) +(1/4)(2x−5)(√((x−3)(2−x)))+C](Q63735.png)
$$\int\sqrt{\left({x}−\mathrm{3}\right)\left(\mathrm{2}−{x}\right)}{dx}= \\ $$$$\:\:\:\:\:\left[{t}=\mathrm{arccos}\:\left(\mathrm{2}{x}−\mathrm{5}\right)\:\rightarrow\:{dx}=−\sqrt{\left({x}−\mathrm{3}\right)\left(\mathrm{2}−{x}\right)}{dt}\right] \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}}\int\mathrm{sin}^{\mathrm{2}} \:{t}\:{dt}=−\frac{\mathrm{1}}{\mathrm{8}}\int{dt}+\frac{\mathrm{1}}{\mathrm{8}}\int\mathrm{cos}\:\mathrm{2}{t}\:{dt}= \\ $$$$=−\frac{\mathrm{1}}{\mathrm{8}}{t}+\frac{\mathrm{1}}{\mathrm{16}}\mathrm{sin}\:\mathrm{2}{t}\:=−\frac{\mathrm{1}}{\mathrm{8}}{t}+\frac{\mathrm{1}}{\mathrm{8}}\mathrm{sin}\:{t}\:\mathrm{cos}\:{t}\:= \\ $$$$=−\frac{\mathrm{1}}{\mathrm{8}}\mathrm{arccos}\:\left(\mathrm{2}{x}−\mathrm{5}\right)\:+\frac{\mathrm{1}}{\mathrm{4}}\left(\mathrm{2}{x}−\mathrm{5}\right)\sqrt{\left({x}−\mathrm{3}\right)\left(\mathrm{2}−{x}\right)}+{C} \\ $$
