
Question Number 63021 by kaivan.ahmadi last updated on 27/Jun/19
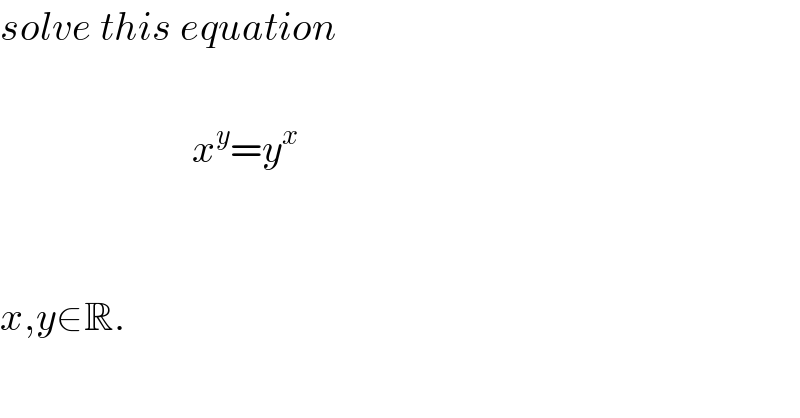
$${solve}\:{this}\:{equation} \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{x}^{{y}} ={y}^{{x}} \\ $$$$ \\ $$$$ \\ $$$${x},{y}\in\mathbb{R}. \\ $$
Commented by MJS last updated on 28/Jun/19

$${x}={y}\:\forall{x},\:{y}\:\in\mathbb{C}\backslash\mathrm{0} \\ $$
Commented by kaivan.ahmadi last updated on 28/Jun/19
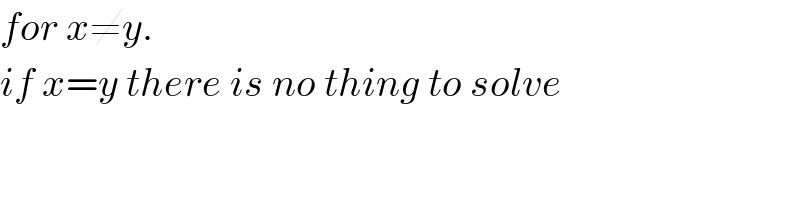
$${for}\:{x}\neq{y}. \\ $$$${if}\:{x}={y}\:{there}\:{is}\:{no}\:{thing}\:{to}\:{solve} \\ $$
Commented by malwaan last updated on 28/Jun/19

$$\left\{\mathrm{2}\:;\:\mathrm{4}\right\} \\ $$$$\mathrm{2}^{\mathrm{4}} \:=\:\mathrm{4}^{\mathrm{2}} \:=\mathrm{16} \\ $$
Answered by MJS last updated on 28/Jun/19
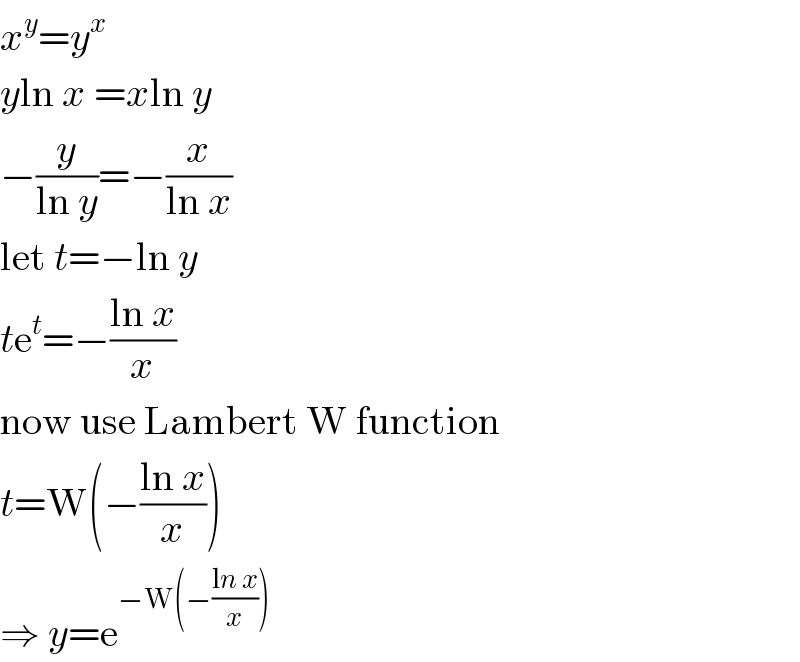
$${x}^{{y}} ={y}^{{x}} \\ $$$${y}\mathrm{ln}\:{x}\:={x}\mathrm{ln}\:{y} \\ $$$$−\frac{{y}}{\mathrm{ln}\:{y}}=−\frac{{x}}{\mathrm{ln}\:{x}} \\ $$$$\mathrm{let}\:{t}=−\mathrm{ln}\:{y} \\ $$$${t}\mathrm{e}^{{t}} =−\frac{\mathrm{ln}\:{x}}{{x}} \\ $$$$\mathrm{now}\:\mathrm{use}\:\mathrm{Lambert}\:\mathrm{W}\:\mathrm{function} \\ $$$${t}=\mathrm{W}\left(−\frac{\mathrm{ln}\:{x}}{{x}}\right) \\ $$$$\Rightarrow\:{y}=\mathrm{e}^{−\mathrm{W}\left(−\frac{\mathrm{l}{n}\:{x}}{{x}}\right)} \\ $$
Commented by MJS last updated on 28/Jun/19
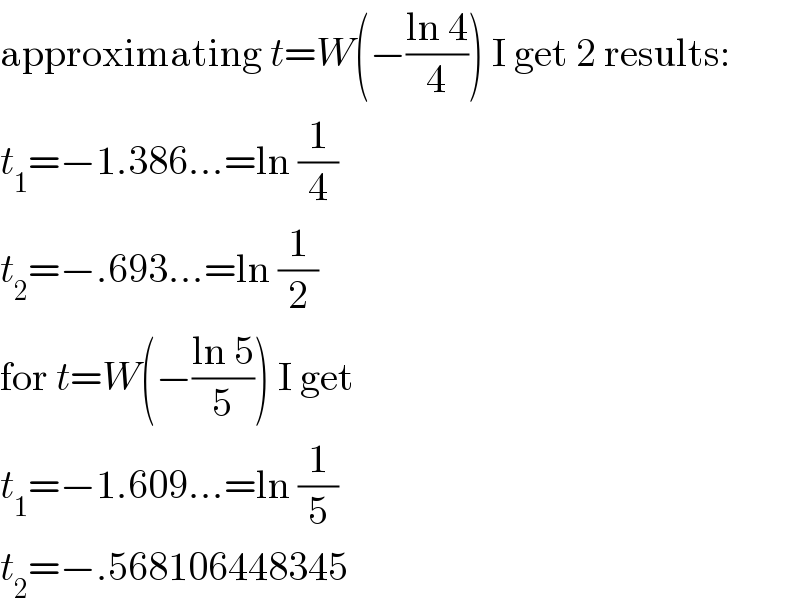
$$\mathrm{approximating}\:{t}={W}\left(−\frac{\mathrm{ln}\:\mathrm{4}}{\mathrm{4}}\right)\:\mathrm{I}\:\mathrm{get}\:\mathrm{2}\:\mathrm{results}: \\ $$$${t}_{\mathrm{1}} =−\mathrm{1}.\mathrm{386}...=\mathrm{ln}\:\frac{\mathrm{1}}{\mathrm{4}} \\ $$$${t}_{\mathrm{2}} =−.\mathrm{693}...=\mathrm{ln}\:\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\mathrm{for}\:{t}={W}\left(−\frac{\mathrm{ln}\:\mathrm{5}}{\mathrm{5}}\right)\:\mathrm{I}\:\mathrm{get} \\ $$$${t}_{\mathrm{1}} =−\mathrm{1}.\mathrm{609}...=\mathrm{ln}\:\frac{\mathrm{1}}{\mathrm{5}} \\ $$$${t}_{\mathrm{2}} =−.\mathrm{568106448345} \\ $$
Commented by mr W last updated on 28/Jun/19

$${good}\:{idea}\:{sir}!\:{but}\:{the}\:{last}\:{line}\:{is}\:{the} \\ $$$${same}\:{as}\:{y}={x},\:{and}\:{this}\:{is}\:{only}\:{one} \\ $$$${possible}\:{case}. \\ $$$${note}: \\ $$$${W}\left(−\frac{\mathrm{ln}\:{x}}{{x}}\right)=−\mathrm{ln}\:{x} \\ $$$${y}={e}^{−{W}\left(−\frac{\mathrm{ln}\:{x}}{{x}}\right)} ={e}^{\mathrm{ln}\:{x}} ={x} \\ $$
Commented by mr W last updated on 28/Jun/19
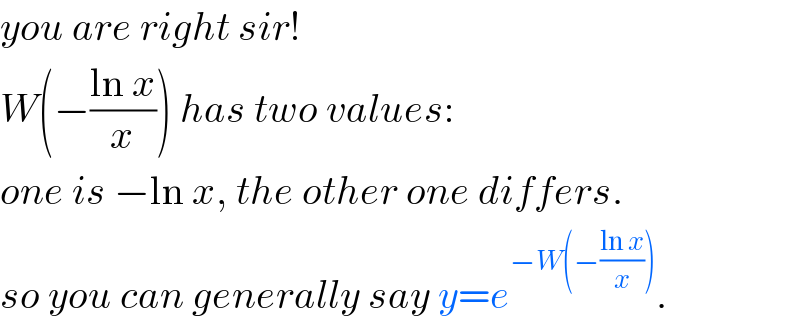
$${you}\:{are}\:{right}\:{sir}! \\ $$$${W}\left(−\frac{\mathrm{ln}\:{x}}{{x}}\right)\:{has}\:{two}\:{values}:\: \\ $$$${one}\:{is}\:−\mathrm{ln}\:{x},\:{the}\:{other}\:{one}\:{differs}. \\ $$$${so}\:{you}\:{can}\:{generally}\:{say}\:{y}={e}^{−{W}\left(−\frac{\mathrm{ln}\:{x}}{{x}}\right)} . \\ $$
Answered by mr W last updated on 28/Jun/19
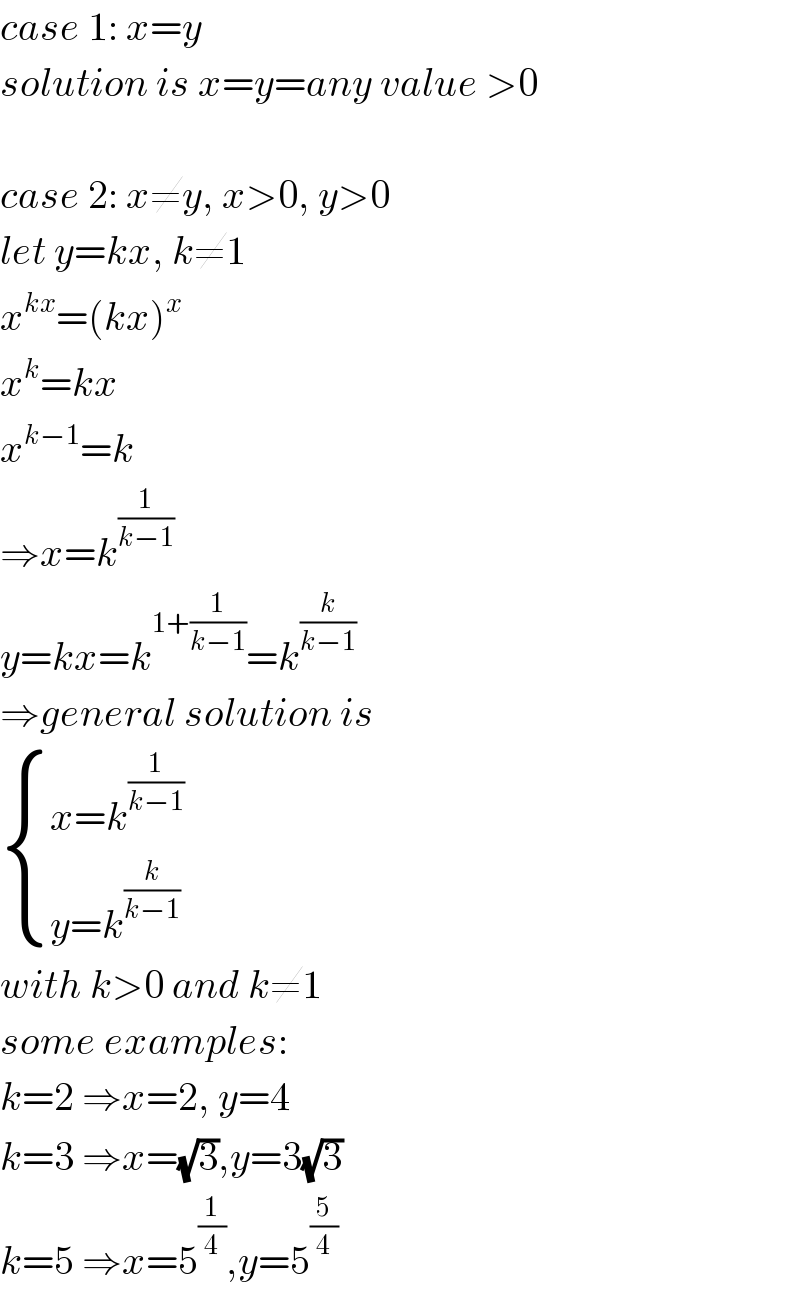
$${case}\:\mathrm{1}:\:{x}={y} \\ $$$${solution}\:{is}\:{x}={y}={any}\:{value}\:>\mathrm{0} \\ $$$$ \\ $$$${case}\:\mathrm{2}:\:{x}\neq{y},\:{x}>\mathrm{0},\:{y}>\mathrm{0} \\ $$$${let}\:{y}={kx},\:{k}\neq\mathrm{1} \\ $$$${x}^{{kx}} =\left({kx}\right)^{{x}} \\ $$$${x}^{{k}} ={kx} \\ $$$${x}^{{k}−\mathrm{1}} ={k} \\ $$$$\Rightarrow{x}={k}^{\frac{\mathrm{1}}{{k}−\mathrm{1}}} \\ $$$${y}={kx}={k}^{\mathrm{1}+\frac{\mathrm{1}}{{k}−\mathrm{1}}} ={k}^{\frac{{k}}{{k}−\mathrm{1}}} \\ $$$$\Rightarrow{general}\:{solution}\:{is} \\ $$$$\begin{cases}{{x}={k}^{\frac{\mathrm{1}}{{k}−\mathrm{1}}} }\\{{y}={k}^{\frac{{k}}{{k}−\mathrm{1}}} }\end{cases} \\ $$$${with}\:{k}>\mathrm{0}\:{and}\:{k}\neq\mathrm{1} \\ $$$${some}\:{examples}: \\ $$$${k}=\mathrm{2}\:\Rightarrow{x}=\mathrm{2},\:{y}=\mathrm{4} \\ $$$${k}=\mathrm{3}\:\Rightarrow{x}=\sqrt{\mathrm{3}},{y}=\mathrm{3}\sqrt{\mathrm{3}} \\ $$$${k}=\mathrm{5}\:\Rightarrow{x}=\mathrm{5}^{\frac{\mathrm{1}}{\mathrm{4}}} ,{y}=\mathrm{5}^{\frac{\mathrm{5}}{\mathrm{4}}} \\ $$
Commented by MJS last updated on 28/Jun/19

$${x}^{\mathrm{4}} =\mathrm{4}^{{x}} \:\mathrm{has}\:\mathrm{got}\:\mathrm{3}\:\mathrm{solutions} \\ $$$${x}=\mathrm{2}\vee{x}=\mathrm{4}\vee{x}\approx−.\mathrm{766664696} \\ $$$$\mathrm{how}\:\mathrm{to}\:\mathrm{get}\:\mathrm{the}\:\mathrm{last}\:\mathrm{one}? \\ $$
Commented by kaivan.ahmadi last updated on 28/Jun/19
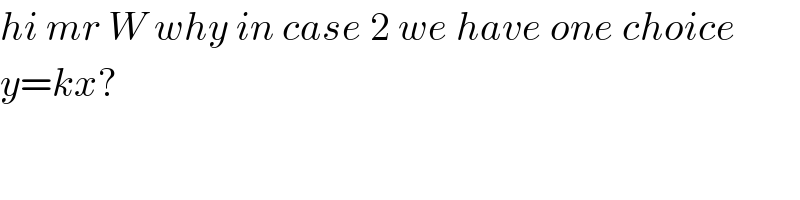
$${hi}\:{mr}\:{W}\:{why}\:{in}\:{case}\:\mathrm{2}\:{we}\:{have}\:{one}\:{choice} \\ $$$${y}={kx}? \\ $$$$ \\ $$
Commented by kaivan.ahmadi last updated on 28/Jun/19
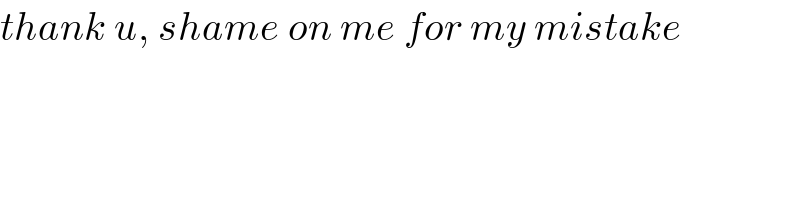
$${thank}\:{u},\:{shame}\:{on}\:{me}\:{for}\:{my}\:{mistake} \\ $$
Commented by MJS last updated on 28/Jun/19

$${x}^{{kx}} =\left({kx}\right)^{{x}} \\ $$$$\sqrt[{{x}}]{{x}^{{kx}} }=\sqrt[{{x}}]{\left({kx}\right)^{{x}} } \\ $$$${x}^{{k}} ={kx} \\ $$
Commented by MJS last updated on 28/Jun/19

$${x}^{{kx}} \neq{x}^{{k}} {x}^{{x}} !!! \\ $$$${x}^{{kx}} =\left({x}^{{k}} \right)^{{x}} =\left({x}^{{x}} \right)^{{k}} \\ $$
Commented by MJS last updated on 28/Jun/19

$$\mathrm{from}\:\mathrm{mistakes}\:\mathrm{we}\:\mathrm{learn} \\ $$$$\mathrm{I}\:\mathrm{didn}'\mathrm{t}\:\mathrm{immediately}\:\mathrm{see}\:\mathrm{it},\:\mathrm{so}\:\mathrm{shame}\:\mathrm{on}\:\mathrm{me}\:\mathrm{too} \\ $$
Commented by mr W last updated on 28/Jun/19

$${MJS}\:{sir}: \\ $$$${when}\:{we}\:{treat}\:{the}\:{particular}\:{equation} \\ $$$${x}^{\mathrm{4}} =\mathrm{4}^{{x}} ,\:{it}\:{has}\:{indeed}\:{three}\:{roots},\:{one} \\ $$$${of}\:{them}\:{is}\:{negative}.\:{but}\:{when}\:{we} \\ $$$${treat}\:{generaly}\:{x}^{{y}} ={y}^{{x}} ,\:{then}\:{the}\:{domain}\:{of} \\ $$$${solution}\:{is}\:{x}>\mathrm{0}\:{and}\:{y}>\mathrm{0}.\:\:{you}\:{see}\:{this} \\ $$$${if}\:{the}\:{particular}\:{equation}\:{is} \\ $$$${x}^{\mathrm{1}.\mathrm{25}} =\mathrm{1}.\mathrm{25}^{{x}} \:{which}\:{has}\:{only}\:{two}\:{roots}, \\ $$$${since}\:{x}>\mathrm{0}. \\ $$
Commented by MJS last updated on 28/Jun/19
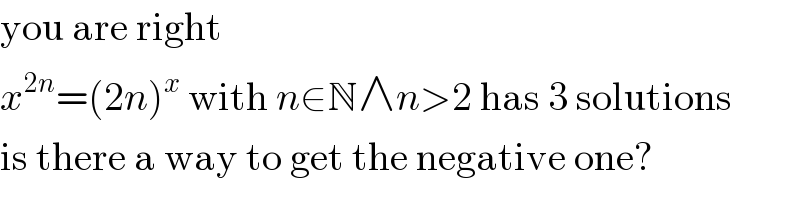
$$\mathrm{you}\:\mathrm{are}\:\mathrm{right} \\ $$$${x}^{\mathrm{2}{n}} =\left(\mathrm{2}{n}\right)^{{x}} \:\mathrm{with}\:{n}\in\mathbb{N}\wedge{n}>\mathrm{2}\:\mathrm{has}\:\mathrm{3}\:\mathrm{solutions} \\ $$$$\mathrm{is}\:\mathrm{there}\:\mathrm{a}\:\mathrm{way}\:\mathrm{to}\:\mathrm{get}\:\mathrm{the}\:\mathrm{negative}\:\mathrm{one}? \\ $$
Commented by mr W last updated on 28/Jun/19
![yes! x^(2n) =(2n)^x ⇒x=±(2n)^(x/(2n)) =±e^((x ln (2n))/(2n)) ⇒xe^(−((x ln (2n))/(2n))) =±1 ⇒[−((x ln (2n))/(2n))]e^([−((x ln (2n))/(2n))]) =±((ln (2n))/(2n)) ⇒−((x ln (2n))/(2n))=W[±((ln (2n))/(2n))] ⇒x=−((2n)/(ln (2n)))W[±((ln (2n))/(2n))] this gets three values, one of them is 2n. one of them is negative.](Q63066.png)
$${yes}! \\ $$$${x}^{\mathrm{2}{n}} =\left(\mathrm{2}{n}\right)^{{x}} \\ $$$$\Rightarrow{x}=\pm\left(\mathrm{2}{n}\right)^{\frac{{x}}{\mathrm{2}{n}}} =\pm{e}^{\frac{{x}\:\mathrm{ln}\:\left(\mathrm{2}{n}\right)}{\mathrm{2}{n}}} \\ $$$$\Rightarrow{xe}^{−\frac{{x}\:\mathrm{ln}\:\left(\mathrm{2}{n}\right)}{\mathrm{2}{n}}} =\pm\mathrm{1} \\ $$$$\Rightarrow\left[−\frac{{x}\:\mathrm{ln}\:\left(\mathrm{2}{n}\right)}{\mathrm{2}{n}}\right]{e}^{\left[−\frac{{x}\:\mathrm{ln}\:\left(\mathrm{2}{n}\right)}{\mathrm{2}{n}}\right]} =\pm\frac{\mathrm{ln}\:\left(\mathrm{2}{n}\right)}{\mathrm{2}{n}} \\ $$$$\Rightarrow−\frac{{x}\:\mathrm{ln}\:\left(\mathrm{2}{n}\right)}{\mathrm{2}{n}}={W}\left[\pm\frac{\mathrm{ln}\:\left(\mathrm{2}{n}\right)}{\mathrm{2}{n}}\right] \\ $$$$\Rightarrow{x}=−\frac{\mathrm{2}{n}}{\mathrm{ln}\:\left(\mathrm{2}{n}\right)}{W}\left[\pm\frac{\mathrm{ln}\:\left(\mathrm{2}{n}\right)}{\mathrm{2}{n}}\right] \\ $$$${this}\:{gets}\:{three}\:{values},\:{one}\:{of}\:{them}\:{is}\:\mathrm{2}{n}. \\ $$$${one}\:{of}\:{them}\:{is}\:{negative}. \\ $$
Commented by MJS last updated on 28/Jun/19

$$\mathrm{thank}\:\mathrm{you}! \\ $$
