
Question Number 62895 by Tawa1 last updated on 26/Jun/19
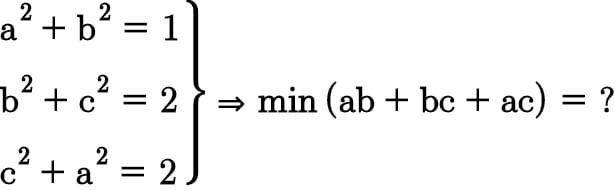
Commented by Tony Lin last updated on 26/Jun/19
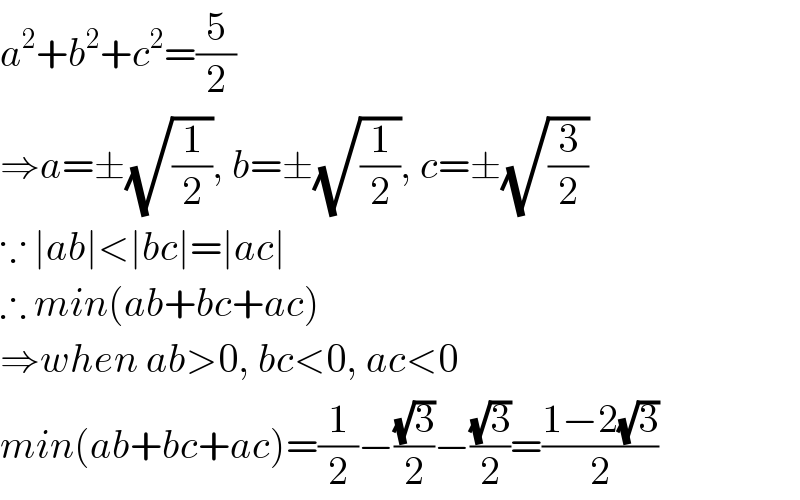
$${a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} =\frac{\mathrm{5}}{\mathrm{2}} \\ $$$$\Rightarrow{a}=\pm\sqrt{\frac{\mathrm{1}}{\mathrm{2}}},\:{b}=\pm\sqrt{\frac{\mathrm{1}}{\mathrm{2}}},\:{c}=\pm\sqrt{\frac{\mathrm{3}}{\mathrm{2}}} \\ $$$$\because\:\mid{ab}\mid<\mid{bc}\mid=\mid{ac}\mid \\ $$$$\therefore\:{min}\left({ab}+{bc}+{ac}\right) \\ $$$$\Rightarrow{when}\:{ab}>\mathrm{0},\:{bc}<\mathrm{0},\:{ac}<\mathrm{0} \\ $$$${min}\left({ab}+{bc}+{ac}\right)=\frac{\mathrm{1}}{\mathrm{2}}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}=\frac{\mathrm{1}−\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$
Commented by Tawa1 last updated on 26/Jun/19
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
Answered by Kunal12588 last updated on 26/Jun/19
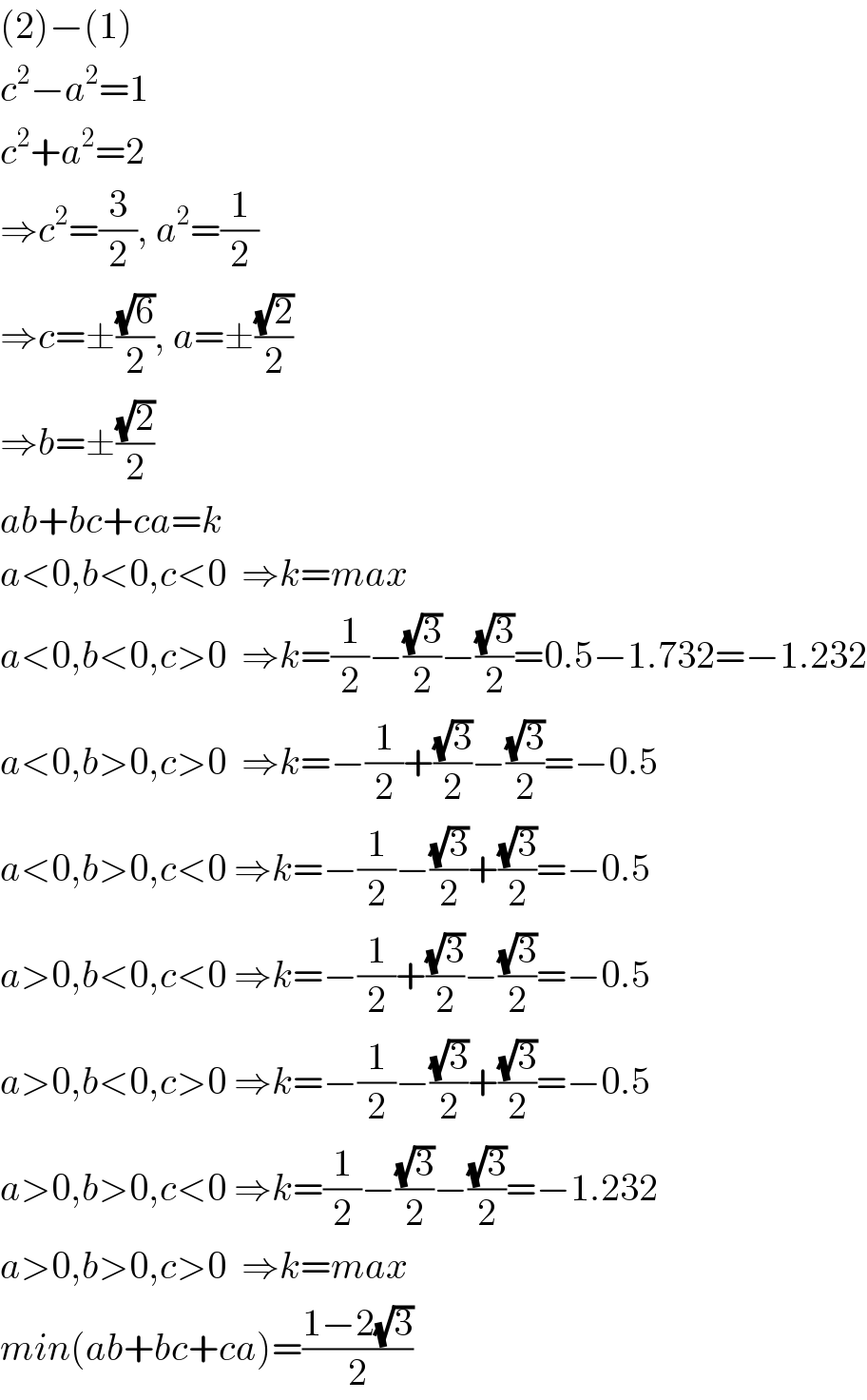
$$\left(\mathrm{2}\right)−\left(\mathrm{1}\right) \\ $$$${c}^{\mathrm{2}} −{a}^{\mathrm{2}} =\mathrm{1} \\ $$$${c}^{\mathrm{2}} +{a}^{\mathrm{2}} =\mathrm{2} \\ $$$$\Rightarrow{c}^{\mathrm{2}} =\frac{\mathrm{3}}{\mathrm{2}},\:{a}^{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\Rightarrow{c}=\pm\frac{\sqrt{\mathrm{6}}}{\mathrm{2}},\:{a}=\pm\frac{\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$$$\Rightarrow{b}=\pm\frac{\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$$${ab}+{bc}+{ca}={k} \\ $$$${a}<\mathrm{0},{b}<\mathrm{0},{c}<\mathrm{0}\:\:\Rightarrow{k}={max} \\ $$$${a}<\mathrm{0},{b}<\mathrm{0},{c}>\mathrm{0}\:\:\Rightarrow{k}=\frac{\mathrm{1}}{\mathrm{2}}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}=\mathrm{0}.\mathrm{5}−\mathrm{1}.\mathrm{732}=−\mathrm{1}.\mathrm{232} \\ $$$${a}<\mathrm{0},{b}>\mathrm{0},{c}>\mathrm{0}\:\:\Rightarrow{k}=−\frac{\mathrm{1}}{\mathrm{2}}+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}=−\mathrm{0}.\mathrm{5} \\ $$$${a}<\mathrm{0},{b}>\mathrm{0},{c}<\mathrm{0}\:\Rightarrow{k}=−\frac{\mathrm{1}}{\mathrm{2}}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}=−\mathrm{0}.\mathrm{5} \\ $$$${a}>\mathrm{0},{b}<\mathrm{0},{c}<\mathrm{0}\:\Rightarrow{k}=−\frac{\mathrm{1}}{\mathrm{2}}+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}=−\mathrm{0}.\mathrm{5} \\ $$$${a}>\mathrm{0},{b}<\mathrm{0},{c}>\mathrm{0}\:\Rightarrow{k}=−\frac{\mathrm{1}}{\mathrm{2}}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}=−\mathrm{0}.\mathrm{5} \\ $$$${a}>\mathrm{0},{b}>\mathrm{0},{c}<\mathrm{0}\:\Rightarrow{k}=\frac{\mathrm{1}}{\mathrm{2}}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}=−\mathrm{1}.\mathrm{232} \\ $$$${a}>\mathrm{0},{b}>\mathrm{0},{c}>\mathrm{0}\:\:\Rightarrow{k}={max} \\ $$$${min}\left({ab}+{bc}+{ca}\right)=\frac{\mathrm{1}−\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$
Commented by Tawa1 last updated on 26/Jun/19
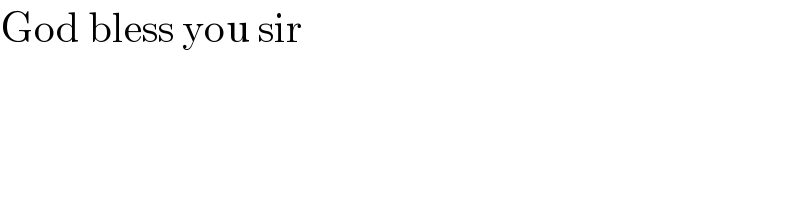
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
