
Question Number 62363 by Tawa1 last updated on 20/Jun/19

Answered by MJS last updated on 20/Jun/19
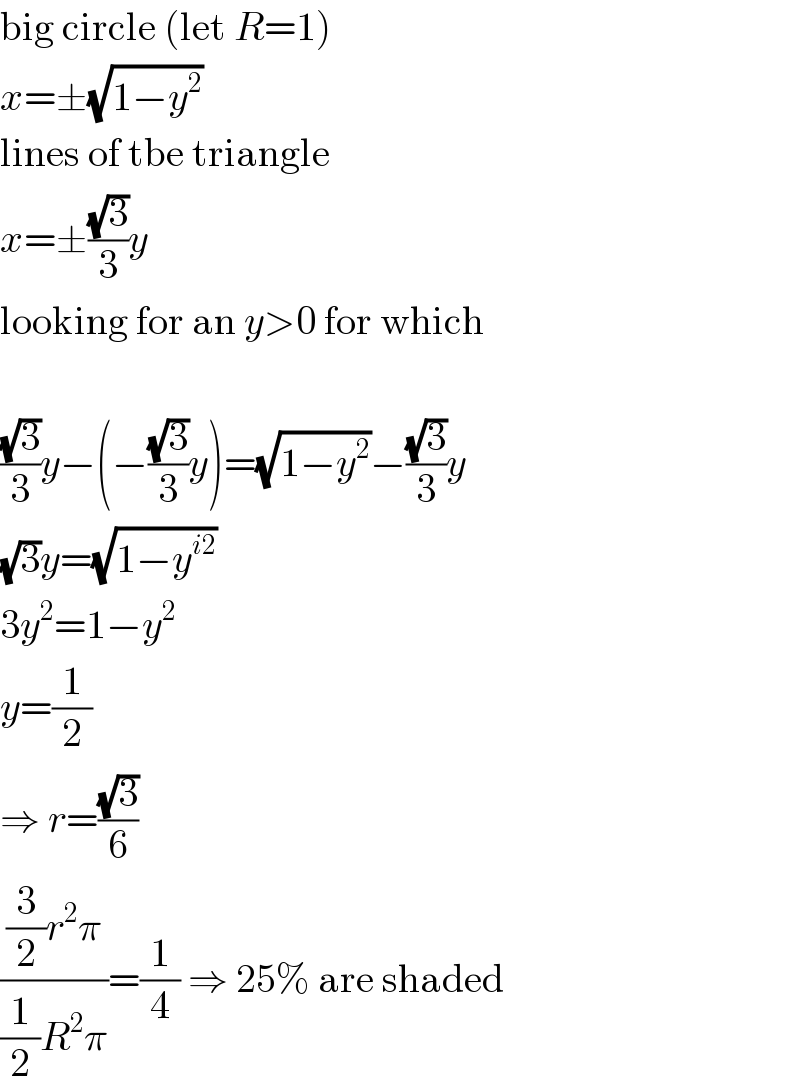
$$\mathrm{big}\:\mathrm{circle}\:\left(\mathrm{let}\:{R}=\mathrm{1}\right) \\ $$$${x}=\pm\sqrt{\mathrm{1}−{y}^{\mathrm{2}} } \\ $$$$\mathrm{lines}\:\mathrm{of}\:\mathrm{tbe}\:\mathrm{triangle} \\ $$$${x}=\pm\frac{\sqrt{\mathrm{3}}}{\mathrm{3}}{y} \\ $$$$\mathrm{looking}\:\mathrm{for}\:\mathrm{an}\:{y}>\mathrm{0}\:\mathrm{for}\:\mathrm{which} \\ $$$$ \\ $$$$\frac{\sqrt{\mathrm{3}}}{\mathrm{3}}{y}−\left(−\frac{\sqrt{\mathrm{3}}}{\mathrm{3}}{y}\right)=\sqrt{\mathrm{1}−{y}^{\mathrm{2}} }−\frac{\sqrt{\mathrm{3}}}{\mathrm{3}}{y} \\ $$$$\sqrt{\mathrm{3}}{y}=\sqrt{\mathrm{1}−{y}^{{i}\mathrm{2}} } \\ $$$$\mathrm{3}{y}^{\mathrm{2}} =\mathrm{1}−{y}^{\mathrm{2}} \\ $$$${y}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\Rightarrow\:{r}=\frac{\sqrt{\mathrm{3}}}{\mathrm{6}} \\ $$$$\frac{\frac{\mathrm{3}}{\mathrm{2}}{r}^{\mathrm{2}} \pi}{\frac{\mathrm{1}}{\mathrm{2}}{R}^{\mathrm{2}} \pi}=\frac{\mathrm{1}}{\mathrm{4}}\:\Rightarrow\:\mathrm{25\%}\:\mathrm{are}\:\mathrm{shaded} \\ $$
Commented by Tawa1 last updated on 20/Jun/19
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
Answered by mr W last updated on 20/Jun/19
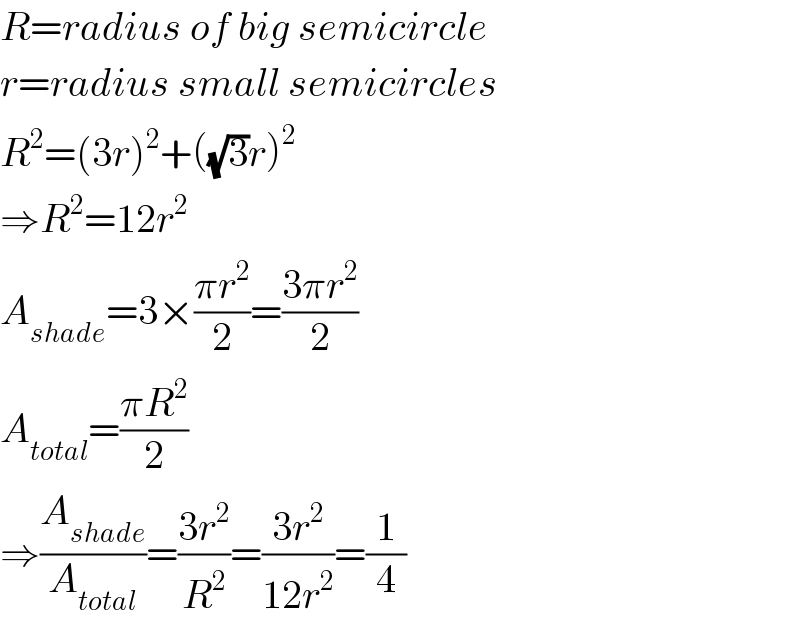
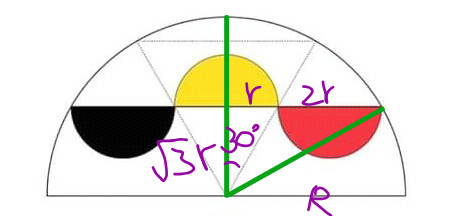
$${R}={radius}\:{of}\:{big}\:{semicircle} \\ $$$${r}={radius}\:{small}\:{semicircles} \\ $$$${R}^{\mathrm{2}} =\left(\mathrm{3}{r}\right)^{\mathrm{2}} +\left(\sqrt{\mathrm{3}}{r}\right)^{\mathrm{2}} \\ $$$$\Rightarrow{R}^{\mathrm{2}} =\mathrm{12}{r}^{\mathrm{2}} \\ $$$${A}_{{shade}} =\mathrm{3}×\frac{\pi{r}^{\mathrm{2}} }{\mathrm{2}}=\frac{\mathrm{3}\pi{r}^{\mathrm{2}} }{\mathrm{2}} \\ $$$${A}_{{total}} =\frac{\pi{R}^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\Rightarrow\frac{{A}_{{shade}} }{{A}_{{total}} }=\frac{\mathrm{3}{r}^{\mathrm{2}} }{{R}^{\mathrm{2}} }=\frac{\mathrm{3}{r}^{\mathrm{2}} }{\mathrm{12}{r}^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{4}} \\ $$
Commented by mr W last updated on 20/Jun/19
Commented by Tawa1 last updated on 20/Jun/19

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
Commented by Tawa1 last updated on 20/Jun/19

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
