
Question Number 62330 by maxmathsup by imad last updated on 19/Jun/19
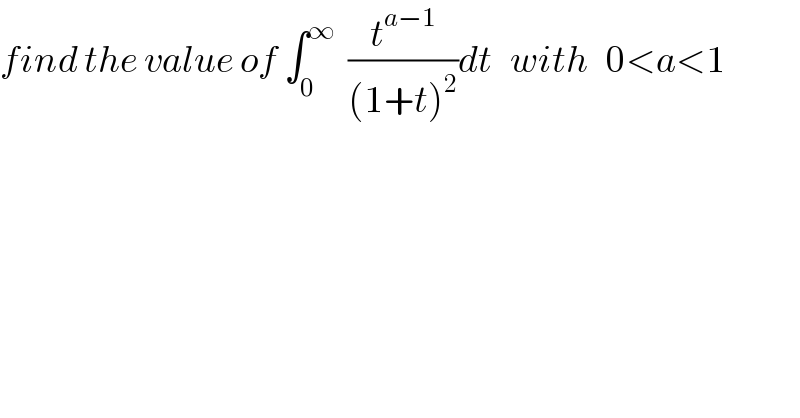
$${find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{t}^{{a}−\mathrm{1}} }{\left(\mathrm{1}+{t}\right)^{\mathrm{2}} }{dt}\:\:\:{with}\:\:\:\mathrm{0}<{a}<\mathrm{1} \\ $$
Commented bymathmax by abdo last updated on 04/Jul/19
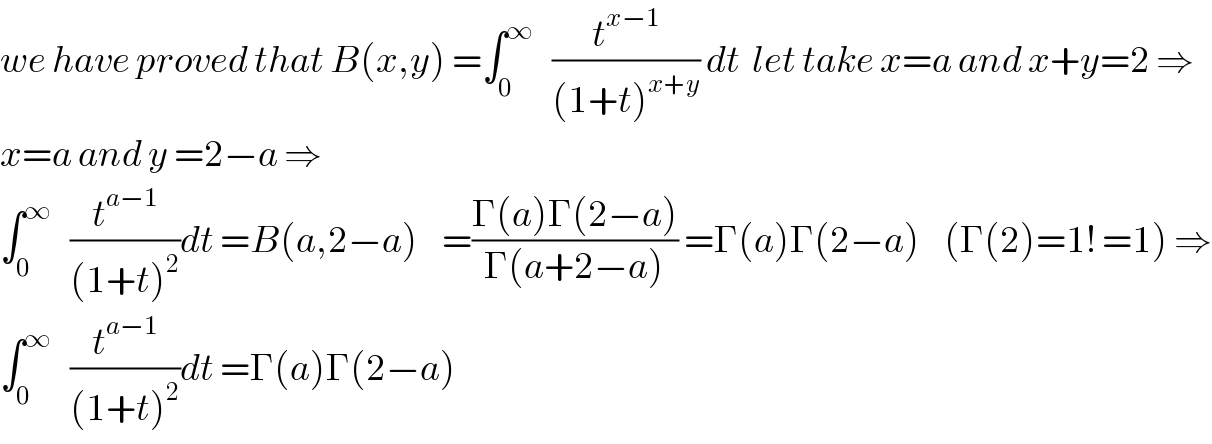
$${we}\:{have}\:{proved}\:{that}\:{B}\left({x},{y}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{t}^{{x}−\mathrm{1}} }{\left(\mathrm{1}+{t}\right)^{{x}+{y}} }\:{dt}\:\:{let}\:{take}\:{x}={a}\:{and}\:{x}+{y}=\mathrm{2}\:\Rightarrow \\ $$ $${x}={a}\:{and}\:{y}\:=\mathrm{2}−{a}\:\Rightarrow \\ $$ $$\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{t}^{{a}−\mathrm{1}} }{\left(\mathrm{1}+{t}\right)^{\mathrm{2}} }{dt}\:={B}\left({a},\mathrm{2}−{a}\right)\:\:\:\:=\frac{\Gamma\left({a}\right)\Gamma\left(\mathrm{2}−{a}\right)}{\Gamma\left({a}+\mathrm{2}−{a}\right)}\:=\Gamma\left({a}\right)\Gamma\left(\mathrm{2}−{a}\right)\:\:\:\:\left(\Gamma\left(\mathrm{2}\right)=\mathrm{1}!\:=\mathrm{1}\right)\:\Rightarrow \\ $$ $$\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{t}^{{a}−\mathrm{1}} }{\left(\mathrm{1}+{t}\right)^{\mathrm{2}} }{dt}\:=\Gamma\left({a}\right)\Gamma\left(\mathrm{2}−{a}\right) \\ $$
Answered by tanmay last updated on 19/Jun/19
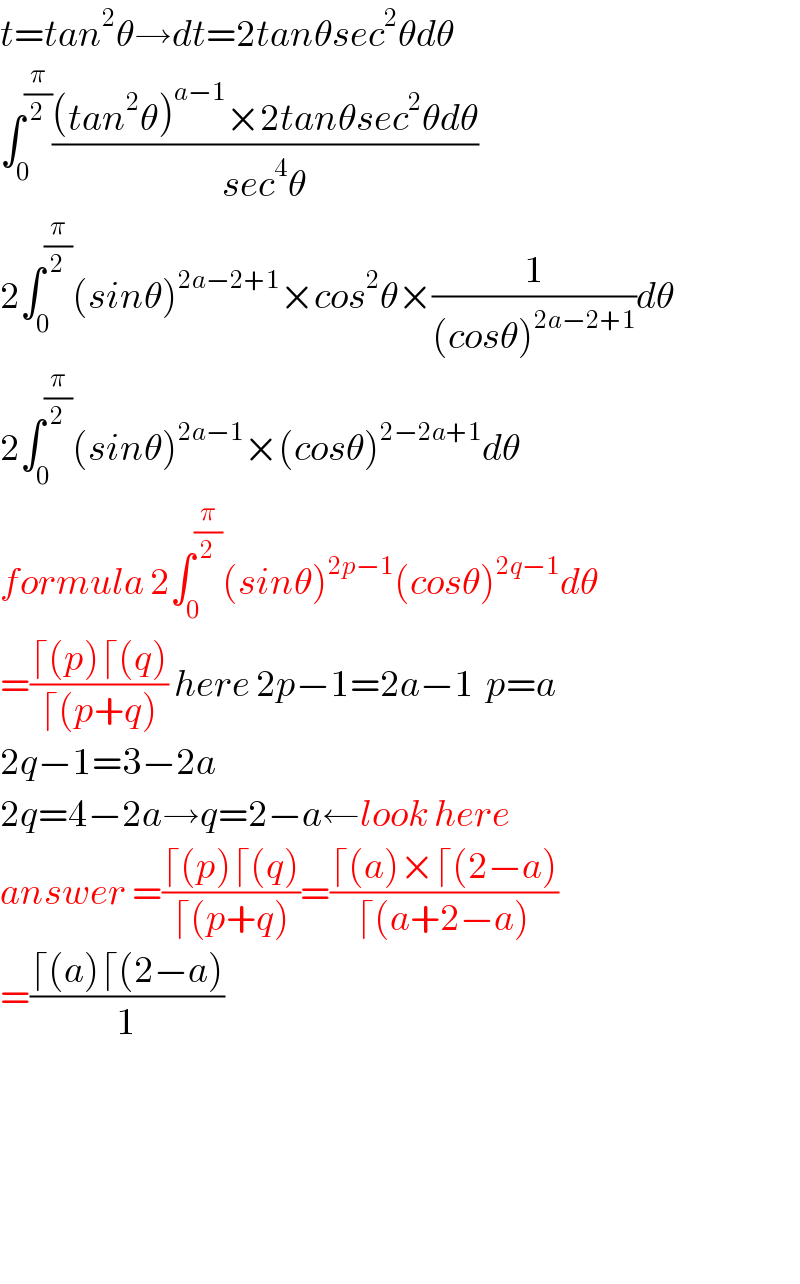
$${t}={tan}^{\mathrm{2}} \theta\rightarrow{dt}=\mathrm{2}{tan}\theta{sec}^{\mathrm{2}} \theta{d}\theta\:\: \\ $$ $$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\left({tan}^{\mathrm{2}} \theta\right)^{{a}−\mathrm{1}} ×\mathrm{2}{tan}\theta{sec}^{\mathrm{2}} \theta{d}\theta}{{sec}^{\mathrm{4}} \theta} \\ $$ $$\mathrm{2}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left({sin}\theta\right)^{\mathrm{2}{a}−\mathrm{2}+\mathrm{1}} ×{cos}^{\mathrm{2}} \theta×\frac{\mathrm{1}}{\left({cos}\theta\right)^{\mathrm{2}{a}−\mathrm{2}+\mathrm{1}} }{d}\theta \\ $$ $$\mathrm{2}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left({sin}\theta\right)^{\mathrm{2}{a}−\mathrm{1}} ×\left({cos}\theta\right)^{\mathrm{2}−\mathrm{2}{a}+\mathrm{1}} {d}\theta \\ $$ $${formula}\:\mathrm{2}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left({sin}\theta\right)^{\mathrm{2}{p}−\mathrm{1}} \left({cos}\theta\right)^{\mathrm{2}{q}−\mathrm{1}} {d}\theta \\ $$ $$=\frac{\lceil\left({p}\right)\lceil\left({q}\right)}{\lceil\left({p}+{q}\right)}\:{here}\:\mathrm{2}{p}−\mathrm{1}=\mathrm{2}{a}−\mathrm{1}\:\:{p}={a} \\ $$ $$\mathrm{2}{q}−\mathrm{1}=\mathrm{3}−\mathrm{2}{a} \\ $$ $$\mathrm{2}{q}=\mathrm{4}−\mathrm{2}{a}\rightarrow{q}=\mathrm{2}−{a}\leftarrow{look}\:{here} \\ $$ $${answer}\:=\frac{\lceil\left({p}\right)\lceil\left({q}\right)}{\lceil\left({p}+{q}\right)}=\frac{\lceil\left({a}\right)×\lceil\left(\mathrm{2}−{a}\right)}{\lceil\left({a}+\mathrm{2}−{a}\right)} \\ $$ $$=\frac{\lceil\left({a}\right)\lceil\left(\mathrm{2}−{a}\right)}{\mathrm{1}} \\ $$ $$ \\ $$ $$ \\ $$ $$ \\ $$ $$ \\ $$
