
Question Number 62093 by naka3546 last updated on 15/Jun/19
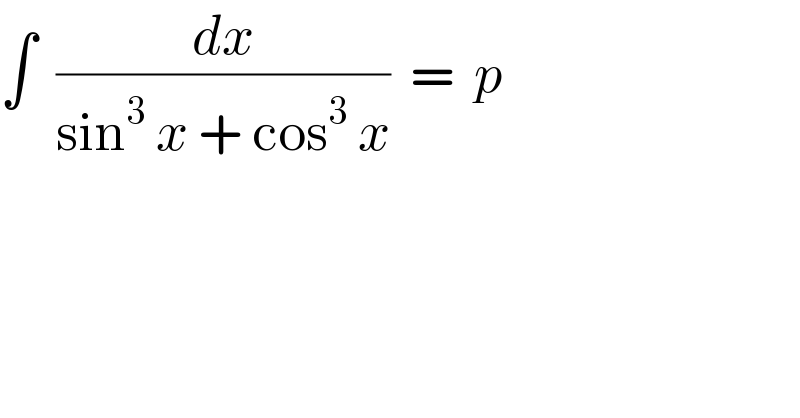
$$\int\:\:\frac{{dx}}{\mathrm{sin}^{\mathrm{3}} \:{x}\:+\:\mathrm{cos}^{\mathrm{3}} \:{x}}\:\:=\:\:{p} \\ $$
Commented by MJS last updated on 15/Jun/19
![Weierstrass Substitution ∫(dx/(sin^3 x +cos^3 x))= [t=tan (x/2) → dx=((2dt)/(1+t^2 )); sin x =((2t)/(1+t^2 )); cos x =((1−t^2 )/(1+t^2 ))] =−2∫(((t^2 +1)^2 )/((t^2 −2t−1)(t^4 +2t^3 +2t^2 −2t+1)))dt= =−2∫(((t^2 +1)^2 )/((t−1−(√2))(t−1+(√2))(t^2 +(1−(√3))t+2−(√3))(t^2 +(1+(√3))t+2+(√3))))dt= =−((√2)/3)∫(dt/(t−1−(√2)))+((√2)/3)∫(dt/(t−1+(√2)))−((1−(√3))/3)∫(dt/(t^2 +(1−(√3))t+2−(√3)))−((1+(√3))/3)∫(dt/(t^2 +(1+(√3))t+2+(√3)))= =−((√2)/3)ln (t−1−(√2)) +((√2)/3)ln (t−1+(√2)) +(2/3)arctan ((1+(√3))t−1) +(2/3)arctan ((1−(√3))t−1) = =((√2)/3)ln ((t−1+(√2))/(t−1−(√2))) +(2/3)(arctan ((1+(√3))t−1) +arctan ((1−(√3))t−1))= =((√2)/3)ln ∣((tan (x/2) −1+(√2))/(tan (x/2)−1−(√2)))∣ −(2/3)(arctan (1−(1+(√3))tan (x/2)) +arctan (1−(1−(√3))tan (x/2)))+C](Q62123.png)
$$\mathrm{Weierstrass}\:\mathrm{Substitution} \\ $$$$\int\frac{{dx}}{\mathrm{sin}^{\mathrm{3}} \:{x}\:+\mathrm{cos}^{\mathrm{3}} \:{x}}= \\ $$$$\:\:\:\:\:\left[{t}=\mathrm{tan}\:\frac{{x}}{\mathrm{2}}\:\rightarrow\:{dx}=\frac{\mathrm{2}{dt}}{\mathrm{1}+{t}^{\mathrm{2}} };\:\mathrm{sin}\:{x}\:=\frac{\mathrm{2}{t}}{\mathrm{1}+{t}^{\mathrm{2}} };\:\mathrm{cos}\:{x}\:=\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }\right] \\ $$$$=−\mathrm{2}\int\frac{\left({t}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} }{\left({t}^{\mathrm{2}} −\mathrm{2}{t}−\mathrm{1}\right)\left({t}^{\mathrm{4}} +\mathrm{2}{t}^{\mathrm{3}} +\mathrm{2}{t}^{\mathrm{2}} −\mathrm{2}{t}+\mathrm{1}\right)}{dt}= \\ $$$$=−\mathrm{2}\int\frac{\left({t}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} }{\left({t}−\mathrm{1}−\sqrt{\mathrm{2}}\right)\left({t}−\mathrm{1}+\sqrt{\mathrm{2}}\right)\left({t}^{\mathrm{2}} +\left(\mathrm{1}−\sqrt{\mathrm{3}}\right){t}+\mathrm{2}−\sqrt{\mathrm{3}}\right)\left({t}^{\mathrm{2}} +\left(\mathrm{1}+\sqrt{\mathrm{3}}\right){t}+\mathrm{2}+\sqrt{\mathrm{3}}\right)}{dt}= \\ $$$$=−\frac{\sqrt{\mathrm{2}}}{\mathrm{3}}\int\frac{{dt}}{{t}−\mathrm{1}−\sqrt{\mathrm{2}}}+\frac{\sqrt{\mathrm{2}}}{\mathrm{3}}\int\frac{{dt}}{{t}−\mathrm{1}+\sqrt{\mathrm{2}}}−\frac{\mathrm{1}−\sqrt{\mathrm{3}}}{\mathrm{3}}\int\frac{{dt}}{{t}^{\mathrm{2}} +\left(\mathrm{1}−\sqrt{\mathrm{3}}\right){t}+\mathrm{2}−\sqrt{\mathrm{3}}}−\frac{\mathrm{1}+\sqrt{\mathrm{3}}}{\mathrm{3}}\int\frac{{dt}}{{t}^{\mathrm{2}} +\left(\mathrm{1}+\sqrt{\mathrm{3}}\right){t}+\mathrm{2}+\sqrt{\mathrm{3}}}= \\ $$$$=−\frac{\sqrt{\mathrm{2}}}{\mathrm{3}}\mathrm{ln}\:\left({t}−\mathrm{1}−\sqrt{\mathrm{2}}\right)\:+\frac{\sqrt{\mathrm{2}}}{\mathrm{3}}\mathrm{ln}\:\left({t}−\mathrm{1}+\sqrt{\mathrm{2}}\right)\:+\frac{\mathrm{2}}{\mathrm{3}}\mathrm{arctan}\:\left(\left(\mathrm{1}+\sqrt{\mathrm{3}}\right){t}−\mathrm{1}\right)\:+\frac{\mathrm{2}}{\mathrm{3}}\mathrm{arctan}\:\left(\left(\mathrm{1}−\sqrt{\mathrm{3}}\right){t}−\mathrm{1}\right)\:= \\ $$$$=\frac{\sqrt{\mathrm{2}}}{\mathrm{3}}\mathrm{ln}\:\frac{{t}−\mathrm{1}+\sqrt{\mathrm{2}}}{{t}−\mathrm{1}−\sqrt{\mathrm{2}}}\:+\frac{\mathrm{2}}{\mathrm{3}}\left(\mathrm{arctan}\:\left(\left(\mathrm{1}+\sqrt{\mathrm{3}}\right){t}−\mathrm{1}\right)\:+\mathrm{arctan}\:\left(\left(\mathrm{1}−\sqrt{\mathrm{3}}\right){t}−\mathrm{1}\right)\right)= \\ $$$$=\frac{\sqrt{\mathrm{2}}}{\mathrm{3}}\mathrm{ln}\:\mid\frac{\mathrm{tan}\:\frac{{x}}{\mathrm{2}}\:−\mathrm{1}+\sqrt{\mathrm{2}}}{\mathrm{tan}\:\frac{{x}}{\mathrm{2}}−\mathrm{1}−\sqrt{\mathrm{2}}}\mid\:−\frac{\mathrm{2}}{\mathrm{3}}\left(\mathrm{arctan}\:\left(\mathrm{1}−\left(\mathrm{1}+\sqrt{\mathrm{3}}\right)\mathrm{tan}\:\frac{{x}}{\mathrm{2}}\right)\:+\mathrm{arctan}\:\left(\mathrm{1}−\left(\mathrm{1}−\sqrt{\mathrm{3}}\right)\mathrm{tan}\:\frac{{x}}{\mathrm{2}}\right)\right)+{C} \\ $$
