
Question Number 59526 by Mr X pcx last updated on 11/May/19
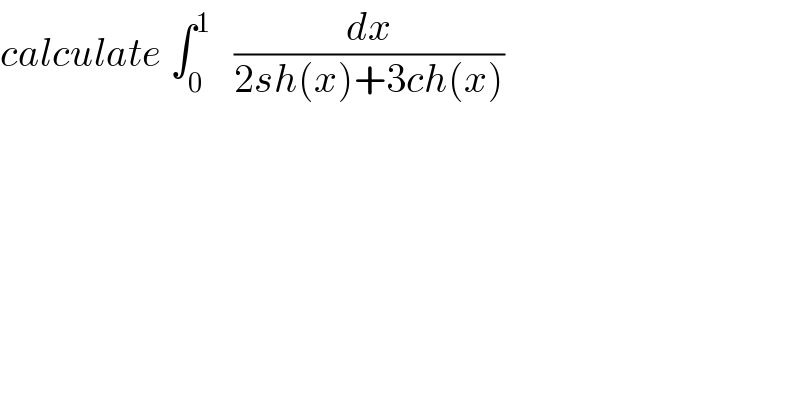
$${calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{dx}}{\mathrm{2}{sh}\left({x}\right)+\mathrm{3}{ch}\left({x}\right)} \\ $$
Commented by maxmathsup by imad last updated on 12/May/19
![let I =∫_0 ^1 (dx/(2sh(x)+3ch(x))) ⇒I =∫_0 ^1 (dx/(2((e^x −e^(−x) )/2) +3((e^x +e^(−x) )/2))) =∫_0 ^1 ((2dx)/(2e^x −2e^(−x) +3e^x +3e^(−x) )) =2∫_0 ^1 (dx/(5e^x +e^(−x) )) =_(e^x =t) 2 ∫_1 ^e (dt/(t(5t +(1/t)))) =2 ∫_1 ^e (dt/(5t^2 +1)) =_((√5)t =u) 2 ∫_(√5) ^(e(√5)) (du/((√5)(u^2 +1))) =(2/(√5)) [arctan(u)]_(√5) ^(e(√5)) =(2/(√5)){arctan(e(√5))−arctan((√5))}](Q59579.png)
$${let}\:{I}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{dx}}{\mathrm{2}{sh}\left({x}\right)+\mathrm{3}{ch}\left({x}\right)}\:\Rightarrow{I}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{dx}}{\mathrm{2}\frac{{e}^{{x}} −{e}^{−{x}} }{\mathrm{2}}\:+\mathrm{3}\frac{{e}^{{x}} \:+{e}^{−{x}} }{\mathrm{2}}} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{2}{dx}}{\mathrm{2}{e}^{{x}} −\mathrm{2}{e}^{−{x}} \:+\mathrm{3}{e}^{{x}} \:+\mathrm{3}{e}^{−{x}} }\:=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{dx}}{\mathrm{5}{e}^{{x}} \:+{e}^{−{x}} }\:=_{{e}^{{x}} ={t}} \mathrm{2}\:\:\:\:\int_{\mathrm{1}} ^{{e}} \:\:\:\:\frac{{dt}}{{t}\left(\mathrm{5}{t}\:+\frac{\mathrm{1}}{{t}}\right)} \\ $$$$=\mathrm{2}\:\int_{\mathrm{1}} ^{{e}} \:\:\:\:\frac{{dt}}{\mathrm{5}{t}^{\mathrm{2}} \:+\mathrm{1}}\:=_{\sqrt{\mathrm{5}}{t}\:={u}} \:\:\:\mathrm{2}\:\:\int_{\sqrt{\mathrm{5}}} ^{{e}\sqrt{\mathrm{5}}} \:\:\:\:\:\frac{{du}}{\sqrt{\mathrm{5}}\left({u}^{\mathrm{2}} \:+\mathrm{1}\right)}\:=\frac{\mathrm{2}}{\sqrt{\mathrm{5}}}\:\left[{arctan}\left({u}\right)\right]_{\sqrt{\mathrm{5}}} ^{{e}\sqrt{\mathrm{5}}} \\ $$$$=\frac{\mathrm{2}}{\sqrt{\mathrm{5}}}\left\{{arctan}\left({e}\sqrt{\mathrm{5}}\right)−{arctan}\left(\sqrt{\mathrm{5}}\right)\right\} \\ $$
Answered by MJS last updated on 11/May/19
![∫(dx/(2sinh x +3cosh x))=2∫(e^x /(5e^(2x) +1))dx= [t=(√5)e^x → dx=(dt/t)] =((2(√5))/5)∫(dt/(t^2 +1))=((2(√5))/5)arctan t =((2(√5))/5)arctan (√5)e^x +C ∫_0 ^1 (dx/(2sinh x +3cosh x))=((2(√5))/5)(arctan (√5)e −arctan (√5))≈.230292](Q59529.png)
$$\int\frac{{dx}}{\mathrm{2sinh}\:{x}\:+\mathrm{3cosh}\:{x}}=\mathrm{2}\int\frac{\mathrm{e}^{{x}} }{\mathrm{5e}^{\mathrm{2}{x}} +\mathrm{1}}{dx}= \\ $$$$\:\:\:\:\:\left[{t}=\sqrt{\mathrm{5}}\mathrm{e}^{{x}} \:\rightarrow\:{dx}=\frac{{dt}}{{t}}\right] \\ $$$$=\frac{\mathrm{2}\sqrt{\mathrm{5}}}{\mathrm{5}}\int\frac{{dt}}{{t}^{\mathrm{2}} +\mathrm{1}}=\frac{\mathrm{2}\sqrt{\mathrm{5}}}{\mathrm{5}}\mathrm{arctan}\:{t}\:=\frac{\mathrm{2}\sqrt{\mathrm{5}}}{\mathrm{5}}\mathrm{arctan}\:\sqrt{\mathrm{5}}\mathrm{e}^{{x}} \:+{C} \\ $$$$\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\frac{{dx}}{\mathrm{2sinh}\:{x}\:+\mathrm{3cosh}\:{x}}=\frac{\mathrm{2}\sqrt{\mathrm{5}}}{\mathrm{5}}\left(\mathrm{arctan}\:\sqrt{\mathrm{5}}\mathrm{e}\:−\mathrm{arctan}\:\sqrt{\mathrm{5}}\right)\approx.\mathrm{230292} \\ $$
Commented by maxmathsup by imad last updated on 13/May/19

$${thanks}\:{sir}\:{mjs}\:. \\ $$
