
Question Number 61855 by aliesam last updated on 10/Jun/19
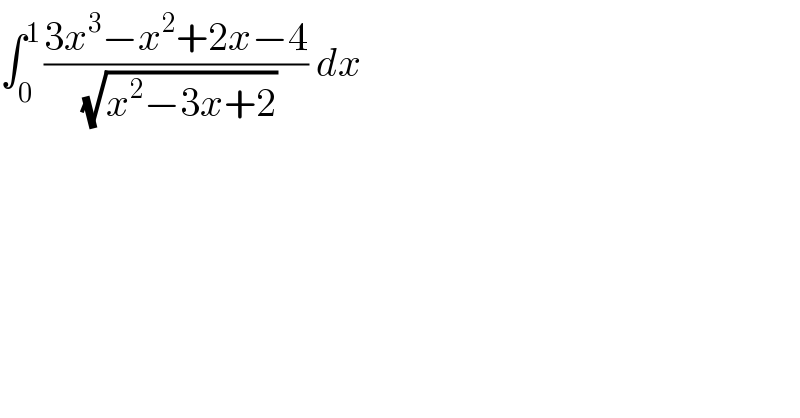
$$\int_{\mathrm{0}\:} ^{\mathrm{1}} \frac{\mathrm{3}{x}^{\mathrm{3}} −{x}^{\mathrm{2}} +\mathrm{2}{x}−\mathrm{4}}{\sqrt{{x}^{\mathrm{2}} −\mathrm{3}{x}+\mathrm{2}}}\:{dx} \\ $$
Answered by MJS last updated on 10/Jun/19
![((3x^3 −x^2 +2x−4)/(√(x^2 −3x+2)))=−(3x^2 +2x+4)(√((x−1)/(x−2))) for x<2 −∫(3x^2 +2x+4)(√((x−1)/(x−2)))dx= [t=(√((x−1)/(x−2))) → dx=−2(√((x−2)^3 (x−1)))] =∫((2t^2 (20t^4 −26t^2 +9))/((t^2 −1)^4 ))dt= =∫((3/(8(t−1)^4 ))+(7/(2(t−1)^3 ))+((185)/(16(t−1)^2 ))+((135)/(16(t−1)))+(3/(8(t+1)^4 ))−(7/(2(t+1)^3 ))+((185)/(16(t+1)^2 ))−((135)/(16(t+1))))dt= =−(1/(8(t−1)^3 ))−(7/(4(t−1)^2 ))−((185)/(16(t−1)))+((135)/(16))ln (t−1)−(1/(8(t+1)^3 ))+(7/(4(t+1)^2 ))−((185)/(16(t+1)))−((135)/(16))ln (t+1) = =−((t(185t^4 −312t^2 +135))/(8(t^2 −1)^3 ))+((135)/(16))ln ((t−1)/(t+1)) = =−(1/8)(8x^2 +26x+101)(√(x^2 −3x+2))+((135)/(16))ln (2x−3−2(√(x^2 −3x+2))) +C ∫_(0 ) ^1 ((3x^3 −x^2 +2x−4)/(√(x^2 −3x+2))) dx=−((101(√2))/8)−((135)/(16))ln (3−2(√2))](Q61893.png)
$$\frac{\mathrm{3}{x}^{\mathrm{3}} −{x}^{\mathrm{2}} +\mathrm{2}{x}−\mathrm{4}}{\sqrt{{x}^{\mathrm{2}} −\mathrm{3}{x}+\mathrm{2}}}=−\left(\mathrm{3}{x}^{\mathrm{2}} +\mathrm{2}{x}+\mathrm{4}\right)\sqrt{\frac{{x}−\mathrm{1}}{{x}−\mathrm{2}}}\:\mathrm{for}\:{x}<\mathrm{2} \\ $$$$−\int\left(\mathrm{3}{x}^{\mathrm{2}} +\mathrm{2}{x}+\mathrm{4}\right)\sqrt{\frac{{x}−\mathrm{1}}{{x}−\mathrm{2}}}{dx}= \\ $$$$\:\:\:\:\:\left[{t}=\sqrt{\frac{{x}−\mathrm{1}}{{x}−\mathrm{2}}}\:\rightarrow\:{dx}=−\mathrm{2}\sqrt{\left({x}−\mathrm{2}\right)^{\mathrm{3}} \left({x}−\mathrm{1}\right)}\right] \\ $$$$=\int\frac{\mathrm{2}{t}^{\mathrm{2}} \left(\mathrm{20}{t}^{\mathrm{4}} −\mathrm{26}{t}^{\mathrm{2}} +\mathrm{9}\right)}{\left({t}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{4}} }{dt}= \\ $$$$=\int\left(\frac{\mathrm{3}}{\mathrm{8}\left({t}−\mathrm{1}\right)^{\mathrm{4}} }+\frac{\mathrm{7}}{\mathrm{2}\left({t}−\mathrm{1}\right)^{\mathrm{3}} }+\frac{\mathrm{185}}{\mathrm{16}\left({t}−\mathrm{1}\right)^{\mathrm{2}} }+\frac{\mathrm{135}}{\mathrm{16}\left({t}−\mathrm{1}\right)}+\frac{\mathrm{3}}{\mathrm{8}\left({t}+\mathrm{1}\right)^{\mathrm{4}} }−\frac{\mathrm{7}}{\mathrm{2}\left({t}+\mathrm{1}\right)^{\mathrm{3}} }+\frac{\mathrm{185}}{\mathrm{16}\left({t}+\mathrm{1}\right)^{\mathrm{2}} }−\frac{\mathrm{135}}{\mathrm{16}\left({t}+\mathrm{1}\right)}\right){dt}= \\ $$$$=−\frac{\mathrm{1}}{\mathrm{8}\left({t}−\mathrm{1}\right)^{\mathrm{3}} }−\frac{\mathrm{7}}{\mathrm{4}\left({t}−\mathrm{1}\right)^{\mathrm{2}} }−\frac{\mathrm{185}}{\mathrm{16}\left({t}−\mathrm{1}\right)}+\frac{\mathrm{135}}{\mathrm{16}}\mathrm{ln}\:\left({t}−\mathrm{1}\right)−\frac{\mathrm{1}}{\mathrm{8}\left({t}+\mathrm{1}\right)^{\mathrm{3}} }+\frac{\mathrm{7}}{\mathrm{4}\left({t}+\mathrm{1}\right)^{\mathrm{2}} }−\frac{\mathrm{185}}{\mathrm{16}\left({t}+\mathrm{1}\right)}−\frac{\mathrm{135}}{\mathrm{16}}\mathrm{ln}\:\left({t}+\mathrm{1}\right)\:= \\ $$$$=−\frac{{t}\left(\mathrm{185}{t}^{\mathrm{4}} −\mathrm{312}{t}^{\mathrm{2}} +\mathrm{135}\right)}{\mathrm{8}\left({t}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{3}} }+\frac{\mathrm{135}}{\mathrm{16}}\mathrm{ln}\:\frac{{t}−\mathrm{1}}{{t}+\mathrm{1}}\:= \\ $$$$=−\frac{\mathrm{1}}{\mathrm{8}}\left(\mathrm{8}{x}^{\mathrm{2}} +\mathrm{26}{x}+\mathrm{101}\right)\sqrt{{x}^{\mathrm{2}} −\mathrm{3}{x}+\mathrm{2}}+\frac{\mathrm{135}}{\mathrm{16}}\mathrm{ln}\:\left(\mathrm{2}{x}−\mathrm{3}−\mathrm{2}\sqrt{{x}^{\mathrm{2}} −\mathrm{3}{x}+\mathrm{2}}\right)\:+{C} \\ $$$$\int_{\mathrm{0}\:} ^{\mathrm{1}} \frac{\mathrm{3}{x}^{\mathrm{3}} −{x}^{\mathrm{2}} +\mathrm{2}{x}−\mathrm{4}}{\sqrt{{x}^{\mathrm{2}} −\mathrm{3}{x}+\mathrm{2}}}\:{dx}=−\frac{\mathrm{101}\sqrt{\mathrm{2}}}{\mathrm{8}}−\frac{\mathrm{135}}{\mathrm{16}}\mathrm{ln}\:\left(\mathrm{3}−\mathrm{2}\sqrt{\mathrm{2}}\right) \\ $$
Commented by aliesam last updated on 10/Jun/19
$${thanks}\:{sir}\:{brilliant}\:{solution} \\ $$
Commented by MJS last updated on 10/Jun/19

$$\mathrm{you}'\mathrm{re}\:\mathrm{welcome} \\ $$
