
Previous in Relation and Functions Next in Relation and Functions
Question Number 56962 by turbo msup by abdo last updated on 27/Mar/19
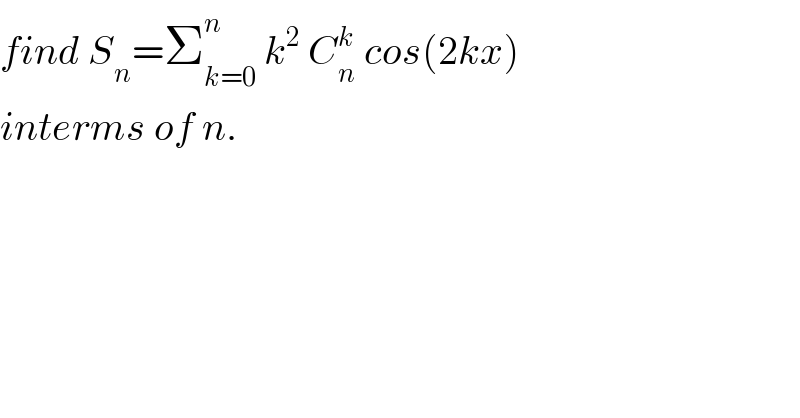
$${find}\:{S}_{{n}} =\sum_{{k}=\mathrm{0}} ^{{n}} \:{k}^{\mathrm{2}} \:{C}_{{n}} ^{{k}} \:{cos}\left(\mathrm{2}{kx}\right) \\ $$$${interms}\:{of}\:{n}. \\ $$
Commented by maxmathsup by imad last updated on 29/Mar/19
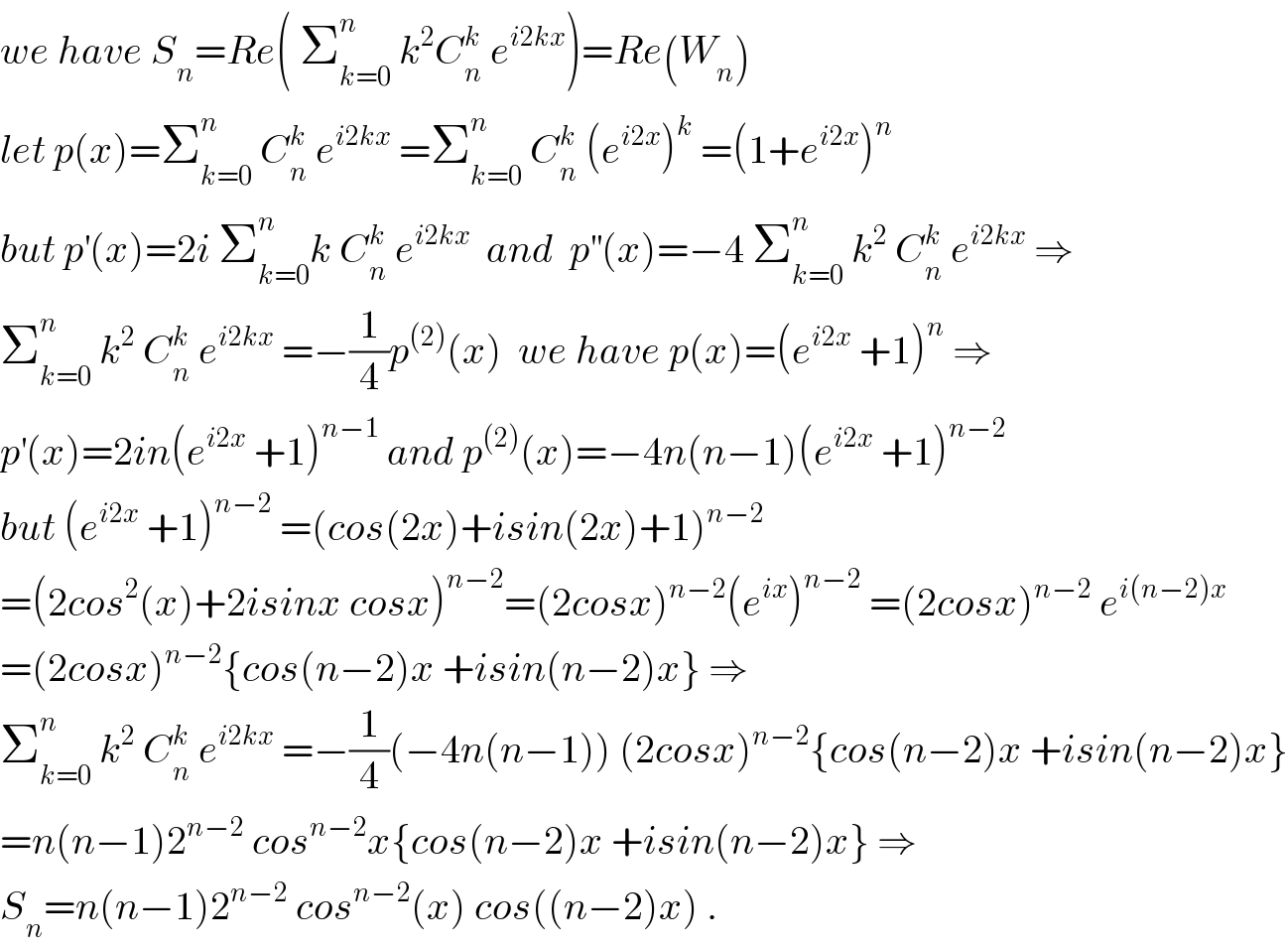
$${we}\:{have}\:{S}_{{n}} ={Re}\left(\:\sum_{{k}=\mathrm{0}} ^{{n}} \:{k}^{\mathrm{2}} {C}_{{n}} ^{{k}} \:{e}^{{i}\mathrm{2}{kx}} \right)={Re}\left({W}_{{n}} \right) \\ $$$${let}\:{p}\left({x}\right)=\sum_{{k}=\mathrm{0}} ^{{n}} \:{C}_{{n}} ^{{k}} \:{e}^{{i}\mathrm{2}{kx}} \:=\sum_{{k}=\mathrm{0}} ^{{n}} \:{C}_{{n}} ^{{k}} \:\left({e}^{{i}\mathrm{2}{x}} \right)^{{k}} \:=\left(\mathrm{1}+{e}^{{i}\mathrm{2}{x}} \right)^{{n}} \: \\ $$$${but}\:{p}^{'} \left({x}\right)=\mathrm{2}{i}\:\sum_{{k}=\mathrm{0}} ^{{n}} {k}\:{C}_{{n}} ^{{k}} \:{e}^{{i}\mathrm{2}{kx}} \:\:{and}\:\:{p}^{''} \left({x}\right)=−\mathrm{4}\:\sum_{{k}=\mathrm{0}} ^{{n}} \:{k}^{\mathrm{2}} \:{C}_{{n}} ^{{k}} \:{e}^{{i}\mathrm{2}{kx}} \:\Rightarrow \\ $$$$\sum_{{k}=\mathrm{0}} ^{{n}} \:{k}^{\mathrm{2}} \:{C}_{{n}} ^{{k}} \:{e}^{{i}\mathrm{2}{kx}} \:=−\frac{\mathrm{1}}{\mathrm{4}}{p}^{\left(\mathrm{2}\right)} \left({x}\right)\:\:{we}\:{have}\:{p}\left({x}\right)=\left({e}^{{i}\mathrm{2}{x}} \:+\mathrm{1}\right)^{{n}} \:\Rightarrow \\ $$$${p}^{'} \left({x}\right)=\mathrm{2}{in}\left({e}^{{i}\mathrm{2}{x}} \:+\mathrm{1}\right)^{{n}−\mathrm{1}} \:{and}\:{p}^{\left(\mathrm{2}\right)} \left({x}\right)=−\mathrm{4}{n}\left({n}−\mathrm{1}\right)\left({e}^{{i}\mathrm{2}{x}} \:+\mathrm{1}\right)^{{n}−\mathrm{2}} \\ $$$${but}\:\left({e}^{{i}\mathrm{2}{x}} \:+\mathrm{1}\right)^{{n}−\mathrm{2}} \:=\left({cos}\left(\mathrm{2}{x}\right)+{isin}\left(\mathrm{2}{x}\right)+\mathrm{1}\right)^{{n}−\mathrm{2}} \\ $$$$=\left(\mathrm{2}{cos}^{\mathrm{2}} \left({x}\right)+\mathrm{2}{isinx}\:{cosx}\right)^{{n}−\mathrm{2}} =\left(\mathrm{2}{cosx}\right)^{{n}−\mathrm{2}} \left({e}^{{ix}} \right)^{{n}−\mathrm{2}} \:=\left(\mathrm{2}{cosx}\right)^{{n}−\mathrm{2}} \:{e}^{{i}\left({n}−\mathrm{2}\right){x}} \: \\ $$$$=\left(\mathrm{2}{cosx}\right)^{{n}−\mathrm{2}} \left\{{cos}\left({n}−\mathrm{2}\right){x}\:+{isin}\left({n}−\mathrm{2}\right){x}\right\}\:\Rightarrow \\ $$$$\sum_{{k}=\mathrm{0}} ^{{n}} \:{k}^{\mathrm{2}} \:{C}_{{n}} ^{{k}} \:{e}^{{i}\mathrm{2}{kx}} \:=−\frac{\mathrm{1}}{\mathrm{4}}\left(−\mathrm{4}{n}\left({n}−\mathrm{1}\right)\right)\:\left(\mathrm{2}{cosx}\right)^{{n}−\mathrm{2}} \left\{{cos}\left({n}−\mathrm{2}\right){x}\:+{isin}\left({n}−\mathrm{2}\right){x}\right\} \\ $$$$={n}\left({n}−\mathrm{1}\right)\mathrm{2}^{{n}−\mathrm{2}} \:{cos}^{{n}−\mathrm{2}} {x}\left\{{cos}\left({n}−\mathrm{2}\right){x}\:+{isin}\left({n}−\mathrm{2}\right){x}\right\}\:\Rightarrow \\ $$$${S}_{{n}} ={n}\left({n}−\mathrm{1}\right)\mathrm{2}^{{n}−\mathrm{2}} \:{cos}^{{n}−\mathrm{2}} \left({x}\right)\:{cos}\left(\left({n}−\mathrm{2}\right){x}\right)\:. \\ $$
Answered by Smail last updated on 27/Mar/19

$${S}_{{n}} \left({x}\right)=\underset{{k}=\mathrm{0}} {\overset{{n}} {\sum}}{k}^{\mathrm{2}} {C}_{{n}} ^{{k}} {cos}\left(\mathrm{2}{kx}\right)={Re}\left(\underset{{k}=\mathrm{0}} {\overset{{n}} {\sum}}{k}^{\mathrm{2}} \:^{{n}} {C}_{{k}} {e}^{\mathrm{2}{ikx}} \right) \\ $$$${z}''\left({x}\right)=\underset{{k}=\mathrm{0}} {\overset{{n}} {\sum}}{k}^{\mathrm{2}} \:^{{n}} {C}_{{k}} {e}^{\mathrm{2}{ikx}} \\ $$$${z}'\left({x}\right)=\frac{\mathrm{1}}{\mathrm{2}{i}}\underset{{k}=\mathrm{0}} {\overset{{n}} {\sum}}{k}\:^{{n}} {C}_{{k}} {e}^{\mathrm{2}{ikx}} +{c} \\ $$$${z}\left({x}\right)=−\frac{\mathrm{1}}{\mathrm{4}}\underset{{k}=\mathrm{0}} {\overset{{n}} {\sum}}\:^{{n}} {C}_{{k}} {e}^{\mathrm{2}{ikx}} +{cx}+{a} \\ $$$$=\frac{−\mathrm{1}}{\mathrm{4}}\left(\mathrm{1}+{e}^{\mathrm{2}{ix}} \right)^{{n}} +{cx}+{a} \\ $$$${z}'\left({x}\right)=\frac{\mathrm{1}}{\mathrm{2}{i}}×{ne}^{\mathrm{2}{ix}} \left(\mathrm{1}+{e}^{\mathrm{2}{ix}} \right)^{{n}−\mathrm{1}} +{c} \\ $$$${z}''\left({x}\right)={n}\left({e}^{\mathrm{2}{ix}} \left(\mathrm{1}+{e}^{\mathrm{2}{ix}} \right)^{{n}−\mathrm{1}} +\left({n}−\mathrm{1}\right){e}^{\mathrm{4}{ix}} \left(\mathrm{1}+{e}^{\mathrm{2}{ix}} \right)^{{n}−\mathrm{2}} \right) \\ $$$$={ne}^{\mathrm{2}{ix}} \left(\mathrm{1}+{e}^{\mathrm{2}{ix}} \right)^{{n}−\mathrm{2}} \left(\mathrm{1}+{e}^{\mathrm{2}{ix}} +\left({n}−\mathrm{1}\right){e}^{\mathrm{2}{ix}} \right) \\ $$$$={ne}^{\mathrm{2}{ix}} \left(\mathrm{1}+{e}^{\mathrm{2}{ix}} \right)^{{n}−\mathrm{2}} \left(\mathrm{1}+{ne}^{\mathrm{2}{ix}} \right) \\ $$$$={ne}^{\mathrm{2}{ix}} \left(\mathrm{1}+{cos}\left(\mathrm{2}{x}\right)+{isin}\left(\mathrm{2}{x}\right)\right)^{{n}−\mathrm{2}} \left(\mathrm{1}+{ne}^{\mathrm{2}{ix}} \right) \\ $$$$={ne}^{\mathrm{2}{ix}} \left(\mathrm{2}{cos}^{\mathrm{2}} {x}+\mathrm{2}{isinxcosx}\right)^{{n}−\mathrm{2}} \left(\mathrm{1}+{ne}^{\mathrm{2}{ix}} \right) \\ $$$$={ne}^{\mathrm{2}{ix}} \mathrm{2}^{{n}−\mathrm{2}} {cos}^{{n}−\mathrm{2}} \left({x}\right)\left({e}^{{ix}} \right)^{{n}−\mathrm{2}} \left(\mathrm{1}+{ne}^{\mathrm{2}{ix}} \right) \\ $$$$=\mathrm{2}^{{n}−\mathrm{2}} {ncos}^{{n}−\mathrm{2}} \left({x}\right){e}^{{inx}} \left(\mathrm{1}+{ne}^{\mathrm{2}{ix}} \right) \\ $$$$=\mathrm{2}^{{n}−\mathrm{2}} {ncos}^{{n}−\mathrm{2}} {x}\left({e}^{{inx}} +{ne}^{{i}\left({n}+\mathrm{2}\right){x}} \right) \\ $$$$=\mathrm{2}^{{n}−\mathrm{2}} {ncos}^{{n}−\mathrm{2}} {x}\left({cosnx}+{isin}\left({nx}\right)+{ncos}\left(\left({n}+\mathrm{2}\right){x}\right)+{isin}\left(\left({n}+\mathrm{2}\right){x}\right)\right) \\ $$$${S}_{{n}} =\mathrm{2}^{{n}−\mathrm{2}} {ncos}^{{n}−\mathrm{2}} {x}\left({cosnx}+{ncos}\left({n}+\mathrm{2}\right){x}\right) \\ $$
