
Question Number 53550 by vajpaithegrate@gmail.com last updated on 23/Jan/19

Commented by Kunal12588 last updated on 23/Jan/19
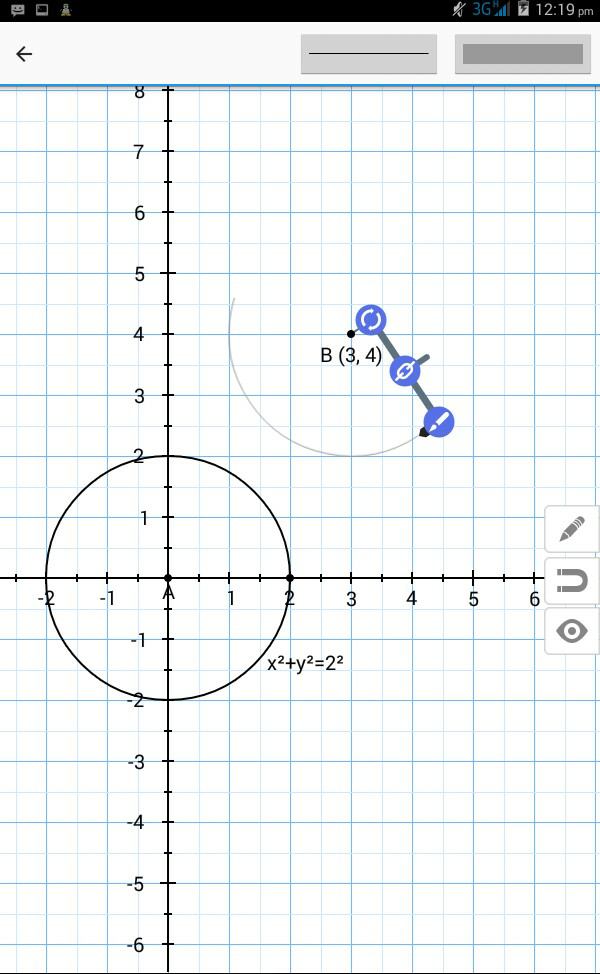
Commented by Kunal12588 last updated on 23/Jan/19
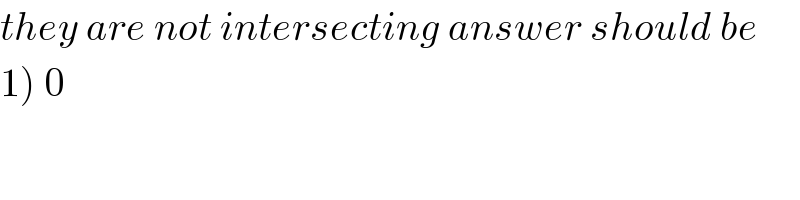
$${they}\:{are}\:{not}\:{intersecting}\:{answer}\:{should}\:{be} \\ $$$$\left.\mathrm{1}\right)\:\mathrm{0} \\ $$
Answered by kaivan.ahmadi last updated on 23/Jan/19
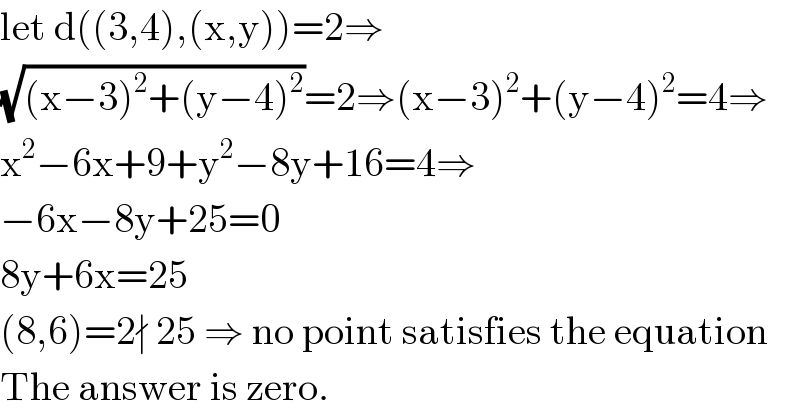
$$\mathrm{let}\:\mathrm{d}\left(\left(\mathrm{3},\mathrm{4}\right),\left(\mathrm{x},\mathrm{y}\right)\right)=\mathrm{2}\Rightarrow \\ $$$$\sqrt{\left(\mathrm{x}−\mathrm{3}\right)^{\mathrm{2}} +\left(\mathrm{y}−\mathrm{4}\right)^{\mathrm{2}} }=\mathrm{2}\Rightarrow\left(\mathrm{x}−\mathrm{3}\right)^{\mathrm{2}} +\left(\mathrm{y}−\mathrm{4}\right)^{\mathrm{2}} =\mathrm{4}\Rightarrow \\ $$$$\mathrm{x}^{\mathrm{2}} −\mathrm{6x}+\mathrm{9}+\mathrm{y}^{\mathrm{2}} −\mathrm{8y}+\mathrm{16}=\mathrm{4}\Rightarrow \\ $$$$−\mathrm{6x}−\mathrm{8y}+\mathrm{25}=\mathrm{0} \\ $$$$\mathrm{8y}+\mathrm{6x}=\mathrm{25} \\ $$$$\left(\mathrm{8},\mathrm{6}\right)=\mathrm{2}\nmid\:\mathrm{25}\:\Rightarrow\:\mathrm{no}\:\mathrm{point}\:\mathrm{satisfies}\:\mathrm{the}\:\mathrm{equation} \\ $$$$\mathrm{The}\:\mathrm{answer}\:\mathrm{is}\:\mathrm{zero}. \\ $$
Commented by vajpaithegrate@gmail.com last updated on 24/Jan/19

$$\mathrm{tnq}\:\mathrm{sir} \\ $$
